"Entangled photons never show interference in the total pattern without coincidence count" implies FTL [closed]

Question

In my previous question, the most defended objection to the gedankenexperiment was that "Entangled photons never show interference in the total pattern without coincidence count".

Here I show another gedankenexperiment where if this affirmation is true then FTL communication is possible.

Assumptions:

• Alice and Bob have a 100% bright entangled photon source
• Alice and Bob's photons are 100% correlated in their polarizations
• Alice and Bob are in spatially disconnected and separated regions
• Alice and Bob have perfectly synchronized clocks
• Alice and Bob know exactly how far away they are from each other

The experiment is:

1. Alice generates a lot of entangled photons (10k for example), sends 1 photon of each pair to Bob and keeps the other photon to herself.
2. Alice keeps her photons undisturbed in some way, could be a mirror in the middle of the path towards Bob. In the diagram I represented it as a "looping device"...
3. Bob's photons take their time to reach Bob's apparatus
4. When the photons reach Bob's apparatus, it first passes through a double slit.
5. After that there is some kind of interference detector (SPAD array? Kolenderski, Piotr, et al. "Time-resolved double-slit interference pattern measurement with entangled photons." Scientific reports 4 (2014).). In this case could be a screen Bob will be watching.

Legend

Experimental setup for FTL if entagled photons never show interference pattern:

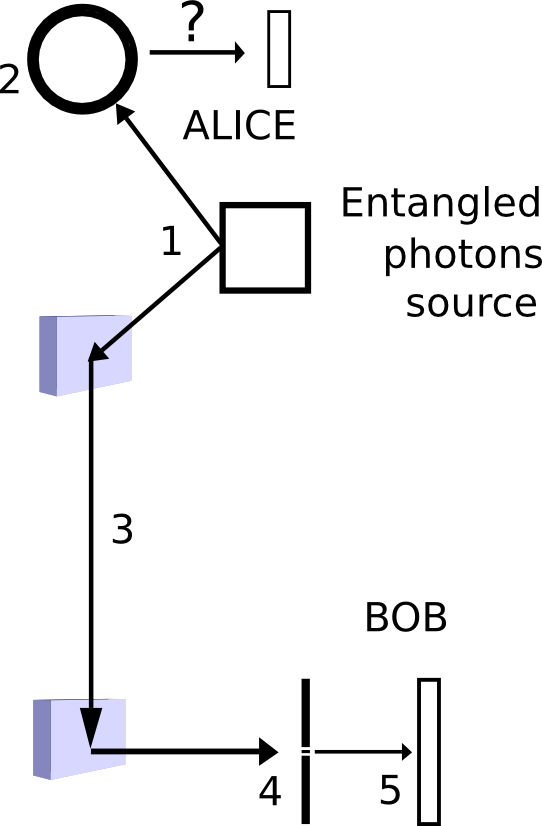
If the affirmation "Entangled photons never show interference in the total pattern without coincidence count" is correct, no interference will be seen by Bob.

Now, if Alice between the steps 3 and 4 (the ideal time would be as the photons get very close to 4, right before the double slit as expected from their synchronized clocks and known distance), measures the polarisation of her photons, the entanglement is broken before Bob's photons arrive at the double slit at step 4.

Thus the photon will have a known polarisation, but that does not interfere with it presenting the typical interference pattern as a non-entagled polarised photon should. Alice is able to choose whether Bob will see the interference pattern or not, nonlocally.

Now to clear up my last question's gedankenexperiment, I also made a drawing for it. The key idea, that circumvents the No-communication theorem, is that once Alice acquires information about the photon's polarisation, she also acquires which-path information. By Wootters, William K., and Wojciech H. Zurek. "Complementarity in the double-slit experiment: Quantum nonseparability and a quantitative statement of Bohr's principle." Physical Review D 19.2 (1979): 473., the interference pattern should not show up, even after Bob's attempt to erase which-path information from the system.

This doesn't contradict the No-communication theorem, but allows FTL communication.

It has the same assumptions as the previous gedankenexperiment.

The experiment is:

1. Alice generates a lot of entangled photons (10k for example), sends 1 photon of each pair to Bob and keeps the other photon to herself.
2. Alice keeps her photons undisturbed in some way, could be a mirror in the middle of the path towards Bob. In the diagram I represented it as a "looping device"...
3. Bob's photons take their time to reach Bob's apparatus
4. When the light reaches Bob's apparatus, it first passes through a double slit.
5. After the double slit, comes the which-path markers, in this case horizontal and vertical polarisers for each slit.
6. After the which-path markers, comes the eraser, in this case a 45o polariser.
7. After the eraser, there is some kind of interference detector (SPAD array? Kolenderski, Piotr, et al. "Time-resolved double-slit interference pattern measurement with entangled photons." Scientific reports 4 (2014).). In this case could be a screen Bob will be watching.

Experimental setup for FTL if complementarity holds:

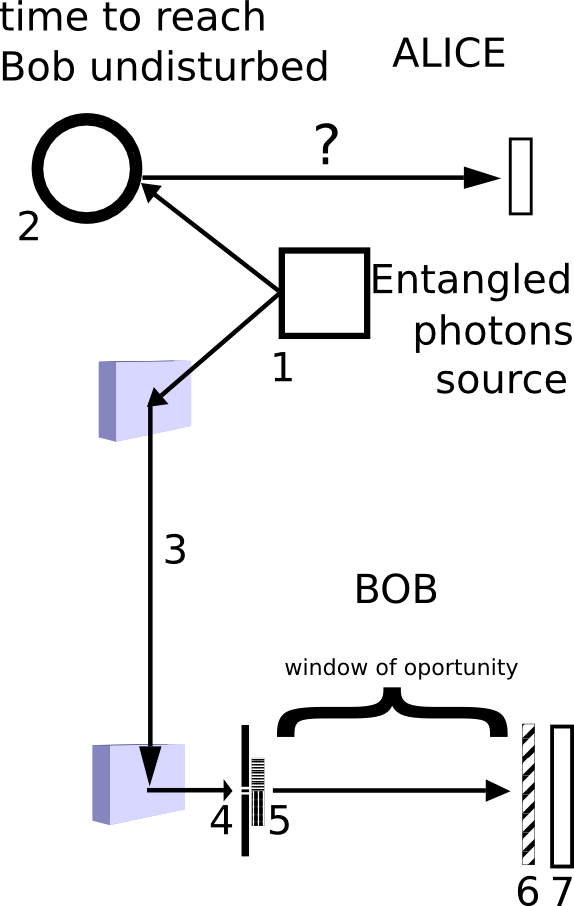
Now, if Alice between the steps 5 and 6 measures the polarisation of her photons, she knows which path Bob's photon took (Of the photons Alice measured, 3/4 of photons were twins of photons absorbed along the way and only 1/4 twins of those that hit the interference detector, but that is irrelevant, the point is of all those that hit the screen, Alice knows the which path information). By complementarity, there should not be an interference pattern.

Alice can choose whether to measure the polarisation of her photons in that specific time the experiment is in between steps 5 and 6, controlling whether the interference pattern will show up for Bob or not, nonlocally.

PS.:

• The 10k number of photons is irrelevant, Alice could send them 1 by 1 and Bob would sum all the detected positions to see if an interference pattern was appearing or not.
• The 100% bright source is not necessary, error-correction will be needed on a real protocol anyway, but there seems to be discoveries of sources with around 18% brightness which should already be enough to test it. The SAGNAC's sources used by Zeilinger's group have very low brightness and cannot be used for this experiment.

Answer 1

Here is the erroneous step:

"Now, if Alice between the steps 3 and 4 (the ideal time would be as the photons get very close to 4, right before the double slit as expected from their synchronized clocks and known distance),measures the polarisation of her photons, the entanglement is broken before Bob's photons arrive at the double slit at step 4. Thus the photon will have a known polarisation, but that does not interfere with it presenting the typical interference pattern as a non-entagled polarised photon should."

As I already explained to you in some comments on the previous question about the delayed choice quantum eraser, entanglement can't get "broken" in a way that will cause Bob to see an interference pattern in the total pattern of signal photons. When you have entangled photons, measurements on one member of the pair cannot tell you anything about whether the other member has been measured or not.

To state this a little more formally, label the pair of entangled photons $a$ and $b$, with $a$ being the photon sent to Alice and $b$ being the one sent to Bob. Bob wants to know the probability that when he measures $b$, he will get some result $B$--call this probability $P(B)$. Now consider two scenarios, defined by the statements in bold:

1. When Bob measures $b$, Alice has not yet measured $a$. Thus according to the quantum formalism, we must treat Bob's measurement as collapsing the entangled 2-particle wavefunction--when measuring only one element of an entangled system, I believe the procedure is to calculate a "reduced density matrix" for that element, which can be used to give the probability of different measurement results for that element alone, without knowledge of any measurement on the other elements of the entangled system. Using this method, we calculate the probability $P_1(B)$, the probability Bob gets result $B$ given that we are dealing with scenario 1 where Alice didn't measure her particle yet.

2. When Bob measures $b$, Alice has already measured $a$. For example, say that Alice's measurement is one that can have only two possible results, $A+$ and $A-$ (like seeing if the photon passes through a polarizer at a given orientation). Either result will collapse the wavefunction in a different way, with different probabilities that Bob will get result $B$ given the new wavefunction determined by Alice's measurement--the conditional probability that Bob gets $B$ given that Alice got $A+$ can be written as $P(B|A+)$, and the conditional probability that Bob gets $B$ given that Alice got $A-$ can be written as $P(B|A-)$. Thus the total probability $P_2(B)$ that Bob gets result $B$, given only the fact that we are dealing with scenario 2 but without knowledge of which specific result Alice got, must be $P_2(B) = P(B|A+)P(A+) + P(B|A-)P(A-)$, where $P(A+)$ and $P(A-)$ can be calculated by taking the reduced density matrix for $a$ using the original entangled wavefunction, like what was done for $b$ in scenarion 1 (since here it is $a$ that is measured first).

So my statement above--"When you have entangled photons, measurements on one member of the pair cannot tell you anything about whether the other member has been measured or not"--would just boil down to the statement that $P_1(B) = P_2(B)$ in this situation, regardless of any other specific details of the experiment. Thus if Bob wouldn't see an interference pattern in the total collection of signal photons in scenario 1 where he measures first, he still won't see it in scenario 2 where Alice measured first. I can't give you a proof of this since I haven't yet studied the technical details of using reduced density matrices, but if this weren't true then it would be possible for Alice to communicate information to Bob by choosing whether or not to measure her particle before the time he is scheduled to measure his, and this has been proved theoretically impossible in QM, see the No-communication theorem.

Answer 2

Your setup with a thought to communicate FTL is impressive, but there's always quantum indeterminacy to interfere when ever an attempt for FTL is taken. Here in your setup with polarisers no matter what Alice do to her entangled photon, will never give an interference pattern on the Bobs end. Alice with no doubt can make bob a non interference pattern by measuring her entangled photons either by a vertical polariser or a horizontal polariser, but even when Alice try to make an interference pattern by measuring her entangled photons either by +45° polariser or -45° polariser there still forms a non interference patter on the Bobs end. When Alice measures her entangled photons with a 45° polariser, the photons will either have +45° polarised or -45° polarised(when photons go through they are +45° polarised if they are absorbed by polariser they are -45° polarised), if one of the entangled photons have a +45° polarisation when Alice measured, Alice would instantly know that bobs photon is also +45° polarised but by no means(unless if there is any classical approch or he directly measure that photon's polarisation) bob can know what's the polarisation does his side photon is having now, and that +45° polarised photon go through bobs double slit and forms an interference pattern, on the other hand if Alice got her one of the entangled photons as -45° polarised, then bob will also have -45° polarised photon (bob can not know whats the polarisation of his entangled photon even now just like previous case), and that -45° polarised photon goes through bobs double slit and form an interference pattern either, but now it will be out of phase of 180°, exactly conjugate to the previous interference pattern, and all the diagonally polarised photons (+45° and -45° polarised photons) will collectively make the interference pattern disappear and leaves no trace for bob to distinguish between which is an interference pattern, and which is non interference pattern. Thus no matter what by this set up Alice and bob can never communicate faster than light.

Answer 3

Please keep in mind Dirac's dictum "a particle interferes only with itself" (I am not sure about the word "particle", it may be that he said "photon").

The polarization of Bob's photon is not relevant here. Be it vertically polarized, or horizontally polarized, the fringes will fall on the same places, because one photon doesn't care of the polarization of the other. The element which plays a role in the interference here, is path-length difference between rays from the two slits. That, as long as you don't disturb the photon on its path from the slits.

However, if you introduce the distinction between the two path, the tableau will become random. Admit that Alice measures x-y polarization. So, Bob's photon comes sometimes from the upper slit, and sometimes from the lower. I am sorry, I didn't read all your proposal because I see a flaw from the very beginning. Maybe you correct it.

No FTL communication is a law of our universe.

I really hope though that I helped.