Let's do some calculus.
Suppose you have two plates, almost parallel (off by an angle $\alpha$). The plates lie in the XY plane, from $(0, 0)$ to $(x_1, y_1)$. At $x = 0$, the plates are separated by a distance $z_0$, and at $x = x_1$, the plates are separated by a distance $z_1$.
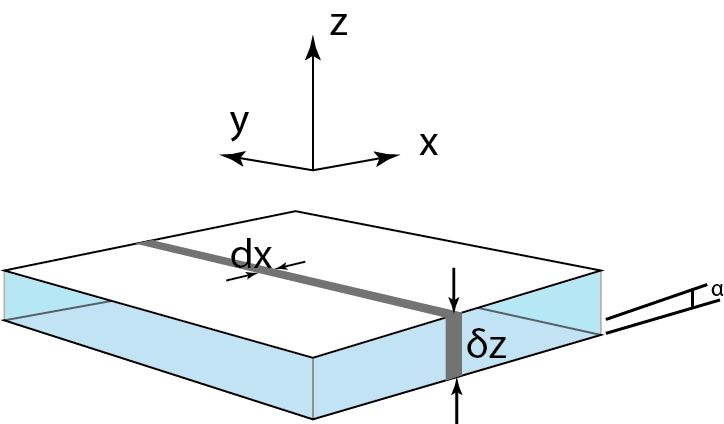
We'll now consider an infinitesimally small element of both plates. (Since parallel capacitances add, and all the infinitesimal pairs are in a parallel configuration, we can use integration)
\begin{align}
\tan \alpha &= \frac{z_1 - z_0}{x_1} \\
\mathrm{d}C &= \varepsilon \frac{\mathrm{d}A}{\delta z} \\
\mathrm{d}A &= y_1 ~\mathrm{d}x \\
\delta z &= z_0 + x \tan \alpha \\
\therefore C &= \int\mathrm{d}C \\
&= \int\limits_A \varepsilon \frac{\mathrm{d}A}{\delta z} \\
&= \int\limits_0^{x_1} \varepsilon \frac{y_1 ~\mathrm{d}x}{z_0 + x \tan \alpha} \\
&= \varepsilon ~ y \left[ \cot \alpha \ln(z_0 \cos \alpha + x \sin \alpha) \right]_0^{x_1} \\
&= \varepsilon ~ y_1 \left(\frac{\ln(z_0 \cos \alpha + x_1 \sin \alpha)}{\tan \alpha} - \frac{\ln(z_0 \cos \alpha)}{\tan \alpha} \right) \\
&= \varepsilon ~ y_1 \left( \frac{\ln(1 + (x_1 / z_0) \tan \alpha)}{\tan \alpha} \right) \\
&= \frac{\varepsilon ~ y_1}{\tan \alpha} \ln \left( 1 + \frac{x_1}{z_0} \frac{z_1 - z_0}{x_1} \right) \\
&= \frac{\varepsilon ~ y_1}{\tan \alpha} \ln \left( \frac{z_1}{z_0}\right)
\end{align}
If you assume $\alpha$ is small, then $\tan \alpha \approx \alpha$, which gives
\begin{align}
C &= \frac{\varepsilon ~ y_1}{\alpha} \ln \left(1 + \frac{x_1}{z_0} \right)
\end{align}
This conclusion is the same as the Eq. 6 in the paper you linked.

