Challenging Magnetostatics Problem - the "blind spot" of a magnetic dipole

Question

I'm reviewing for an electromag exam and I stumbled upon a problem that's really hard to figure out. Here it is:

A small magnetic dipole with moment $\vec m = m_o \hat z$ is in a region with uniform magnetic field $\vec B = B_o \hat z $. Show that there is a sphere where the net magnetic field is zero. What is the radius of the sphere?

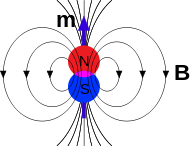
That certain sphere is what I'm referring to as the "blind spot" of a magnetic dipole. It's the first time I've encountered something like this. I believe that it is a sphere with its hemispheres on either side of the x-y plane. Why? I'm not really sure, but my argument is that the fields cancel from both sides of the hemisphere since the field lines in the close vicinity of the dipole point along its direction (you can see it from the usual sketches of the field lines of a dipole, ie a bar magnet)

I also don't think the dipole is centered, or even inside, that sphere.

However the actual calculation is really tricky. I've experimented with using the scalar potential $$\Delta \phi_m = 0 $$ but the geometrical analysis is killing me.

I'm a bit of stuck, so..... anybody out there willing to help me? Even just a rough outline of what to do will be extremely helpful. Thank you very much!!!

Answer 1

As the other answers already pointed out, there's no such spheric region. The field produced by the magnetic dipole will be given by $$\mathbf{B}_d(r,\theta)=\frac{\mu_0m_0}{4\pi}\frac{3\hat{\mathbf{r}}\cos\theta-\hat{\mathbf{z}}}{r^3}$$ So, adding both fields, we cannot make it null for a whole spherical surface, which in spherical coordinates means fixed $r$, since the component on the $z$ direction will be constant but the radial is $\theta$ dependent. What can be made, as @mcodesmart posted is to get a zero flux on a sphere. In this case, the radial field will be zero. For this we must have $$\frac{\mu_0m_0}{4\pi}\frac{3\cos\theta-\cos\theta}{r^3}+B_0\cos \theta=0\implies\\ r=\left(-\frac{\mu_0m_0}{2\pi B_0}\right)^{1/3}$$ which shows that the uniform field must be oriented in the opposite sense of the dipole.

Answer 2

After seeing the good comment of mcodesmart and the useful answer of Mateus Sampaio the question should either be looking for....

• circle with zero magnetic field

or

• sphere through which no flux passes

my original answer

That question doesn't make sense to me - I would understand if it was asking for a circular region where the magnetic field is zero. - To have a sphere with zero magnetic field implies that the sum of the two magnetic fields is zero at all points inside (or on the surface) of a sphere. As the uniform magnetic field is uniform and parallel it means that the small dipole must generate a sphere of uniform magnetic field in exactly the opposite direction, which it surely cannot - It can however generate a circle of magnetic field in exactly the opposite direction of equal magnitude at a distance $r$ from the centre of the dipole in the $xy$ plane. The challenge then would be to find $r$ in terms of $m$ and $B$.

I think the question must mean a circle because the dipole is aligned with the uniform field - both going in the $z$ direction.- so the magnetic field lines from the dipole will only be in the $-z$ (negative $z$) direction and able to cancel it out in the this $xy$ plance which includes the centre of the dipole.

In this picture from wikipedia the dipole is in the +ve z direction and in the xy plance centred on the dipole there is magnetic field due to the dipole in the opposite $-z$ direction.