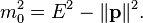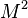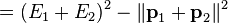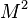Intuitive explanation of how hadron mass emerges from the strong force

Question

I'm not familiar with QCD, but I'm looking for intuitive explanation of this phenomenon (it could be that easy explanation does not exist).

What I've read is that large part of hadron masses arises from strong interactions between constituent quarks and gluons.

Of course quarks are massive, but sum of their masses is much smaller than the mass os composite hadron. Moreover, using simplified QCD calculations - with massless quarks - we get, as a result, also a massive particle.

It seems that hadron mass (at least most of it) i a result of some interactions between quarks and gluons inside of it.

My question is what kind of energy we see as the rest mass of hadrons? Also how is it connected to phenomenon called "chiral symmetry breaking"?

Answer 1

To start with, special relativity is necessary when describing elementary particles and hadrons composed by them.

Special relativity defines the invariant mass of a particle or an ensemble of particles as the measure of the four momentum vector carried by the particle/ensemble.

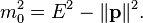
In the case of one elementary particle, an electron for example, it is called the rest mass and characterizes the elementary particle.

For composite particles, as the proton and neutron, even at a naive level one cannot imagine that the three quarks are at rest in the nucleon, i.e. have no four momentum vector characterizing them. Adding four momenta does not add the masses, for example in a two particle system:

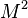
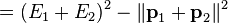
suppose the dot product of the momenta is zero it is clear that the higher the energy the particles have, the larger the invariant mass.

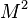
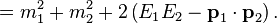
Now our studies have shown that the proton is not only composed by the three quarks and their four momenta , but also by a sea of gluons and quark antiquark pairs which will be adding their four momenta to define the total proton four momentum and the proton invariant mass. Thus it should be intuitively clear that the mass of the proton will be much larger than the mass of its constituent quarks since a lot of four momentum vectors are involved.

The problem then naively becomes : why does the proton have a fixed mass if there exists this soup of gluons and quark antiquark pairs in the bag defining the proton? That is where chiral symmetry breaking comes in because it gives the underlying theory that defines the way the constituents build up the hadrons as QCD bound states, and as we know bound states have unique energy.