Maximum friction force for a wheel to be able to roll [duplicate]

Question

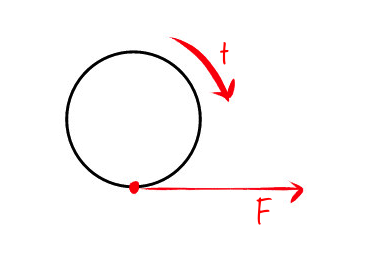
The wheel with mass $M$ and radius $R$ below is free in space (it is not on the ground). A torque $\tau$ is applied to it through an engine. A horizontal force $F = \frac{\tau}{R}$ is also applied to it on its bottom-most point. I work out the net torque on the wheel by

$$\tau_{net} = \tau - \frac{\tau}{R}R = 0$$

Since the net torque acting on the wheel is zero, I expect the wheel to slide to the right with linear acceleration $a = \frac{\tau}{MR}$, and to not roll (due to zero torque).

Now, another wheel (below) with radius $R$ is on the ground, and an engine applies a torque $\tau$ to it. The ground applies a force $F_{friction}$ in order to prevent the wheel to slip. The wheel translates to the right, but this time let's say that it rolls with a bit of slipping.

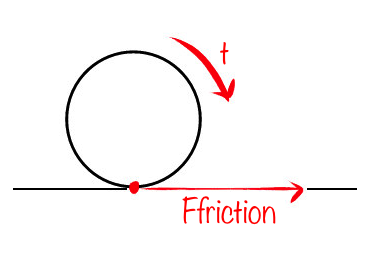
From this, can I assume that the friction force $F_{friction}$ the ground applies to the wheel is always smaller than $\frac{\tau}{R}$? Because, from what I see above, if friction is equals to this $\frac{\tau}{R}$, the wheel would not roll at all.

Answer 1

Since the net torque acting on the wheel is zero, I expect the wheel to slide to the right with linear acceleration $\alpha = \tau MR$, and to not roll (due to zero torque).

Be careful. There are subtleties here. This will not necessarily be true in an accelerating frame of reference. In your particular case since it holds for the axis that goes through the center of mass, it will be okay. But you may need to keep that in mind when the object is accelerating.

The wheel translates to the right, but this time let's say that it rolls with a bit of slipping.

It won't really matter if it's slipping or not. You can do the same analysis with it not slipping because the wheel is changing rotational speed even in the no-slip case.

can I assume that the friction force $F_{friction}$ the ground applies to the wheel is always smaller than $\tau R$? Because, from what I see above, if friction is equals to this $\tau R$, the wheel would not roll at all.

Basically correct. Although it's not that "the wheel would not roll", it's that "the wheel will not roll faster over time". If the torques were equal, the wheel would roll along at a constant speed. But the torque from the engine must be greater to make it accelerate.

From this, you do indeed know the frictional force is less than the force required to null the engine torque.