I think I've get a better idea of what you are looking for now, thanks.
As background for others in the future: classic ion-solid interaction theory dates back to the 1960s and is commonly called LSS theory after Linhard, Scharff, and Schiott who first formulated the concepts. It splits the energy loss mechanisms of the ion into two components, electronic stopping (ion-electron interactions), and nuclear stopping (ion-nuclei interactions). There are many ion-electron interactions, and the maximum energy transferred by any one of them is small, so this acts generally as a viscous drag force on the ion, giving an increasing angular spread to the beam as it travels through the solid. The ion-nuclei interactions are what Geiger and Marsden saw: Rutherford scattering. The larger the scattering angle, the greater the energy transferred to the nucleus from the incident ion (simple kinematics).
LSS theory allows one to calculate the range and distributions for ions implanted into solids. Many folks use SRIM from John Ziegler (srim.org) to get range, stopping, and perform simulations of ion implants. Note one should take the absolute numbers from SRIM with at least a grain of salt, since it doesn't handle channeling effects.
For MeV H into almost any material, the overwhelming energy loss mechanism, on average, is electronic stopping. From SRIM one can get that 99+% of the energy loss of 2MeV hydrogen into B, P, or Au is ionization (electronic stopping) as averaged over many incident ions. Those nuclei are small! Nuclear cross sections are measured in barns, or $10^{-24} cm^2$.
As an example: MeV H or He is regularly used in Rutherford Backscattering Spectrometry (RBS) for materials composition analysis of thin films. Often one uses about 20nC of ions, which is about 125,000,000 ions hitting the film. Not that many bounce back.
A (simplified) equation for the differential Rutherford cross section comes from Chu, Mayer, and Nicolet, 'Backscattering Spectrometry' (1979). It is:
$\sigma(\theta) = ({{Z_{1}Z_{2}e^{2}} \over {2E_{0}}})^{2} {{(cos(\theta) + \sqrt{1-x^{2} sin^{2}(\theta)}})^{2} \over sin^{4}(\theta)\sqrt{1-x^{2} sin^{2}(\theta)}}$
where $x = m_{1}/m_{2}$ and $E_0$ is the incident energy. This is in units of barns/steradian (cross section to be deflected into solid angle at $\theta$, in lab coordinates).
How big are these numbers? Well, for 1MeV H on B, $\sigma(175^{\circ}) = 0.0319$ barns. For fun I'll plot the Rutherford cross sections for 2MeV H onto B, P, and Au:
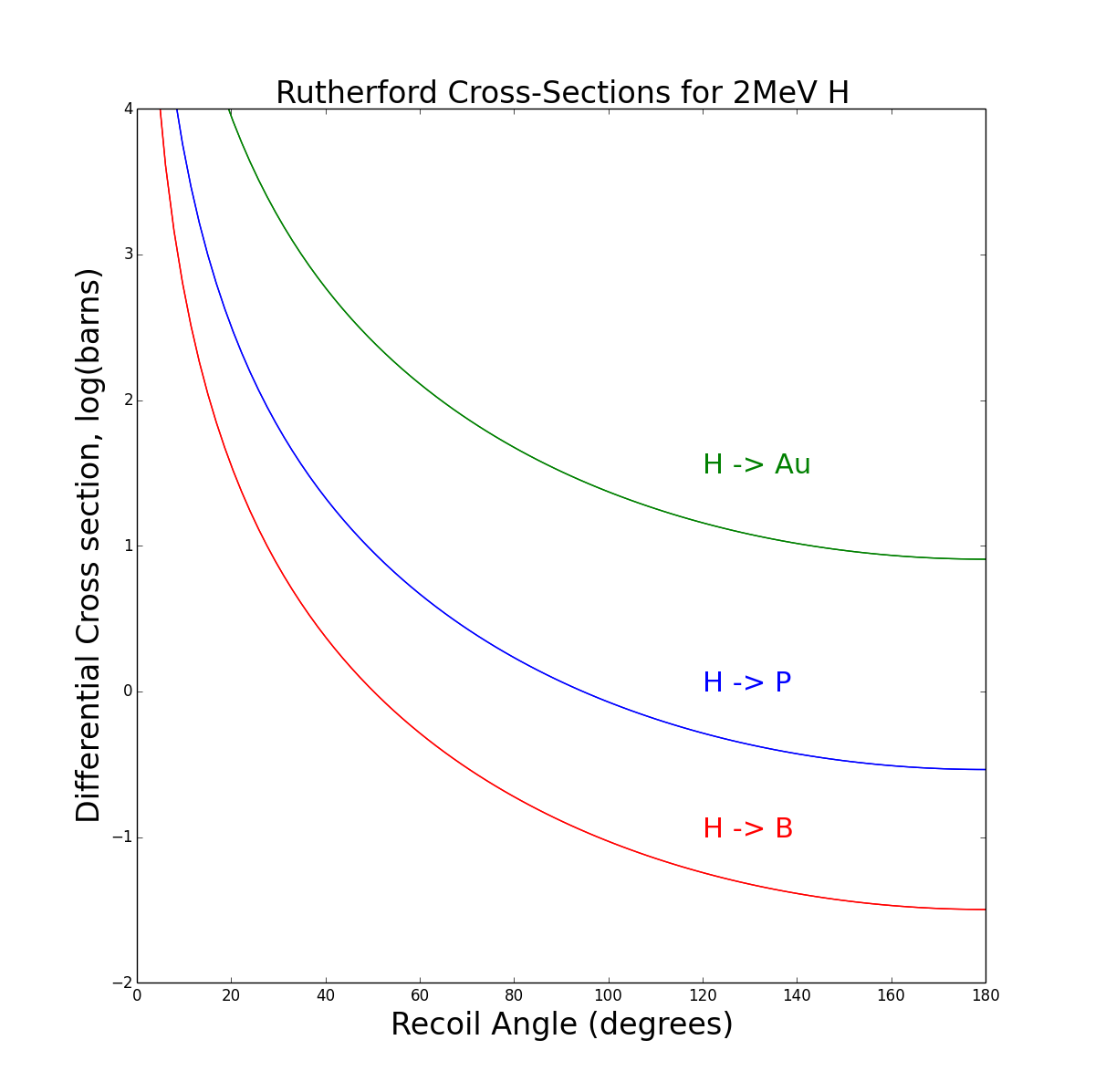
Large recoil angles are large energy transfers to the target nucleus. As you can see, such large transfers are much much much less likely than small energy transfers at these energies. Now, as the incident ion loses energy (through electronic or nuclear stopping), you can see from the formula that the cross sections go up. Near the end of range, multiple nuclear collisions become fairly normal, but the total energy transferred is pretty low (remember, 99+% of the incident H energy is going into electronic stopping).
So, multiple high-energy-transfer collisions are only likely at low ion energy, i.e. already near their end of travel - such multiple scatterings should not dramatically affect the straggle. This is caused much more by spreading from electronics stopping, a few low angle collisions, and a very few high angle collisions. (Now, lower energy heavy ions, such as 100keV Au->Au will show multiple large-angle collisions since the cross sections are much bigger).

