Skin depth of current density in magnetic conductor at boundary between two different materials

Question

Imagine a magnetic conductor with a cylindrical cross section, surrounded by a coil with a time varying current of $$I = I_0\cdot \cos (2\pi f t)$$ The conductor is split into two parts, the first with a conductivity and a relative permeability of $\kappa, \mu$, the second with $4\kappa, \mu$. There is a magnetic field $B$ through the conductor, which is caused by the current and therefore time varying as well: $$B = B_0\cdot \cos (2\pi f t)$$

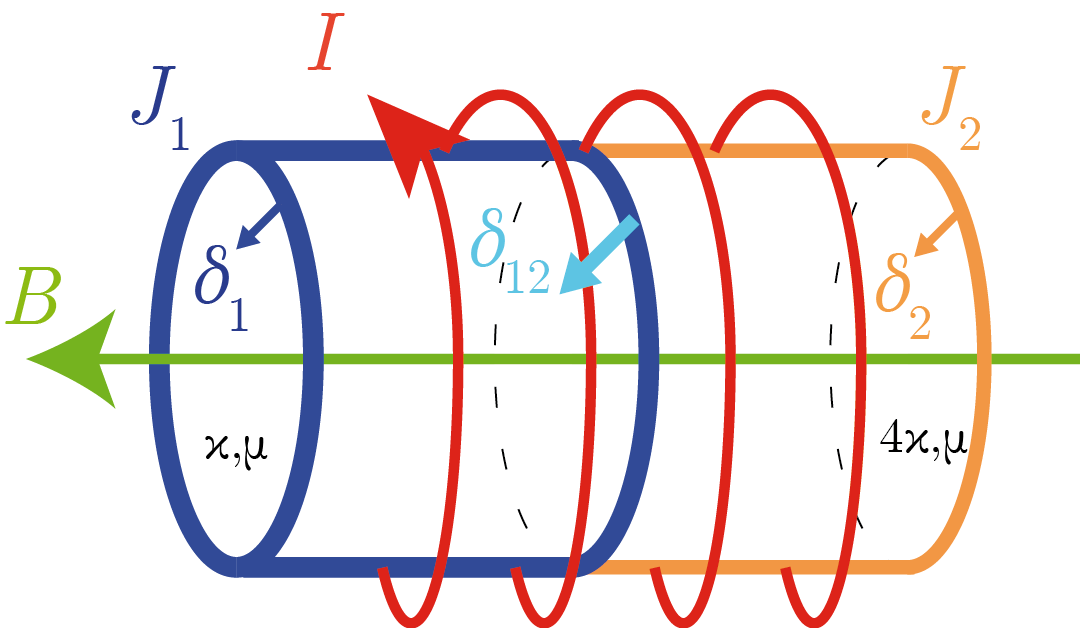
The change of this magnetic field induces a voltage inside the material and causes a current density $J$. This current density has the value $J_1$ on the surface of the left conductor and $J_2$ on the right side.

The skin depth $\delta$ is defined by the distance from the surface where $J = 0.37 \cdot J_1$, respectively $J = 0.37 \cdot J_2$ , with $0.37 = 1/e$ and also:

$$\delta = \frac{1}{\sqrt{\pi f\kappa\mu}} = \frac{\sqrt{2j}}{\alpha}$$

where a $\alpha$ is the propagation constant. I found out by simulation, that at the boundary between both materials, the blue one, and the orange one, applies:

$$\frac{1}{\delta_{12}} = \frac{1}{2}(\frac{1}{\delta_{1}}+\frac{1}{\delta_{2}})$$

and therefore

$$\alpha_{12} = \frac{1}{2}(\alpha_1 + \alpha_2)$$

But I'm really struggling to prove that. Can someone give me some hints, how I could get these relations analytically?

---

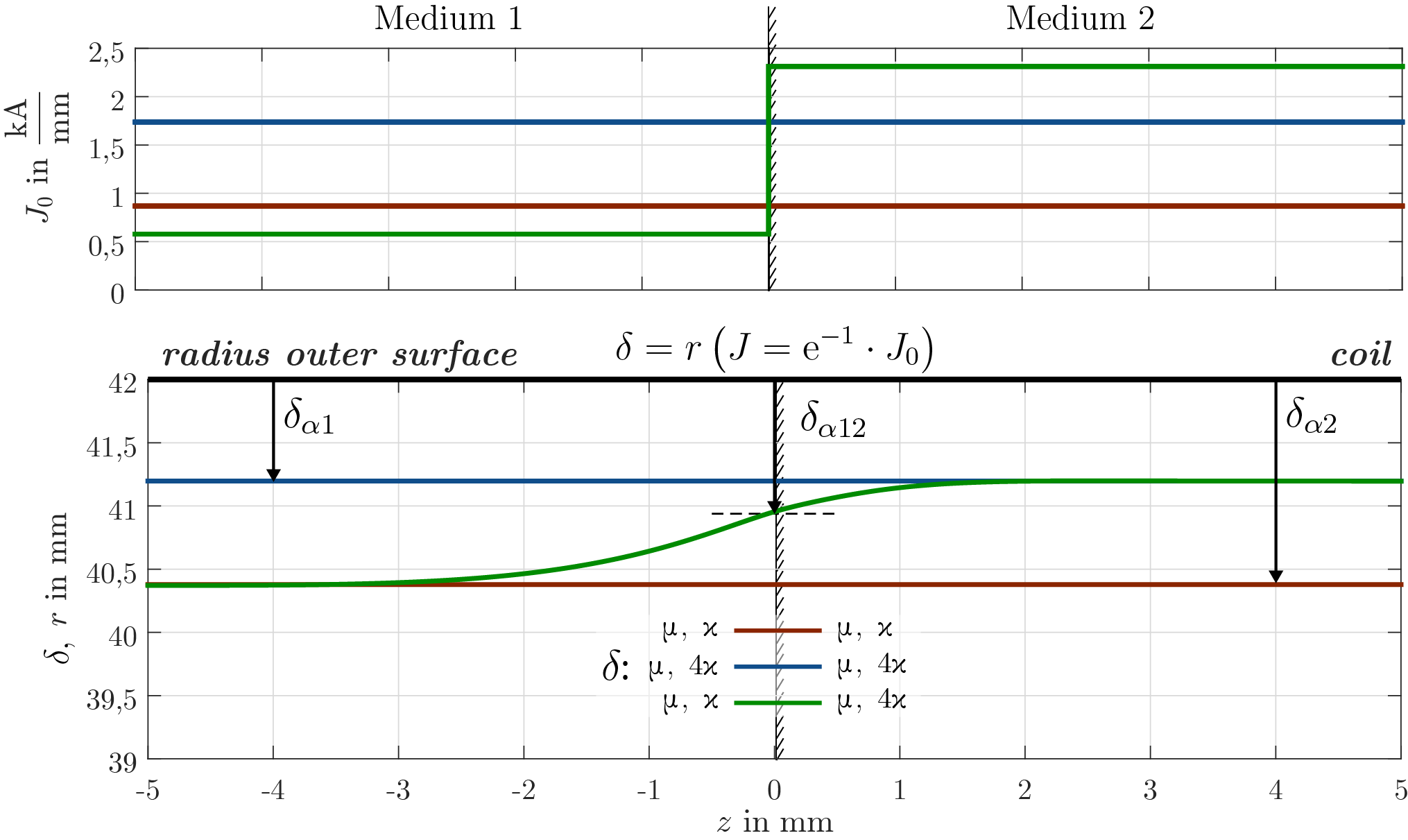
Here another plot:

The upper one shows the current density at the surface. The second one shows the contour line where the current density decreased about $63\% = skin depth$. At $z=0$ is the boundary between both materials. Though the current density is a step function, the skin depth is continuous and has the value $\delta_{12}=\frac{2}{\frac{1}{\delta_{1}}+\frac{1}{\delta_{2}}}$ at $z=0$.

Answer 1

Well, I hope I didn't go too much off track. I'm open for discussion.(possible solution path is on the bottom)

Looking at the formula for $\delta$ I see that it is actually related to the speed of EM waves in the medium. The speed of propagation for a medium with the properties $\kappa_1$ and $\mu_1$ is: $$c_1=\frac{1}{\sqrt{\kappa_1\mu_1}}$$ Therefore we can rewrite it as: $$\delta=\frac{1}{c\sqrt{\pi f}}$$ Looking at it, I would like to have the $\omega$ in it rather than $f$: $$\delta=\frac{\sqrt{2}}{c\sqrt{\omega}}$$ Lets square it: $$\delta^2=\frac{2}{c^2\omega}$$ What stays same, I guess its $\omega$: $$\omega=\frac{2}{c^2\delta^2} $$ The frequency is the same everywhere, so it is: $$\frac{1}{c_1^2\delta_1^2}=\frac{1}{c_2^2\delta_2^2} $$ or: $$\frac{c_2}{c_1}=\frac{\delta_1}{\delta_2} $$ So now we actually reduced it to a problem of finding the speed of propagation of EM-waves at the interface. That will not help directly but it gives me another idea.

I would now suggest to look at it similar to a semiconductor PN-junction problem, and calculate the change of $\kappa$ on the interface due to the differences in charged particle density. The drift currents will do the charge compensation on the interface.

So the diffusion current is: $$I_{diff}=qD\frac{dn}{dx}$$ Using this formula you get some current, this affects the local $\kappa$ and creates a $\kappa'$. Using this value you should get the $\delta$ on the boundary(and even the functional dependence on the distance from the interface).