Highly diminished image of an object placed at infinity

Question

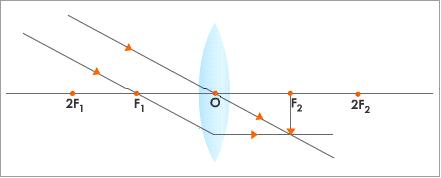
Consider an object at infinity. The rays coming from it are parallel to each other. Let one of the parallel rays pass through the focus $F_1$ of a thin lens, and let a second ray pass through the optical center $O$. The ray which passes through $F_1$ becomes parallel to the principal axis after refraction and the ray which passes through the optical center does not suffer any deviation.

We therefore get the image as formed at $F_2$, inverted, real and highly diminished.

My question is: without comparing the size of the image with the size of the object placed at the infinity, how can we say that the image is highly diminished?

Answer 1

If the object is at infinity, lies across the axis and some rays from it are coming in at a non zero angle, then the object is infinite. A finite image of the object is then highly diminished.

Answer 2

First of all the infinity used in optics is not the type of infinity in mathematics. 1000 meters for an ant is also infinity for it! Similarly for a small lens ( of focal length 10cm-25cm ) 1 Km is infinity.

Just bulge the rays a little outward from the left side of the optical centre, when you extend them, the point where they will meet will be really high from the principle axis. So if we compare the two heights, we say that it’s highly diminished ( compared to the object off course )