I mean, what is happening at a microscopic level to cause this behavior? Here's what I got from Wikipedia:
- On Reflection (physics)#Reflection of light it says that "solving Maxwell's equations for a light ray striking a boundary allows the derivation of the Fresnel equations, which can be used to predict how much of the light reflected, and how much is refracted in a given situation."
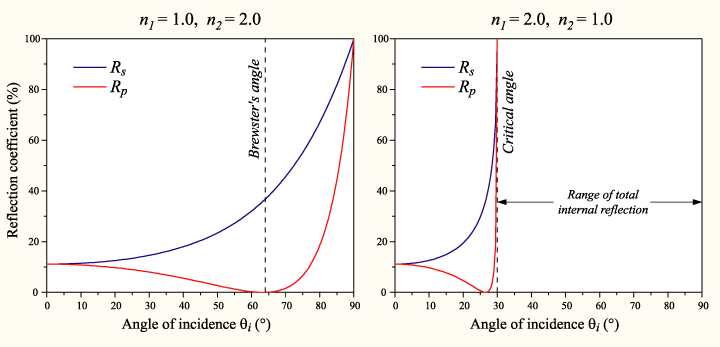
- On Specular reflection#Explanation it says that "for most interfaces between materials, the fraction of the light that is reflected increases with increasing angle of incidence $\theta_i$" (but doesn't explain why)
- Finally, on Reflection coefficient#Optics, it says basically nothing, redirecting the reader to the Fresnel equations article.
What I'm trying to find, instead, is a basic level explanation that could provide an intuition on why this happens, rather than analytic formulations or equations to calculate these values. Is there a good analogy that explains this behavior?