55
$\begingroup$

Imagine we have cup A with 50 g of water and cup B (smaller in width than A) with 100 g of water. Now put cup B into cup A. If the width of both cups are of comparable size then the cup with 100 g of water floats. It does not touch the bottom of cup B.

Now think about Archimedes law of flotation. It says that the weight of displaced liquid = weight of the floating object. However in this case the bottom cup has only 50 g of water. How can an object float without displacing water equal to its own weight? Am I not applying Archimedes principle correctly or because of both things beings of comparable size Archimedes principle does not apply?

Improve this question
$\endgroup$
9
  • 1
    $\begingroup$ Are you sure it floats? This is really interesting if true. $\endgroup$
    – pho
    Commented Apr 6, 2014 at 1:06
  • 4
    $\begingroup$ Is the cup lighter that water? If so the total density will be less than that of water and hence it floats. It is not about weight, but about density. $\endgroup$
    – John Alexiou
    Commented Apr 6, 2014 at 1:13
  • 2
    $\begingroup$ You should probably draw or take a picture. It can either float or sink, depending on the density of the cup, as well as the distance between the liquid surface in cup B and the rim of cup B, so as stands, the question is not really well-defined. $\endgroup$
    – DumpsterDoofus
    Commented Apr 6, 2014 at 1:27
  • 5
    $\begingroup$ Oh wait, never mind. I see what your question is: you're asking how it's possible that the cup with 100 g water floats, even though Archimedes principle claims that it should displace 100 g of water, which seemingly contradicts the fact that there's only 50 g of water in cup A to be displaced. There is a subtle but clever resolution to this. I'll post it in a bit. $\endgroup$
    – DumpsterDoofus
    Commented Apr 6, 2014 at 1:31
  • 2
    $\begingroup$ "on" in the question title should probably be "in". $\endgroup$
    – naught101
    Commented Apr 7, 2014 at 12:23

4 Answers 4

Reset to default
71
$\begingroup$

As best I can tell, what you're confused about is the fact that Cup A (weighing 100 g) is floating in 50 g of water, while Archimedes principle states that Cup A ought to be displacing 100 g of water, which seems to contradict the fact that there's only 50 g of water available to displace. How can that be possible?

There is a subtle reason; just because you have 50 g of water doesn't mean you can't effectively displace more than 50 g of water. This is probably best illustrated with a picture. Here's what the system looks like before Cup B is dropped in:

Enter image description here

Here's what it looks like when you drop in Cup B:

Enter image description here

The tricky thing is: Cup B effectively displaced 100 g of water, even though there was only 50 g of water available to displace! If it's not immediately obvious how it is that Cup B is displacing 100 g of Cup A's water (even though Cup A only has 50 g of water), stare at diagram 2 for a while.

Improve this answer
$\endgroup$
14
  • 6
    $\begingroup$ oooo, pretty pictures, I should have done that. $\endgroup$
    – LDC3
    Commented Apr 6, 2014 at 2:02
  • 5
    $\begingroup$ I was under the impression that the displaced water means the actual water that is displaced. Normally in these demo of Archimedes principle they use a bucket full of water and drop some object slowly and let the water spill and collect the spilled water and show that that mass is the same as the mass of the object. In that sense i thought the displaced water was the actual water that is displaced from the system but in this case it turns out to be the effective displacement? Am I saying this correctly? $\endgroup$
    – quantum
    Commented Apr 6, 2014 at 2:42
  • 4
    $\begingroup$ Related old brain teaser: How much dirt is in a 1x1x1' hole? $\endgroup$
    – Ben Jackson
    Commented Apr 6, 2014 at 6:54
  • 6
    $\begingroup$ @quantum To see why the "displaced" water doesn't actually have to be present, cut the outer cup at the height is will obtain once the inner cup is floating and then fill it completely. As we lower the inner cup, water will spill over the outer rim until it floats. If we capture the spill it will have a mass of 100g (from the weight of displaced water version of Archimedes principle). If we now remove the inner cup there is only 50g of water left, but we can surely "un-remove" it and so we are confident that it will float in only 50g of water as long as those 50g are properly confined. $\endgroup$
    – dmckee --- ex-moderator kitten
    Commented Apr 6, 2014 at 14:25
  • 19
    $\begingroup$ How to Physics: Stare at appropriate diagram for a while. :P $\endgroup$
    – naught101
    Commented Apr 7, 2014 at 10:07
9
$\begingroup$

Let's assume that cup B has a mass of 1 g, so the total mass it needs to displace for it to float is 101 g. When cup B is placed in cup A, the level of the water rises. With cup B floating, we mark the level of the water in cup A. We take out cup B and notice the level of water is lower. If we now add water to cup A to bring it up to the earlier level, we would need to add 101 g of water.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I think what you said is true but that does not answer my question? Does displaced volume of displaced water in Archimedes principle mean the actual water displaced by the object or is it just the volume of water that is equivalent to the volume of object under water. Archimedes principle says volume of displaced water= volume of object under water but my confusion regarding the above question is what we actually mean by displaced water in this context $\endgroup$
    – quantum
    Commented Apr 6, 2014 at 1:16
  • $\begingroup$ What I was pointing out was that the water needed to fill to the same level is the displaced water so that cup B will float. $Does displaced volume of displaced water in Archimedes principle mean $ $the actual water displaced by the object ...?$ Yes it is the actual water displaced. $... or is it just the volume of water that is equivalent to the volume of object under water?$ It is the same. $\endgroup$
    – LDC3
    Commented Apr 6, 2014 at 1:32
2
$\begingroup$

Depth is what matters here.

  1. It is a given that a partially filled cup will float in a body of water. Of course, that body just has to have enough depth to contain the immersion. If the cup requires X centimeters of depth in order to float, and the surface of the water is > X centimeters above the bottom of the larger container (taking into account the displacement caused by the immersion), then the cup will float.

  2. The depth of the water is not restricted by the available volume. 50 grams of water can be displaced in such a way that sufficient depth is created to float a cup of 100 grams.

Depth, together with density and gravity is what actually creates the pressure that causes buyoancy. Each unit of area of the object is acted upon by pressure, and the net difference in pressure between the upper and lower parts of the object creates buyoancy: because the parts of the object's surface which are deeper are subjected to greater pressure than the parts of the object's surface which are immersed less deeply.

Archimedes' Law is just a corollary which arises from this pressure gradient. Because of the way the surface integral works out around an object, a short-cut pops out from the mathematics: the buoyant force can be obtained knowing just the gravitational force which acts upon the equivalent volume of the fluid in which the object floats. This is usually stated as "the volume displaced by the object", but the displacement is an abstraction: there might not be enough fluid available such that when the buoyant object is removed, its entire volume is filled by the fluid that remains.

The "displacement visualization" of buyoancy assumes that the body of water is large enough that the availability of fluid is practically unlimited. But buyoancy does not depend on actually pushing out all the fluid out of the object's space; it's just that: a visual aid.

This is all related to the fact that the pressure in a column of fluid under gravity is irrespective of the width of that column and therefore its volume. A column of water 10m high, and as thin as a pencil, has the same pressure at the bottom as a 10m deep lake (aobut 1 atmosphere). This is why only a thin jacket of water around a paper cup is enough to create the pressure to float that cup.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks everyone for the answers. Its clear why the object floats. The point behind asking this question is to clarify the idea of displaced water when talking about Archimedes principle. Normally in Physics demo, we full the bucket with water and let it spill then collect it and measure its mass and volume to show that Archimedes principle holds. In that sense I always thought the displaced water as being water displaced but in context like these where water level chances displaced water will be the effective volume of water that is equal to the volume of object under water. $\endgroup$
    – quantum
    Commented Apr 8, 2014 at 4:07
1
$\begingroup$

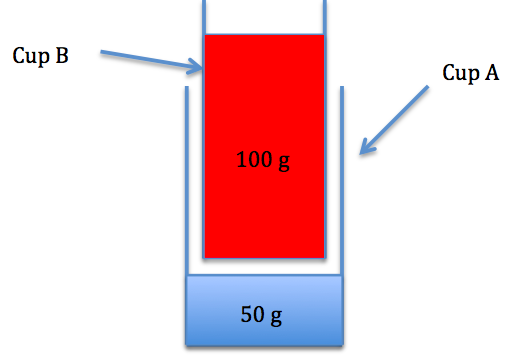
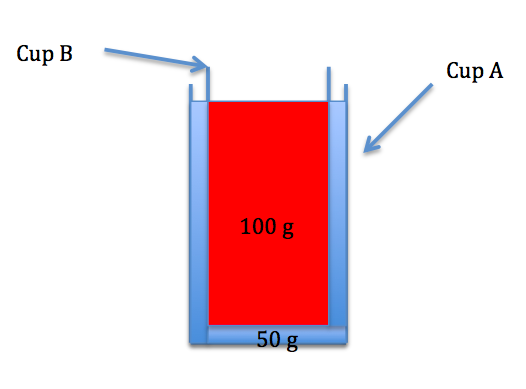
How about this experiment:

Cup A is full of water to the rim. You place cup B filled with some water to float in cup A. Most of the water of cup A spills over the rim except for a tiny amount. However cup B finally floats in cup A. You pull the floating cup B out of cup A.

  • How much water spilled from cup A?
  • How much volume of water was spilled?
  • Will cup B float again, if you put it back?

Bonus questions:

  • By how much rises the water level, if you put a stone (smaller but as heavy as cup B) into cup A?
  • Why doesn't the stone float?
  • How much water do you need to fill into a cup C to make cup B float in it, if cup C equals cup A except for a higher rim?
  • How much (more) volume of water would you need to fill into cup D to make cup B float in it, if cup D would be wider than cup A?

I guess the confusion arises from measuring water by its weight instead of its volume. The water spilled from cup A is the volume of water displaced by cup B. But you don't need to actually spill it. It's just important that the water level rises as much as if it was spilled.

And now to answer your question: You are applying the term "displace" incorrectly, because you relate it to the water that is still there. But the meaning is that it is not there, e.g. the spilled water. However spilling water is only a way to visualize that something is not there.

In short: Displacement does not mean "spilling", but raising the water level because a certain "volume is pushed aside".

BTW: The last paragraph in the marked answer is kind of critical:

The tricky thing is: Cup B effectively displaced 100 g of water, even though there was only 50 g of water available to displace!

The last verb shouldn't be "displace" but "push aside", otherwise it's the same erroneous way of thinking.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged or ask your own question.