Accelerate the car by static friction

Question

I asked this question about friction and slip ratio and now I still don't understand it

Why friction is zero when wheel slip is zero?

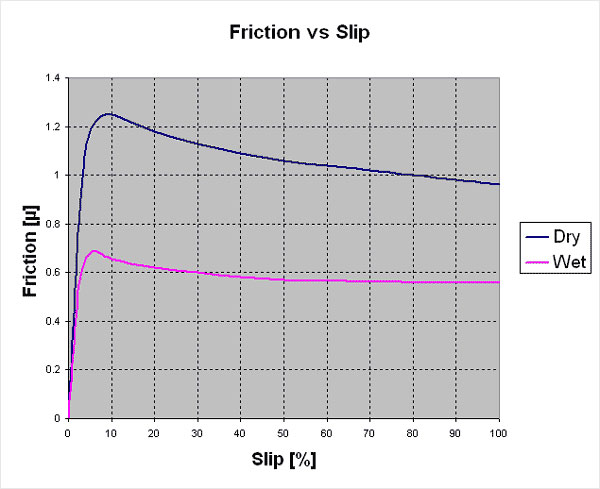
As the below graph of wheel friction vs slip ratio shows that in order for the car to has acceleration, its driving wheels must slip. No slip means no acceleration. Almost all graph I searched showed the similar concept

But then there is this question which says that a train is only accelerated by static friction, which means a non-slip wheel still can accelerate the vehicle.

Static Friction - Only thing that can accelerate a train?

My understanding of physics favors this later model for car as well. But this model is completely wrong: no slip means no (kinetic) friction and no acceleration

But why can't a tire accelerate a car when there is no slip, via static friction?

Let say a car moving at a constant speed, the tire has no slip. Now the engine accelerates the tire, the tire will roll faster. If this accelerated force is under the static friction limit, then the tire will not slip, but it still can accelerate the car. But according to reality, a la every friction vs slip ratio graph, this model is completely wrong. No slip or a very low slip ratio means no acceleration at all

Could anyone explain it for me?

And is there any difference between steel wheel and rubber tire? IMO, there isn't any from physical point of view. Two models above, one must wrong

Answer 1

The slip ratio depends on the speed for the car you would calculate based circumferential speed of the wheel in the frame of the car (angular velocity of wheel times radius), and the actual linear speed of the car.

The reason that you get slip at even the smallest forces results not from the fact that the tire is slipping against the ground, but that the tire is elastic. Let's see how this could happen.

To measure the slip, lets put little green splotches of die on the circumference of the tire spaced 1cm apart. From this we can tell how much the wheel has rotated. Now imagine a car that is accelerating. What happens to the tires? Well the road is providing a force on the tire. What does that do to the bottom of the tire? Well just imagine a stationary tire that can't rotate and you apply a force tangent to the tire. This will cause the tire to deform and the part of the tire you are apply the force to will get scrunched up in the direction of the force. Now if you force the tire to rotate against the force, the scrunched up part will go to where the "ground" (the thing applying the force) is.

This means that our little green splotches, instead of being 1 cm apart, they will be .8 cm apart. Suppose there are a total of 11 splotches. Then by the time tire turns enough for each splotch gets to its original position, the wheel has rotated a full revolution. On the other hand, the car has only moved 8cm, because each of the splotches is .8 cm apart and there are 11 of them (so 10 intervals). Now when we compare this 8cm that the car has actually moved while the wheel rotated once to the full circumference of the wheel, which is 10 cm, we conclude that the wheel has slipped.

Since there will always be some scrunching given a non-zero tangent force, you will always get slip for a nonzero tangent force.

Of course this scrunching goes to zero in the limit of an infinitely rigid wheel, which is the sort of wheel used in physics homework problems.

Now for high enough slip ratios, the wheel will actually slide across the pavement, but until you get to the this point, static friction is still in play, so the car is accelerating from static friction.