When measuring blood viscosity, the literature claims that we generally use a cone-and-plate viscometer. Why is this; is there any way to explain this mathematically in terms of the shear rate, etc? Does it have to do with the fact that the shear rate is constant in this device? Why won't it work with a parallel-plate viscometer?
$\begingroup$
$\endgroup$
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
4
As you say in your question, the advantage of a cone and plate viscometer is that the shear rate is constant and therefore well defined. If you use parallel plates the shear rate increases lineraly with distance from the centre.
The reason this matters is that many fluids are shear thinning, or to use the more precise term non-Newtonian. This means the viscosity is a function of shear rate, and to measure this function accurately you need a precisely defined shear rate. This cannot be done with a parallel plate viscometer.
One quick Google later I find that blood is indeed a non-Newtonian fluid.
Response to comment:
This is an illustration of a cone and plate viscometer:
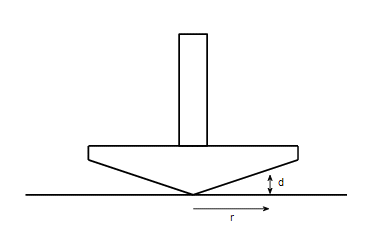
Shear rate is velocity divided by separation. If the cone is rotating at an angular velocity $\omega$ then the velocity at the point indicated is $v = r\omega$. If the cone angle is $\alpha$ then the separation is $d = r \tan \alpha$, so the shear rate is:
$$ \text{shear rate} = \frac{r\omega}{r\tan\alpha} = \frac{\omega}{\tan\alpha} $$
So the shear rate is independant of $r$ so it's constant everywhere across the cone, which is what we want. For a given cone angle the shear rate just depends on the angular velocity $\omega$.
answered Mar 16, 2014 at 16:29
-
$\begingroup$ Well, what if you measure the blood viscosity in the parallel-plate viscometer at a fixed radius. In that casem the shear-rate wouldn't change. So, would that allow you to meassure blood viscosity? Either way, I think that the way to measure it is to measure viscosity at several different constant shear-rates in the cone-and-plate viscometer, correct? $\endgroup$ Commented Mar 16, 2014 at 16:38
-
1$\begingroup$ @user42668: how do you propose to do that? A viscometer measures the angular velocity and torque. Typically viscometers apply a torque and measure the resulting angular velocity. I can't see how you would only measure the contribution to the torque from the blood lying at some specified distance from the centre. $\endgroup$ Commented Mar 16, 2014 at 16:40
-
$\begingroup$ What would that shear rate actually be? Can we use a velocity distribution found from the NS equation to determine the shear rate? $\endgroup$ Commented Mar 17, 2014 at 20:37
-
$\begingroup$ @user42668: I've edited my answer to show how to calculate the shear rate. $\endgroup$ Commented Mar 18, 2014 at 6:46