Can black holes form in a finite amount of time?

Question

One thing I know about black holes is that an object gets closer to the event horizon, gravitation time dilation make it move more slower from an outside perspective, so that it looks like it take an infinite amount of time for the object to reach the event horizon. It seems like a similar process should slow the formation of the black hole itself: As the star collapses, its gravitational time dilation make itself collapse more slowly. This make me wonder, are what astronomers claim to be black holes really black holes, or are they stars that progressively make themselves more similar to one without actually reaching the stage of having an event horizon?

EDIT: Contemplating one answer, I realize the question is ambiguous. What does finite time mean in general relativity. Here is a less ambiguous question: Is there a connected solution of 3+1 dimensional general relativity with one space-like slice not have a singularity, and another space-like slice having one.

Answer 1

The conceptual key here is that time dilation is not something that happens to the infalling matter. Gravitational time dilation, like special-relativistic time dilation, is not a physical process but a difference between observers. When we say that there is infinite time dilation at the event horizon we don't mean that something dramatic happens there. Instead we mean that something dramatic appears to happen according to an observer infinitely far away. An observer in a spacesuit who falls through the event horizon doesn't experience anything special there, sees her own wristwatch continue to run normally, and does not take infinite time on her own clock to get to the horizon and pass on through. Once she passes through the horizon, she only takes a finite amount of clock time to reach the singularity and be annihilated. (In fact, this ending of observers' world-lines after a finite amount of their own clock time, called geodesic incompleteness, is a common way of defining the concept of a singularity.)

When we say that a distant observer never sees matter hit the event horizon, the word "sees" implies receiving an optical signal. It's then obvious as a matter of definition that the observer never "sees" this happen, because the definition of a horizon is that it's the boundary of a region from which we can never see a signal.

People who are bothered by these issues often acknowledge the external unobservability of matter passing through the horizon, and then want to pass from this to questions like, "Does that mean the black hole never really forms?" This presupposes that a distant observer has a uniquely defined notion of simultaneity that applies to a region of space stretching from their own position to the interior of the black hole, so that they can say what's going on inside the black hole "now." But the notion of simultaneity in GR is even more limited than its counterpart in SR. Not only is simultaneity in GR observer-dependent, as in SR, but it is also local rather than global.

Is there a connected solution of 3+1 dimensional general relativity with one space-like slice not have a singularity, and another space-like slice having one.

This is a sophisticated formulation, but I don't think it succeeds in getting around the fundamental limitations of GR's notion of "now." Figure 1 is a Penrose diagram for a spacetime that contains a black hole formed by gravitational collapse of a cloud of dust.[Seahra 2006]

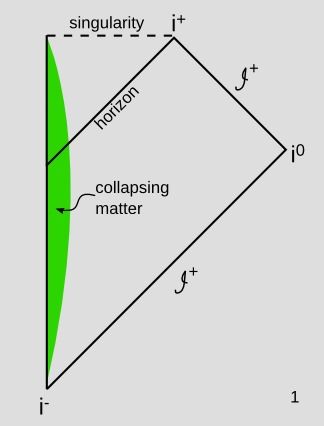
On this type of diagram, light cones look just like they would on a normal spacetime diagram of Minkowski space, but distance scales are highly distorted. The upright line on the left represents an axis of spherical symmetry, so that the 1+1-dimensional diagram represents 3+1 dimensions. The quadrilateral on the bottom right represents the entire spacetime outside the horizon, with the distortion fitting this entire infinite region into that finite area on the page. Despite the distortion, the diagram shows lightlike surfaces as 45-degree diagonals, so that's what the event horizon looks like. The triangle is the spacetime inside the event horizon. The dashed line is the singularity, which is spacelike. The green shape is the collapsing cloud of dust, and the only reason it looks smaller at early times is the distortion of the scales; it's really collapsing the whole time, not expanding and then recontracting.

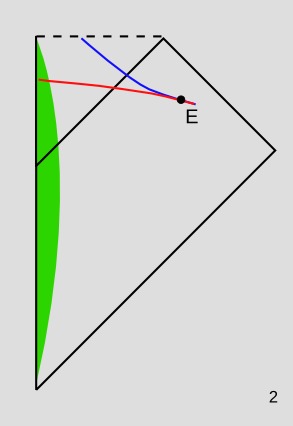
In figure 2, E is an event on the world-line of an observer. The red spacelike slice is one possible "now" for this observer. According to this slice, no dust particle has ever fallen in and reached the singularity; every such particle has a world-line that intersects the red slice, and therefore it's still on its way in.

The blue spacelike slice is another possible "now" for the same observer at the same time. According to this definition of "now," none of the dust particles exists anymore. (None of them intersect the blue slice.) Therefore they have all already hit the singularity.

If this was SR, then we could decide whether red or blue was the correct notion of simultaneity for the observer, based on the observer's state of motion. But in GR, this only works locally (which is why I made the red and blue slices coincide near E). There is no well-defined way of deciding whether red or blue is the correct way of extending this notion of simultaneity globally.

So the literal answer to the quoted part of the question is yes, but I think it should be clear that this doesn't establish whether infalling matter has "already" hit the singularity at some "now" for a distant observer.

Although it may seem strange that we can't say whether the singularity has "already" formed according to a distant observer, this is really just an inevitable result of the fact that the singularity is spacelike. The same thing happens in the case of a Schwarzschild spacetime, which we think of as a description of an eternal black hole, i.e., one that has always existed and always will. On the similar Penrose diagram for an eternal black hole, we can still draw a spacelike surface like the red one, representing a definition of "now" such that the singularity doesn't exist yet.

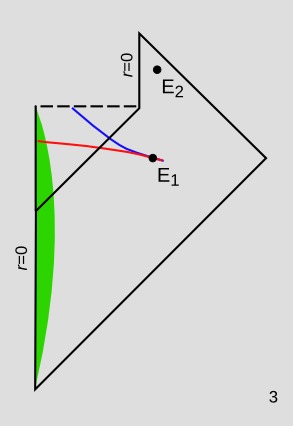
Figure 3 shows the situation if we take into account black hole evaporation. For the observer at event E$_1$, we still have spacelike surfaces like the blue one according to which the matter has "already" hit the singularity, and others like the red according to which it hasn't. However, suppose the observer lives long enough to be at event E$_2$. There is no spacelike surface through E$_2$ that intersects the cloud of infalling dust. Therefore the observer can infer at this time that all the infalling matter has hit the singularity. This makes sense, of course, because the observer has seen the Hawking radiation begin and eventually cease, meaning that the black hole no longer exists and its history is over.

Seahra, "An introduction to black holes," http://www.math.unb.ca/~seahra/resources/notes/black_holes.pdf

Answer 2

You are simply looking at it from an observer's viewpoint. Yes, looking from outside, matter tends to asymptotically approach but never reach the event horizon. If you were part of that matter spiraling into a black hole, there would be no problem reaching the horizon, crossing it, and going right down to the singularity. The event horizon is not a physical barrier. You could be free falling, and your time would not be infinitely dilated. So the answer is yes they can form easily in a finite time.

Answer 3

To begin with, there is a connected solution of 3+1 GR in which particles fall to the singularity in finite time. In particular, Gullstrand-Painleve coordinates do this. The big difference with Schwarzschild coordinates is that the speed of light depends on direction: light moves into a black hole faster than it moves out. See:
http://en.wikipedia.org/wiki/Gullstrand%E2%80%93Painlev%C3%A9_coordinates

For the formation of a black hole in these coordinates, see:

Phys.Rev.D79:101503,2009, J. Ziprick, G. Kunstatter, Spherically Symmetric Black Hole Formation in Painlevé-Gullstrand Coordinates
http://arxiv.org/abs/0812.0993

For the generalization of Gullstrand-Painleve coordinates to the rotating black hole, see the very readable paper that gives an intuitive explanation for what is going on, see:
Am.J.Phys.76:519-532,2008, Andrew J. S. Hamilton, Jason P. Lisle, The river model of black holes http://arxiv.org/abs/gr-qc/0411060

Note: that the above paper is peer reviewed and shows that yes indeed, particles falling past the event horizon travel with speeds greater than the 1 (in GP coordinates). In GR, the speeds of objects depend on the choice of coordinates. Consequently, this exceeding of the speed 1 is not equivalent to exceeding the speed of light. In GP coordinates, a light beam moving towards the singularity inside the event horizon also moves at speed greater than 1. Consequently, there is no violation of special relativity.

Answer 4

(this answer addresses the new question)

As a consequence of the singularity theorems, it is not only possible but (arguably) inevitable for singularities to form in a finite amount of "time" in a physically reasonable spacetime. The word "time" in this context means "proper time along a specific timelike geodesic". For example, if there is a trapped surface* in spacetime, then a singularity will appear within a finite amount of proper time (along a timelike geodesic) in the future of that surface; so, an observer sitting in a collapsing star will reach the singularity in finite time. Thus, the collapse of matter is one possible way to create a singularity "out of nothing". If your spacetime is globally hyperbolic and you foliate it by Cauchy surfaces you can say in a much more "universal" way that the singularity didn't exist at time [$t_{0}$] and came to exist at time [$t_{1}$].

I should point out that the singularities are a generic feature of physically reasonable spacetimes; take a look at the Hawking-Penrose theorem- it applies in very general situations.

Also, as the original question was about black holes and not singularities, I should advise you to make a clear distinction between the two concepts. Trapped surfaces form due to the condensation of matter (this is the famous Schoen-Yau theorem), and under a certain extra hypothesis, these surfaces will be hidden inside black holes. This extra hypothesis is the well-known (weak) Cosmic Censorship Conjecture (CCC). If it does not hold, gravitational collapse can create naked singularities, that is, singularities not "causally hidden" by the event horizon of a black hole. Much of what is known in general about black holes depend crucially on the CCC.

*A trapped surface is a two-dimensional spacelike compact surface such that the null geodesics departing from it are accelerating towards each other - mathematically, we say that the expansion of the congruence of future-directed null geodesics orthogonal to the surface is negative.

Answer 5

What astronomers claim to be black holes are objects that "progressively make themselves more similar to [a black hole] without actually reaching the stage of having an event horizon", as they reckon. That's assuming that GR is valid, since all such claims depend on GR's equations. Plenty of books on GR note that black holes are perhaps better named "frozen stars" from a distant observer's perspective.

Answer 6

Ben's answer did not show that black holes/event horizons can ever form from a distant observer's (e.g. our) perspective. In our frame of reference, strictly according to GR, no event horizon could ever have formed. For us, it doesn't matter what happens in the reference frame of collapsing matter of a star that went super nova, no matter how many Penrose diagrams one shows.

Answer 7

I get lost in the Jargon of most of the above replies. My original question may have been answered but I am not able to follow it.

I understand that to an observer falling into a Black Hole (BH), time seems just normal up until the state of Singularity is attained. However, my question is - to anyone far outside of the BH's Event Horizon (our observation place), there has not been enough of "our time" that has passed for the material inside the BH to have reached the singularity. The age of our Universe right now is fixed to 13.8 Billion Years. 13.8 Billion years inside a BH (now) has not been attained - right ?

If a BH formed (began) at 1 Billion Years (in our time), by now 12.8 Billion years has elapsed for us, but inside the BH only 1.00000001 Billion years may have elapes since the Big Bang.....the inside may be only a few seconds old !

I hope I am saying this correctly - 13.8 Billion years (the age of our Universe) has passed for us outside the BH, but much less elapsed time has passed (relative to the outside) inside any BH. Thus, what passes for the elapsed time inside the BH, is much, much less than what has passed outside the black hole. Yes ?

In our time outside the BH, the BH is still in the process of collapsing. If someone inside the BH were able to travel back to the outside, they would be young in a universe that had aged maybe thousands of times faster. In the big picture (the whole universe) the insides of BH's must be very young - i.e. less than 13.8 Billion years old and therefor still in the early stages of collapse.

Thank you for your patience.

Answer 8

I wouldn't know that any of the answers above has shown that, from an outsider's perspective, anything can ever reach the horizon, which was essentially the question of the OP. From an in-falling observer's point of view, there is no problem because kinematic time dilation and gravitational time contraction of the rest of the universe ("looking" in the rear view mirror of an in-falling observer's space ship, let's say) cancel each other out, exactly. But from an outsider's point of view, this is never the case.

Btw, that does not contradict any of the phenomena of black holes like jets and accretion disk-dependent events.

P.S. I suggest to not first think about e.g. light signals when we want to understand the nature of relativistic phenomena. The reality can always be "retro-engineered" from light signals, their arrival time and location and their redshifts, but that's very complicated and confusing to begin with. In order to understand things, we should first assume an instant information transfer.

Answer 9

It seems like, if there is a sufficient amount of matter localized during the Big Bang, then a Black Hole could form. But after that, a massive star, as seen by the rest of the universe could never shrink to the size of the event horizon.

In other words, a Black Hole would have to preexist in the universe to be an actual Black Hole.

Answer 10

I like your question. This model of a stellar black hole is created by freezing time as it is created from the inside out. It ends being solid frozen neutron matter with no singularity or event horizon, which is known as a black star. This is all relative to a remote location.

Using a program written in Excel it was found that a supernova remnant, with a mass between 1.44 and 2 solar masses, contracts down to a neutron star. The physical compressive forces during a supernova cause the remnant to contract down to neutron matter. Due to increased gravity, it continues to contract down to where the pressure, beginning at the center, supports the incoming mass where the contraction stops. During this contraction the decreasing gravitational potential also caused time to slow, but it was not enough to cause time to freeze.

For larger solar mass remnants, during the contraction, the increased gravitational potential is enough to causes time to relatively freeze starting at the center and stop the contraction before the pressure gets high enough to stop it, as it did in a neutron star. This also freezes the flow of information concerning the decrease in gravitational potential, thus; the frozen portions remain frozen and do not contract down any further. While this remnant is contracting down through a point where its’ radius is about 1.75 times the Schwarzschild radius, the gravitational potential meets the condition that causes time to first freeze at the center. By freeze I mean the rate of time flow becomes equal to the square root of a value that gets ever so close to zero; but, it never crosses over and becomes negative, where the rate of time flow would become imaginary. This means that in the position where time is getting close to being frozen, the coordinates do not reverse and become null light-like instead of time-like as it would on the inside of an event horizon or a radius that is frozen. The remaining shells of matter, which have not been frozen, continue to contract in and cause the gravitational potential to decrease which causes the next layer is freeze. Most of all the remaining unfrozen shells will continue to contract and freeze each successive layer almost all the way to the surface. The last bit of contracting matter will only have enough mass to cause time to significantly slow, but it will not quite freeze. If the freeze made its way to the surface, it would meet the condition of a black hole, having a Schwarzschild radius; but, it does not quite get there. What has been created is sometimes called a black star which has no event horizon or singularity and has matter through-out its volume.

The frequency of the light emitted by a black star is gravitationally red shifted and the rate of photon emission is also reduced. Because of this and the small size, a black star is almost black and extremely hard to see. These black stars are fairly uniform in density except near the surface where the density increases. The density is about the same as that of neutron stars with the larger black stars having a smaller density.

This model also discusses how the Chandrasekhar limit relates to black stars which in turn answers questions like:

1. Why is there such a large size gap between stellar black holes and super massive black holes?
2. Why are there no stellar black holes below 2 solar masses?
3. Why are there no supernova created stellar black holes above 15 solar masses?
4. Why does the smallest super massive black hole start at 50,000 solar masses?
5. Are super massive black holes made before or after the existence of first-generation stars in a galaxy?

These questions cannot be answered using black holes that have a singularity. They are answered by this model of the formation of black stars. The information produced by this model agrees with observation when available.

A link to my article, http://file.scirp.org/pdf/JHEPGC_2017072816470248.pdf was recently published in the pier reviewed Journal of High Energy Physics, Gravitation and Cosmology, in which I discuss this computer model that poses an alternative process to the accepted theory of the formation of a black hole. Due to a unique requirement of creating black holes by freezing time and space from the inside out, the conventional method of deriving results from general relativity could not be used. Instead I used the Newtonian model, while factoring in relativistic corrections derived from general relativity which includes the relative contraction of both time and space and the equivalence principal. This mathematical model, using Excel and Visual Basic, takes about a day to run.