9
$\begingroup$

In my physics book, it says that in the human ear, the sensation of loudness is approximately logarithmic. And that the relative sound intensity is directly proportional to a logarithmic ratio expressed as follows:

$ \beta = 10\log(\frac{I}{I_0}) $

So does that mean an average human wont perceive a , for example , sound of intensity $2*10^{-5} \frac{W}{m^2}$ to be a as twice as loud as a sound that has an intensity of $1*10^{-5}\frac{W}{m^2}$ , while they will perceive a sound that is $ 10 dB$ to be twice as loud as one that is $5dB$ ?

Improve this question
$\endgroup$
6
  • 2
    $\begingroup$ "while they will perceive a sound that is 10dB to be twice as loud as one that is 5dB ?" - no, that is not correct. A sound pressure level that is 10dB higher than another will be perceived as (roughly) twice as loud. $\endgroup$
    – Alfred Centauri
    Commented Sep 26, 2017 at 11:54
  • $\begingroup$ @AlfredCentauri how does that work though? $\endgroup$
    – Dahen
    Commented Sep 26, 2017 at 11:57
  • 3
    $\begingroup$ @AlfredCentauri: You are incorrect. The human hearing is logarithmic. The sound is perceived twice louder when the number of deciBells is doubled. You are confusing the subjective loudness with the physical volume that doubles at 6dB (not 10dB). However, doubling the physical volume does not double the subjective loudness. $\endgroup$
    – safesphere
    Commented Sep 26, 2017 at 15:36
  • 1
    $\begingroup$ @Dahen: Also note that intensity or physical volume depends on the squared voltage or current. This doubles the coefficient before the logarithm from 10 to 20. This is why you often hear that 6dB corresponds to the doubled signal (doubled as voltage): $10 Log((2 V)^2/V^2) \approx 6$ Compare to: $20 Log(2 V/V) \approx 6$ $\endgroup$
    – safesphere
    Commented Sep 26, 2017 at 15:47
  • 1
    $\begingroup$ @VictorStorm, I disagree - it is well known in the audio field that it takes 10 times the power to sound twice as loud. Further, increasing the power by a factor of 10 is a 10dB increase in power. I've provided a supporting reference in my answer. $\endgroup$
    – Alfred Centauri
    Commented Sep 26, 2017 at 21:53

3 Answers 3

Reset to default
9
$\begingroup$

From Victor Storm:

it is necessary to increase the power from a source by 10 times in order to double the loudness

This statement implies a linear 1:5 dependence of the subjective loudness on the source power.

This is not correct. Rather, the implied relation from @Alfred Centauri 's source is the following: loudness units (arbitrary) power 1 10 W 2 100 W 4 1000 W 8 10,000 W

and so on. This is neither a linear relation, nor a logarithmic one. It is a power law, $l_p= \frac{1}{2} P ^{\log_210}\approx\frac{1}{2}P^{0.301}$ , where $l_p$ is the perceived loudness and $P$ is the power in Watts.

Perceived loudness also varies with frequency:

enter image description here

(Courtesy of wikipedia: https://en.wikipedia.org/wiki/Sound_pressure)

An increase of 10 phons corresponds roughly to a doubling of perceived loudness (which has another unit, called the sone), so near the middle of the spectrum the relation given by @Alfred Centauri, that an increase in 10 dB corresponds to a doubling of loudness, is correct.

What about the supposed logarithmic behavior of hearing? Well, it simply appears that this is not necessarily correct. The alternate model proposed by the creator of the sone unit is Stephen's power law, which claims that perception of various senses occurs, well, as a power law. However, it is not clear to me whether a consensus exists on which of these models is better, either in general or for hearing specifically.

Improve this answer
$\endgroup$
8
  • $\begingroup$ So to answer my original question, I WILL perceive a 40 dB sound (around 3000 Hz so to not go the extremes) to be twice as loud as a 20dB one with the same frequency, right? The other sources I tried looking at also say what @AlfredCentauri is saying, but I'm not sure if those were talking about subjective human-perceived loudness or actual physical volume $\endgroup$
    – Dahen
    Commented Sep 27, 2017 at 6:20
  • 1
    $\begingroup$ Hi @Dahen, please see my edit which corrects a major initial misunderstanding I had. My current understanding is simply that sound perception does not scale in a logarithmic way, and that the use of decibels is basically just a historical artifact. $\endgroup$
    – Rococo
    Commented Sep 27, 2017 at 6:41
  • 1
    $\begingroup$ I see, so that means AlfredCantauri's answer is correct, right? Since the sone-to-dB table On the wiki page implies that. Alright, thanks for clearing up everything, much appreciated. $\endgroup$
    – Dahen
    Commented Sep 27, 2017 at 7:08
  • $\begingroup$ @Dahen Alfred's answer is a good rule of thumb for the middle of the auditory range (the book he links to emphasizes this). However, you can see from the graph that at 30 Hz, going from 80 to 90 decibels will actually increase the perceived sound by a factor of four, not a factor of two. $\endgroup$
    – Rococo
    Commented Sep 27, 2017 at 7:15
  • 1
    $\begingroup$ Yeah, I can see that. my question was intended to just cover the kind of "middle" range of the audible frequencies , in which case his answer is more correct than Victor's. Though honestly this makes me wonder, aside from making numbers look cleaner and more usable, what's the point of the decibel scale? $\endgroup$
    – Dahen
    Commented Sep 27, 2017 at 7:20
8
$\begingroup$

In a comment, Victor Storm writes:

@AlfredCentauri: You are incorrect. The human hearing is logarithmic. The sound is perceived twice louder when the number of deciBells is doubled. You are confusing the subjective loudness with the physical volume that doubles at 6dB (not 10dB). However, doubling the physical volume does not double the subjective loudness.

I respond with a quote from the book Recording Studio Design:

Our hearing perception tends to correspond to changes in sound pressure level, and it was stated earlier that a roughly 10 dB increase or decrease was needed in order to double or halve loudness. What therefore becomes apparent is that if a 10 dB increase causes a doubling of loudness, and that same 10 dB increase requires a ten times power increase (as explained in the last paragraph), the it is necessary to increase the power from a source by 10 times in order to double the loudness.

The emphasis in both quotes is mine.

From the (now removed) comment thread to this answer:

You are still wrong along with the non-scientific source you are quoting.

From the textbook The Hearing Sciences:

if you judge a 60 dB SPL pure tone to be twice as loud as a 50 dB tone, then you will judge a 70 dB SPL tone as twice as loud as a 60 dB SPL pure tone.

The quoted statement directly contradicts the claim that 'doubling the deciBells' is perceived twice louder.

Improve this answer
$\endgroup$
0
4
$\begingroup$

Unfortunately sometimes users post incorrect answers. For example @AlfredCentauri quotes incorrect statements from "the book of Recording Studio Design" without critically analyzing them to see that they have little to do with science or reality:

it is necessary to increase the power from a source by 10 times in order to double the loudness

As other have pointed out (thanks!) his statement implies a power law dependence of the subjective loudness on the source power. However, the human hearing (among other senses) is logarithmic. This phenomenon is known in psychophysics as the Fechner law that applies to all human senses:

Fechner's law states that the subjective sensation is proportional to the logarithm of the stimulus intensity. According to this law, human perceptions of sight and sound work as follows: Perceived loudness/brightness is proportional to logarithm of the actual intensity measured with an accurate nonhuman instrument.

$p = k\ln{\frac{S}{S_0}}$

The logarithmic scale means that multiplying the power by the same factor adds (as opposed to multiplying) the same amount to the subjective loudness. For example, every 10-times increase of the power adds the same amount to the loudness, but does not "double" it. The logarithmic scale has been well explained on this forum earlier by @dmckee and others:

Why is the decibel scale logarithmic?

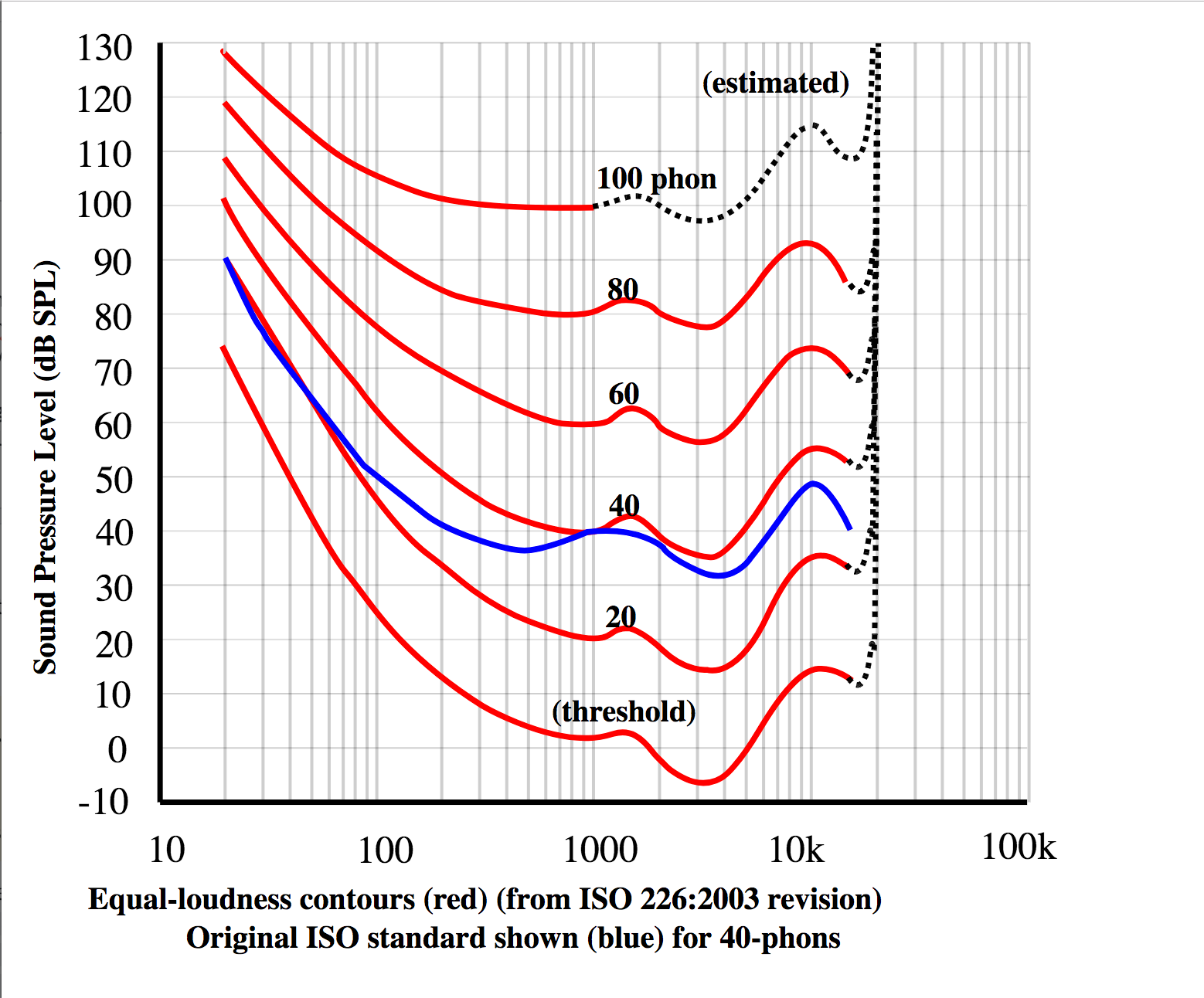
The human perception is logarithmic, because the sensitivity of the senses is variable (vision, hearing, etc.). When the sound becomes louder, the sensitivity of the ear is adjusted down (via the amount of the blood flow). As a result, the subjective volume increase is not linear, but logarithmic. The typical time of such an adjustment is approximately one second. For example, if you listen to a very loud sound and then to a very quiet sound, you would not hear the quiet sound, if the sounds are less than a second apart.

The dynamic range of the human hearing is about 130dB for sounds separated in time, but only a half of that (about 65dB) for simultaneous sounds. For this reason, the noise of an LP vinyl record (unless badly worn out) is not audible on the music background, but only between songs. The dynamic range of LPs is about 65-70dB designed to match the sensitivity of the human hearing.

Improve this answer
$\endgroup$
13
  • $\begingroup$ 1. That you need to increase power by a factor of 10 to double the loudness does not imply a linear 1:5 dependence, since it also implies that you need to increase power by a factor of 100 to increase loudness by a factor of 4, which is certainly not a linear relationship between power and loudness. 2. The very Wikipedia article on Fechner's law you link to states rather clearly that it is a poor approximation for human sound perception, so your claim that it "applies to all human senses" is undermined by your own reference. $\endgroup$
    – ACuriousMind ♦
    Commented Sep 27, 2017 at 8:48
  • $\begingroup$ So you would say that a jet engine at 100 feet away (140 decibels) is perceived as only around twice as loud as a normal conversation (60 decibels). This is patently ridiculous. $\endgroup$
    – Peter Shor
    Commented Sep 27, 2017 at 11:54
  • $\begingroup$ @PeterShor No I wouldn't and I didn't say this in my answer. You misinterpret the logarithmic law. $\endgroup$
    – safesphere
    Commented Sep 27, 2017 at 13:27
  • $\begingroup$ I think this follows from the statement: "For example, every 10-times increase of the power adds the same amount to the loudness, but does not 'double' it." So 0 decibels (the lower limit of perception) to 60 decibels increases the loudness by 6 units. And 60 decibels to 140 decibels increases the loudness by 8 units. Thus, according to a logarithmic scale, 140 decibels would be a little more than twice as loud as 60 decibels. Please explain the mistake in my reasoning. $\endgroup$
    – Peter Shor
    Commented Sep 27, 2017 at 15:01
  • 1
    $\begingroup$ @PeterShor I see what you mean, it is the same thing. These are dimensional values, so we must write it this way: $\frac{L_1}{L_0}=\log_B{\frac{P_1}{P_0}}$ and $\frac{L_2}{L_0}=\log_B{\frac{P_2}{P_0}}$ then $\frac{L_2}{L_0}-\frac{L_1}{L_0}=\log_B{\frac{P_2}{P_0}}-\log_B{\frac{P_1}{P_0}}$ or $L_2-L_1=L_0\log_B{\frac{P_2}{P_1}}$ What you mean by subtraction is the same as what I wrote in my answer, "multiplying the power by the same factor adds the same amount to the subjective loudness." I believe we are in agreement :) $\endgroup$
    – safesphere
    Commented Sep 29, 2017 at 4:07

Not the answer you're looking for? Browse other questions tagged or ask your own question.