0
$\begingroup$

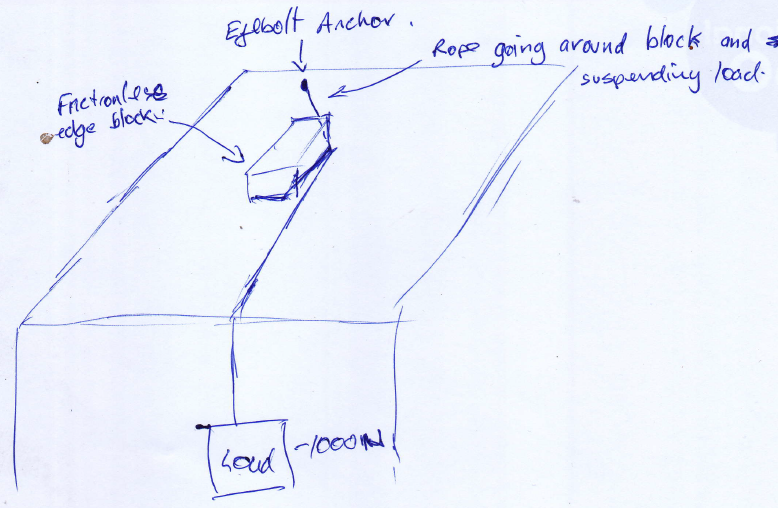
Consider the diagram below. All corners are assumed to have no friction. Is it true that the tension throughout the rope is equal in all sections? The section over the edge of the building, the section between the edge of the building and the back right corner of the block where the rope turns around it, and finally the section between the back right corner of the block and the eyebolt anchor point.

Maybe another way I could reframe my question is, can the angles around these edges influence the tension in the different sections? For example, the angle between the rope and the block is not the same on the load-side as the angle between the rope and the block on the anchor-side. Does that matter?

enter image description here

Improve this question
$\endgroup$
11
  • 1
    $\begingroup$ If you don't consider friction edge angle don't affect tension. It will be uniform throughout its length. $\endgroup$
    – Fire
    Commented Jul 14 at 2:49
  • $\begingroup$ Thankyou for your help @Fire $\endgroup$
    – Bucephalus
    Commented Jul 14 at 3:15
  • 1
    $\begingroup$ As long as all surfaces are considered frictionless and the rope is considered non extensible, the tension would theoretically be constant throughout. IMO this is an example of “pushing the envelope”. Normally, at least the surfaces are rounded. $\endgroup$
    – Bob D
    Commented Jul 14 at 6:50
  • $\begingroup$ All corners are assumed to have no friction. This is quite an assumption! $\endgroup$
    – Farcher
    Commented Jul 14 at 10:57
  • 1
    $\begingroup$ @Bucephalus The direction of a normal to an ideal corner is an interesting concept. With you two bits of rope, either side of the corner, you can always choose the normal to be one which bisects the angle. $\endgroup$
    – Farcher
    Commented Jul 15 at 12:37

1 Answer 1

Reset to default
1
$\begingroup$

I would consider each bent part a point of interest. Starting at the last bend before the hanging mass, let's fix the rope on the edge and calculate the tension for this segment. Call it $|T_2|=mg$.

Next, the kink at the block. Pretend the rope is fixed at this point. This time, let's simplify by replacing the edge and hanging mass with a constant force of $T_2$. See where this is going? This tension is just $T_1 = T_2$.

The rope is not stretching, sliding, or moving, so the presence of friction does not matter. This is an essential aspect of your question. In the static case, Any corner perfectly steers the rope's tension from the start to the hanging mass. If you had a knob that increased the static friction of your system, and you turned it all the way up so that it takes more than the tension to make the string start moving, then you effectively have three separate segments of string, each held at the original tension.

Start letting things move, and this becomes a totally different question.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for your response @ABetheGammow $\endgroup$
    – Bucephalus
    Commented Jul 15 at 5:12

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.