Can I get burned with warm air?

Question

If temperature is the "average" result of measuring a mix of hot and cold air particles, then I can be in a room of some warm temperature but being hit with both extremely hot particles and extremely cold particles at the same time? So even though the overall temperature is totally safe and warm, I can still get (heat/cold) burn together?

Edited:

I guess my original question was not about diffusion nor what considered as "burn" so I want to clarify. Consider this an experiment, there is a room built to constantly supplies some extremely hot air from one vent and some extremely cold air from another vent (not directly blowing onto the person). Walking into this room you feel warm as the hot and cold air average out to warm temperature. Comparing to another warm temperature normal room without all these, would a person staying inside the experiment room experiences more damage on skin than a person in the normal room? My thinking was: yes, because of the skin being constantly hit by both extremely high/low energy air particles, i.e. double the damage than the normal room; or no, as the constant heat transferred onto the skin is averaged out by the heat taken away at the same rate, so its the same as the normal room.

Answer 1

Not really. In a more precise language, what you are describing is that temperature is an ensemble property of particles, and individual particles can store more energy (in case of a gas: mostly be faster) or less than the average. However, this applies to cells, heat sensors or anything else that would “feel” the heat too. Those are still sufficiently big that they are primarily affected by temperature as an ensemble property of their molecules.

For example, a single fast air molecule hitting a cell usually will slightly heat it up as the impact dissipates over the many molecules of the cell. Heating up the entire cell to the extent that it suffers heat damage would take so many fast molecules to hit it in a short time that such an event is extremely unlikely. The same applies to a single ultra-fast molecule holding all the energy. This is rather something you might get from radioactivity and similar.

Now, a fast air molecule that hits a protein (or similar) might disintegrate it, but that’s not a critical damage to the cell as this happens all the time and proteins get replenished by the cell. (This is also why the critical molecules such as DNA need to have a considerable heat tolerance to keep the incidence of cancer at a tolerable level.)

Consider this an experiment, there is a room built to constantly supplies some extremely hot air from one vent and some extremely cold air from another vent (not directly blowing onto the person). Walking into this room you feel warm as the hot and cold air average out to warm temperature.

The coldest temperature at which you have air is roughly −190 °C. If we assume that the heat capacity of air does not change much with temperature, that your warm temperature is 30 °C, and you supply hot and cold air in equal rates, your hot air would have to be 250 °C. That’s a temperature that humans can withstand for a short time (just put your hand into a heated oven).

Thus, we do not even need to think about whether the velocity distribution of particles equilibrates to the Maxwell–Boltzmann distribution faster than it reaches your skin.

Answer 2

The kind of room you are thinking about, with a mix of extremely energetic and extremely slow moving particles, averaging out to a warm temperature room, will very very quickly spread apart the energies of its particles so that it returns to the distribution of energies in a "normal" warm temperature room. So really, unless this air you are speaking of was just created by mixing very hot and very cold air a fraction of a second ago, all air you come into contact with will have pretty much the same exact distribution of hot, medium, and cold particles as any other bit of air at the same temperature, the Maxwell–Boltzmann distribution, and you are unlikely to get hurt by just the fast moving particles unless you were right next to the constant stream of newly mixed "extreme" air.

Answer 3

Others have answered that when the hot and cold gases are in contact, they redistribute their energies very fast, so you won't be able to feel their temperatures separately, but only as a temperature of the mixture. But how fast? Would we feel anything, in the very short time even before the gases have redistributed their energies?

To answer that, we need to understand how fast this heating/cooling happens, compared to this redistribution of energies. Let's look at some numbers.

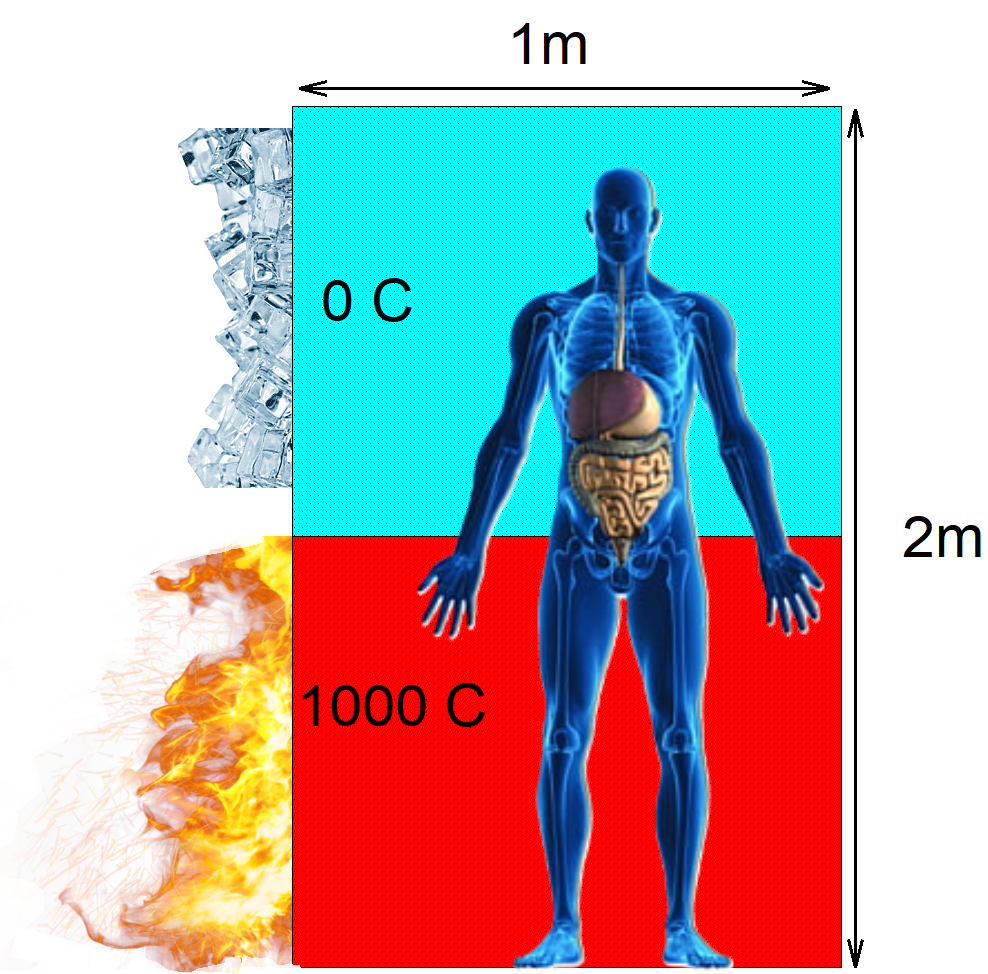
You need $800-1000^\circ\:C$ to cremate a human body. But you cannot go higher than $1530^\circ\:C$, since nitrogen in the air could ignite. Consider a box of volume 2 m^3 separated by an adiabatic partition. On one side of the partition, we heat the air to 1000 C, and on the other side, we cool the air to 0 C. Let the partition be removed at t=0.

First, let's get an idea of how much time it takes for the hot air to diffuse to the cold air side. Would it be slow, like smoke entering a room, or would it be instantaneous, like an explosion?

For an air parcel sitting in the hot compartment, the momentum equation is given by:

$$\rho\dfrac{du}{dt}=\dfrac{dP}{dx}$$ where $\rho $ is the density of air, $u$ is horizontal velocity, $P$ is the pressure.

Here only the pressure gradient force is written on the RHS since all other terms are negligible. For air, ideal gas law $PV=nRT$, $\rho=\:1 kg/m^3$, assuming one mole of gas and unit volume, we obtain:

\begin{align*} \dfrac{du}{dt}&=\dfrac{1}{\rho}\dfrac{dP}{dx}\\ &=\dfrac{1}{\rho}\dfrac{P_1-P_2}{dx}\\ &=\dfrac{1}{\rho}nR\dfrac{T_1-T_2}{V\:dx}\\ &=\dfrac{1}{1\:kg\:m^{-3}}1\:(mol)\:8.314\:(J\:mol^{-1}\:K^{-1})\dfrac{1000\:K}{1\:(m^3)\:1\:(m)}\\ &=\:8314 ms^{-2} \end{align*}

With this, the time the parcel takes to reach the other end of the 2m box starting from rest is $\bf{\sqrt{2*2\:(m)/8314\:(m/s^2)}=0.02\:seconds}$. That's more like an explosion. For reference, the blink of an eye takes 0.1 seconds.

Assuming that the order of magnitude of the mixing of energies and this spatial mixing is the same, the time scale of mixing is one-hundredth of a second.

Now, how much energy does it take for the $1000^\circ\: C$ air to warm your body by 1 degree?

\begin{align*} Q&=mc\Delta T\\ &=70\:(kg)\:4186\:(Jkg^{-1}K^{-1}) 1\:(K)\\ &\approx 300\:kJ \end{align*}

The equation for convective heat transfer is given by:

\begin{align*} \dot{Q}&=hA\Delta T\\ &\approx 20\:(W/m^2K)\:2\:(m^2)\:1000\:(K)\\ &\approx 40\:kW \end{align*}

Time it takes to heat the human by $\bf{1^\circ C=300/40\:s=7.5\:seconds}$

It takes $7.5$ seconds for the air to heat the body by just 1 degree, but just $0.02$ seconds for the air from the bottom compartment to reach the other side of the box. So forget about heating $1000^\circ\:C$, the mixing is at least 2 orders of magnitude faster.

The minimum temperature difference sensible for humans is 0.05 K. Plugging in this instead of 1 in the equation gives 0.38 seconds of exposure, but still mixing would have happened much before that.

TLDR: No, you won't get burned. You won't even feel the temperature change. You won't feel hot and cold gases separately, but only the temperature of the mixed gas.

---

_{Assumptions: Here only the thermal effects are considered. Effects like mutation happening to DNA due to molecules banging on it are neglected. The human body is at 37 degrees Celsius. m is the mass of the body, c is the specific heat capacity of the body, h is the convective heat transfer coefficient of the surrounding fluid and A is the surface area of the body. For an average human, $m=70\:kg$ and surface area is $\approx 2m^2$, $c=4186\:kJkg^{-1}K^{-1}$ since body is mostly water. $h$ for air is $5-40\:W/m^2 K$, lets assume $20\:W/m^2 K$. Since $T_{surrounding}>>\Delta T_{body}$, the heat transfer is assumed to be linear.}

Answer 4

An example of this is nonthermal plasma, also commonly called cold plasma. In a cold plasma the electrons of a gas are stripped away from their nuclei using a strong electromagnetic field, but the remaining ions are not given the chance to thermalize. This means that the electrons are at a much higher temperature than the ions. Because ions are so heavy, they deliver the bulk of the thermal energy when something touches it. Therefore, it is possible to create to create a plasma that is cold to the touch even when the electrons could be at $20\,000K$. See for example this article from Hackaday that describes a video from the plasma channel.

Answer 5

By systematically recording the distribution of free paths (distances traveled by molecules between genuine collisions), the mean free path, λ, of air is determined as 38.5 ± 1 nm at 300 K and 1 atm.

That means our energetic particle will have collided with a million other air molecules by the time it has travelled 38 mm and that won't be in a straight line. Each time it collides with less energetic molecules, it loses some of its momentum and kinetic energy. The chances of an energetic molecule traveling even 1 mm in a straight line without without losing energy is almost zero.

This is at normal room temperature and pressure. If we made the room air tight and reduced the pressure to almost a vacuum, with just a few molecules, then there is a possibility of being impacted by an energetic molecule, but the damage would be very localised and the impact energy very quickly distributed to nearby skin molecules and the damage would probably be unnoticeable. The top layer of our skin is continuously shed and replaced, so such localised damage is not permanent.

But what about the bulk properties of air coming from fans as a comoving block of hot or cold air? You said the fans are not directed directly at the person, but we could arrange the fans to create comoving inspiralling streams of hot and cold air moving parallel with each other. At the interface of the two streams there will still be collisions and the collisions not only reduce the difference in kinetic energy of individual molecules but also rebound in random directions increasing the mixing between streams. the nature of a vortex is that it reaches an equilibrium where the entire circulating volume has a common angular velocity with local tangential velocity lower at the centre like the eye of a hurricane so at the centre of the room the local tangential velocity is relativity low further increasing the time for mixing and averaging of the molecular kinetic energies and it is unlikely even in this contrived set up that any significant damage would be done to person at the temperature differences you mention.

Answer 6

A "mix" of hot and cold is not the same as warm. The thing that "burns" is not the temperature, but the cumulative effect of a huge number of reactions. Were all of the reactions linear, hot plus cold may be the same as warm. However, in practice chemical reactions are not linear. Most reactions we are interested in for "burning" have an activation energy. The collisions need to put enough energy into the molecules to cause the reaction to occur. Low energy collisions cause little-to-no effects, while high energy collisions exceed the activation energy and cause something to occur.

Temperature is a measure of the average energy of these collisions. For virtually all real interactions, this range of energies is rather narrow, because you have a large number of interacting particles (central limit theorem). In these environments, temperature is a good metric with which to predict burning. However, in the exotic environment you describe temperature no longer adequately describes the quantity of high energy interactions that will occur.

Ahavens had a "link only" answer to your question. It was down voted because link only answers are not encouraged on stack exchange, but they brought up an excellent example of one such exotic situation where "temperature" isn't a valid way to measure the interactions that occur. In the story of Anatoli Burokski that they linked, such an unusual mix of energies did occur. In that environment, room temperature air and exceedingly high energy particles (typically thought of as "high temperature") were mixed when an accident exposed Burkoski to a beam of protons. The resulting damage was not associated with the "average" of the two, but was more associated with the high energy of the proton beam, which exceeded the activation energy of many critical reactions in the human body.

Answer 7

Unless the air is moving at high speed in contact with your body (which according to your parameters it is not) you will not get burned. Air is a very poor conductor of heat and you will be exposed to air of varying temperatures on each part of your body, sometimes hot and sometimes cold.
Here is a video of a person walking over hot coals at 400C without getting burned. This is because the coals are a very poor conductor of heat.walking on hot coals
In cryotherapy people expose their naked bodies to temperatures as low as -200F for several minutes - supposed to be healthy! cryotherapy

If however you are in a fast moving stream of hot air you will get burned, this is because the fast moving air greatly increases the rate of heat transfer. This is why when freezing a product blast freezers are used. These move the cold air at a very high speed which reduces the time for a pallet of product to freeze from days to hours. Individual products on a conveyor belt will freeze in minutes.

Answer 8

Yes it is possible and has happened (if you consider a high energy proton beam from a particle accelerator "air"). https://en.wikipedia.org/wiki/Anatoli_Bugorski