It follows from the theorem of kinetic energy, namely
$$\dot{K} = P^{tot} = P^{ext} + P^{int} \ ,$$
i.e. the time derivative of the kinetic energy of a closed system equals the total power (sum of internal and external) of forces and moments acting on it.
In the complete system, there is no action with net power, neither internal or external (see below).
Thus, the kinetic energy is constant. The only thing moving is the disk, so kinetic energy only has its contribution. Thus
$$K^{disk} = \frac{1}{2} m |\mathbf{v}_P|^2 = \text{const.}$$
Forces in the system has no power - Proof.
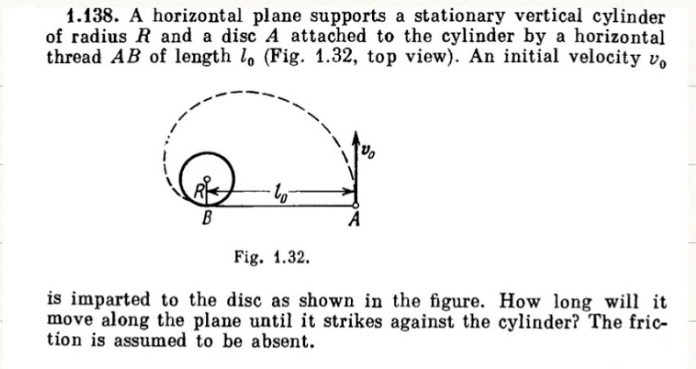
The wire is assumed to be inexstensible and massless. This implies that it transfer traction $T$ along its direction.
The only force acting on the disk is the rope tension, $T$, that is always orthogonal to the trajectory, and thus it has no power no the disk, and thus the disk keeps constant kinetic energy.
Let's prove it, with reference at the following figure.
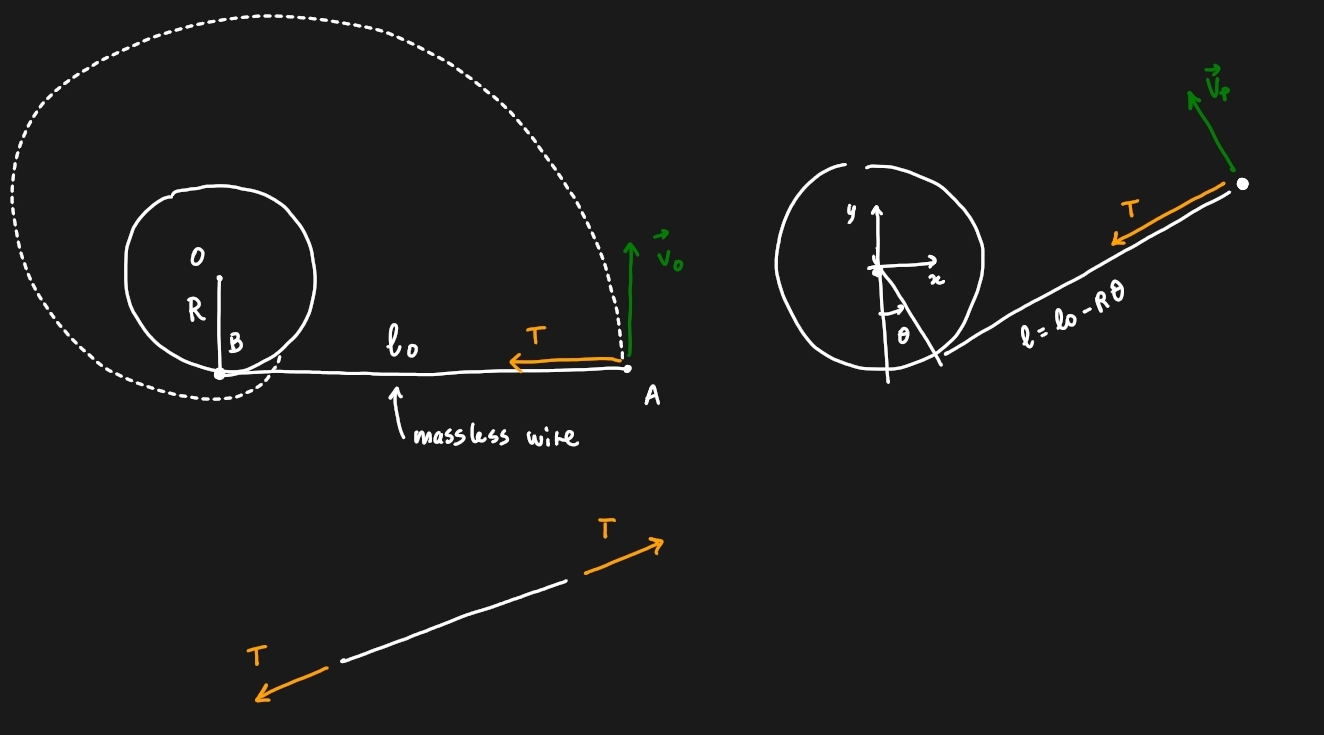
Position of the disk is $$\mathbf{r}_P(t) = \mathbf{\hat{x}}(R\sin\theta+(\ell_0-R\theta)\cos\theta) + \mathbf{\hat{y}}(-R\cos\theta+(\ell_0-R\theta)\sin\theta)$$
Its velocity is $$\mathbf{v}_P(t) = -\mathbf{\hat{x}}(\ell-R\theta)\dot{\theta}\sin\theta + \mathbf{\hat{y}}(\ell-R\theta)\dot{\theta}\cos\theta$$
Tension: $$\mathbf{T} = -T (\mathbf{\hat{x}}\cos\theta + \mathbf{\hat{y}}\sin\theta)$$