I am confused by the physical significance of image charges. A similar question has been asked about the fact that image charges add up to the total charge in the conductor, which I think is reasonable, but an example I came across seems to violate this rule.
In the well-known problem of determining the capacitance of two conducting spheres, taken from William Smythe's Static and Dynamic Electricity, the following ingenious application of the method of images can be used:
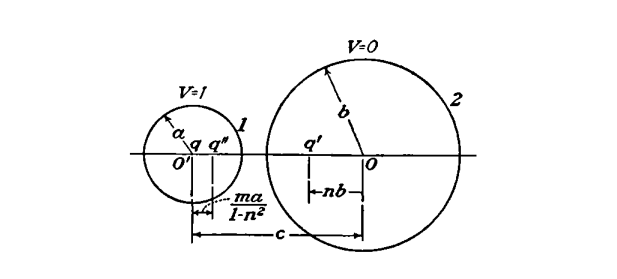
Consider two conducting spheres of radius $a$ (sphere $1$) and $b$ (sphere $2$) whose centres are a distance $c$ apart. To determine the capacitance, we raise sphere $1$ to potential $V_1$ by placing a charge
$$Q^{(1)}_1=4\pi\epsilon_0aV_1. $$
at its centre $O'$ and keep sphere $2$ untouched. Use the superscript to signify in which sphere the images are located, while the subscripts signify the $n$-th image charge in the sequence.
The charge in sphere $1$ induces charges in sphere $2$, which can be accounted for by placing an image charge $Q^{(2)}_1=-\frac{b Q^{(1)}_1}{c}$ a distance $x^{(2)}_1=\frac{b^2}{c}$ from $O$. The same goes for sphere $1$ with $Q^{(1)}_2=-\frac{a}{c}Q^{(2)}_1$ at $x^{(1)}_2=\frac{a^2}{c-x^{(2)}_1}$ from $O'$, and so on... This gives the recurrence relation
$$Q^{(1)}_n = -\frac{a}{c}Q^{(2)}_{n-1}, \quad x^{(1)}_n = \frac{a^2}{c-x^{(2)}_{n-1}}, $$
$$ Q^{(2)}_n = -\frac{b}{c}Q^{(1)}_{n-1}, \quad x^{(2)}_n = \frac{b^2}{c-x^{(1)}_{n-1}}. $$
Solving for $Q^{(1)}_n$ we get the difference equation
$$ \frac{1}{Q^{(1)}_{n+1}} + \frac{1}{Q^{(1)}_{n-1}} - \frac{c^2-a^2-b^2}{ab}\frac{1}{Q^{(1)}_n} = 0, $$
hence
$$ Q^{(1)}_n = \frac{4\pi\epsilon_0 ab}{b\sinh n\alpha + a\sinh (n-1)\alpha}, \quad \cosh\alpha = \frac{c^2-a^2-b^2}{2ab}. $$
The self-capacitance can be found by noting that $c_{11} = Q^{(1)}/V_1$, that is,
$$ c_{11} = \frac{\sum^\infty_{n=0} Q^{(1)}_n }{V_{1}} = 4\pi\epsilon_0 ab\sinh\alpha\sum^\infty_{n=1}\left[b\sinh n\alpha + a\sinh (n-1)\alpha\right]^{-1}. $$
My question is that the "total charge" $Q^{(1)}=\sum^\infty_{n=0} Q^{(1)}_n$ in the capacitor after applying the method of images infinitely many times is not what we started with, which was $Q^{(1)}_1$. Is the interpretation that image charges should add up to the total charge on the conductor wrong?