Parallel Axis Theorem Derivation

Question

My professor was deriving the parallel axis theorem wherein he took the Center of mass of an object as some point O and was calculating the moment of inertia about an axis through point P located at a distance d from O. I consider a point i with infinitesimal dimensions located at a distance r from P ( given by vector $\vec r$ ) and distance $r_i$ from O ( given by vector $\vec r_i$ ).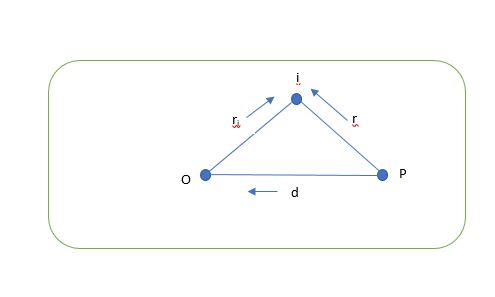

\begin{align} I_p=\sum_i m_i r^2 &= \sum_i m_i (\vec d+ \vec r_i)(\vec d+ \vec r_i) \\ &= d^2\sum_i m_i + \sum_i m_i r_i^2 + 2d \sum_i m_i r_i= d^2\sum_i m_i + I_{cm} + 2\vec d \sum_i m_i \vec r_i \end{align}

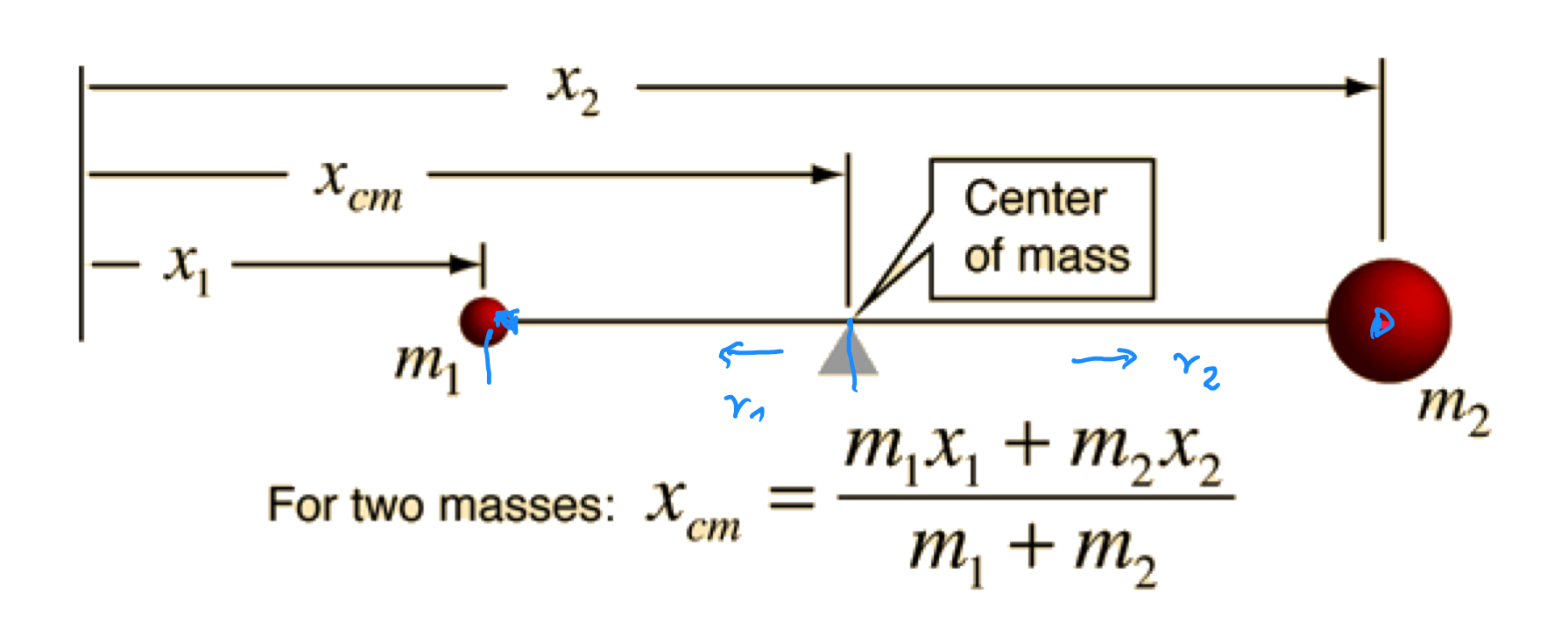
According to my professor $\sum_i m_i \vec r_i$ vanishes because $\sum_i m_i \vec r_i$ can be written as $(\frac{\sum_i m_i \vec r_i}{M}) \times M$ and $\frac{\sum_i m_i \vec r_i}{M} =0 $ because this is the center of mass in a co-ordinate system with center of mass as the origin and hence is zero I do not understand this. Maybe because I do not have complete grip over the concept of center of mass.

Answer 1

So, to first give you an idea about what the centre of mass (COM) is and why it is useful to introduce it, imagine we have some object that is performing a superposition of an unaccelerated drift motion along a straight line and a circular rotation with small radius around some comoving centre (see the figure with drift motion to the right). This could be for example a planet circling a central star which is moving unaccelerated in space.

If we ask about its moment of inertia around some axis, it is intuitively clear, that we should not care about the moment of inertia with respect to some fixed axis since this will change with time as the object drifts through space. Instead, we know that if we have an axis comoving with the object, we can separate the contribution coming from the drift motion and a contribution constant in time coming from the rotation around the centre of rotation in the comoving system.

The COM $\vec{R}=\frac{1}{M}\sum_i m_i \vec{r_i}$ is then the formal tool we use for this separation. It gives us the contribution of the drift motion, which we can set to zero by switching to the comoving coordinates frame $\vec{r}_i'=\vec{r}_i-\vec{R}$ (physics should be the same in all inertial frames).

Thus, when proofing the parallel axis theorem, we can always assume to be in the $\vec{r}'$ system where $\vec{R'}=0$ since all interesting physics is captured by the contribution from the comoving system. Hope the answer helps

Answer 2

It is sometimes easier to look at a simple system when trying to understand what to do.

Centre of mass at $O$, with mass $2m$ at $Y$ and mass $m$ at $X$.

$I_{\rm O}=2m\,y^2 + m\,x^2$

$I_{\rm P} = 2m(y+d)^2+m(d-x)^2$

Simplifying and rearranging gives,

$I_{\rm P} = \underbrace{d^2(m + 2 m)}_{\Large d^2\, \Sigma m_{\rm i}} + \underbrace{2m\,y^2 + m\,x^2}_{\Large I_{\rm O}} + \underbrace{2d(2m\,y-m\,x)}_{\Large2d\,\Sigma m_{\rm i}r_{\rm i}}$

and it is the last term that have asked about.

You can see by inspection that if $O$ is the centre of mass of the system then $\Sigma m_{\rm i} \times 0 = \Sigma m_{\rm i} \,x_{\rm i} = m\,x - 2m\, y$ which makes that last term zero.

Answer 3

http://hyperphysics.phy-astr.gsu.edu/hbase/cm.html

for two masses the center of mass position is:

$$x_{cm}=\frac{m_1\,x_1+m_2\,x_2}{m_1+m_2}\tag 1$$

with $$ x_1=x_{cm}+r_1\quad ,x_2=x_{cm}+r_2$$

you obtain from equation (1)

$$0\,(x_{cm})=\frac{m_1\,r_1+m_2\,r_2}{m_1+m_2}=0$$

or in general case $~\sum_i\,m_i\,r_i=0$

Answer 4

The centre of mass is defined such that the sum of mass moments about it is zero. Recall how the CoM was defined: $$ M\vec{r}_{cm} = \sum_{all}m_i\vec{r}_i $$ M can be written as $\sum_{all}m_i$. The above equation can then be written as: $$ \sum_{all}m_i\vec{r}_i = \vec{r}_{cm}\sum_{all}m_i = \sum_{all}m_i \vec{r}_{cm} $$

Transposing:

$$ \sum_{all}m_i\vec r = 0 $$ where r is distance vector from the CoM.