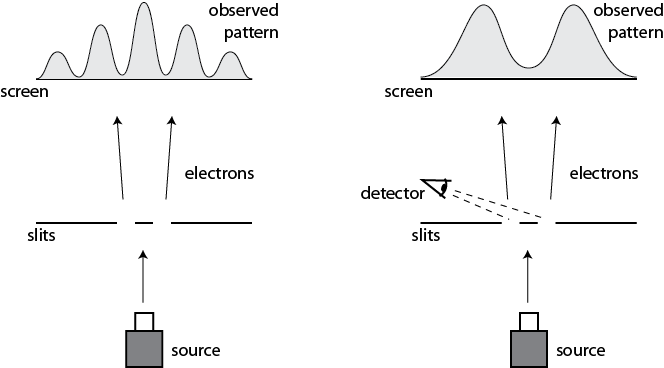
I agree that the Double Slit (DS) with photons is conceptually similar to the DS with an electron (or other quantum particle types). However, there are experimental & theoretical variations on the DS with photons that cannot be easily performed for other particle types. And this gives us more insight. Let's take the case of photons one at a time to build up a pattern, and vary our knowledge of "which slit" information. We CAN do this without affecting the total number of photons which will hit the screen.
As you know, the general rule is that if you could NOT know which slit the photon goes through, there will be interference. If you could know which slit the photon goes through, there will NOT be interference. It does not matter if you actually know the which slit information, it is enough that you could know it. And it is possible to vary continuously between these two extremes and get corresponding variation in the pattern (i.e. a mixture of interference and no interference).
The method we consider is when we place polarizers over each slit. a) If the polarizers are aligned parallel, there WILL be interference. b) If the polarizers are aligned orthogonal (crossed, perpendicular), there will NOT be interference. Parallel: it is NOT possible to extract which slit information. Orthogonal: it IS possible to extract which slit information. Either way, 50% of the photons will be blocked by the polarizers. So the total light detected remains constant.
Also, you may choose to look at the b) scenario as follows: there can be no interference between the slits if they are sending through the "portion" of the possible "paths" (for lack of a better description - insert your preferred description here) that are themselves now orthogonal. By definition pretty much, these cannot self interfere. Of course they can self interfere in the a) scenario.
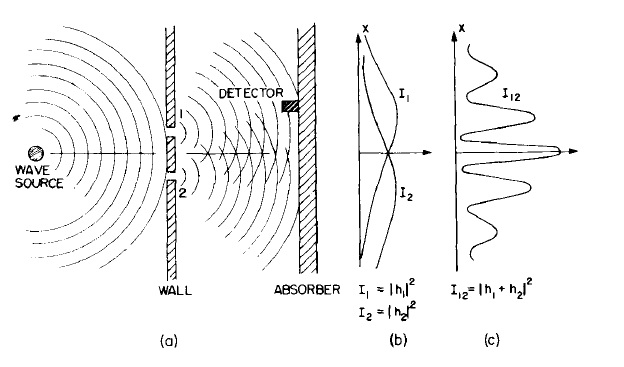
Note that there is no mention of "collapse" or "localization". There is no need to refer to point particles or similar. So yes with a "*"; the answer is that in the DS with photons (or whatever), detection will occur much greater in some places when there is self-interference ("unobserved") than when there is no such interference ("observed"). As pointed out by @benrg, the diffraction that occurs when there is no self-interference places some light everywhere. So it might be more accurate to say: When observed, we see light in places we do not see when unobserved. You can compare Figures 8 and 9 in the experimental reference below to see this is the case. References:
Theory: Polarized Light https://books.google.com/books?id=w6PMBQAAQBAJ&pg=PA271&lpg=PA271&dq=double+slit+interference+polarizers+Fresnel&source=bl&ots=MumGUCvweh&sig=7wit8bq5xXkunYoHpnbB3hL3YXw&hl=en&sa=X&ved=0CB0Q6AEwAGoVChMI-tm_ueGNyQIVBigeCh1SLwwt#v=onepage&q=double%20slit%20interference%20polarizers%20Fresnel&f=false
Experiment: Young’s double-slit experiment with single photons and quantum
eraser https://sciencedemonstrations.fas.harvard.edu/files/science-demonstrations/files/single_photon_paper.pdf