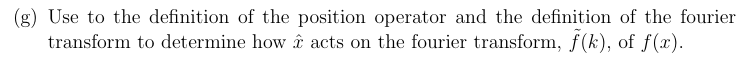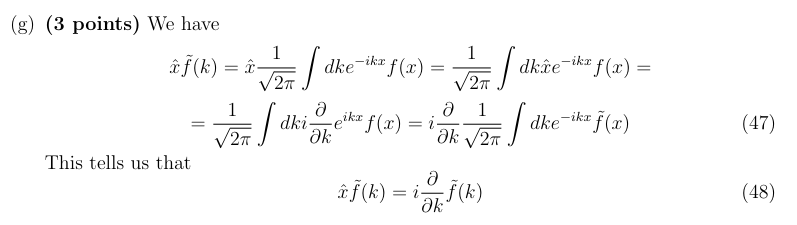Doubt in position operator acting on fourier transform, $\tilde{f}(k)$ of $f(x)$

Question

I was solving a problem from the problem sets provided by MIT OCW. Here's the problem set.

I was stuck in the problem 2(g)

So, I looked at the solution to the problem and couldn't understand it.

Link to the entire solution set.

Things which I didn't get from the solution provided:

1. The Fourier transform should be: $$\tilde{f}(k) = \frac{1}{\sqrt{2\pi}}\int dx e^{-ikx}f(x)$$ There should be $dx$ but in the solution it is written $dk$.

2. If, say, there is a typo, and it should be $dx$, then we can't take the position operator inside the integral, now how to approach the problem.

3. And if it isn't a typo ( I need to learn more!!!), how were the following calculations obtained. $$\hat{x}e^{-ikx} = i\frac{\partial}{\partial k}e^{ikx}$$ and then in the next step there is again a minus sign in the exponential.

Answer 1

I'll try my best to answer each of your questions in the following:

1. Yes, it should be $dx$ not $dk$ (it's a typo)
2. No, you can still take the position operator inside the integral. This is probably a bit clearer if we re-write the steps as follows \begin{align} \hat x \tilde f(k) &= \hat x \int dx' e^{-ik x'} f(x')\\ &= \int dx' \hat x e^{-ik x'} f(x')\\ &= \int dx' x' e^{-ik x'} f(x') \end{align}
3. The missing minus sign in the exponential is a typo. The solutions are using the following: $$i \frac{\partial}{\partial k}e^{-ikx} = i (-ix) e^{-ikx} = x e^{-ikx} = \hat x e^{-ikx} $$

Looks like the solutions just have a couple of typos. Otherwise you seem fine.

---

Throughout this answer I have just accepted the definition \begin{equation} \hat{x} f(x) = x f(x), \end{equation} This definition is a little loose, see my answer to another question here for more details.