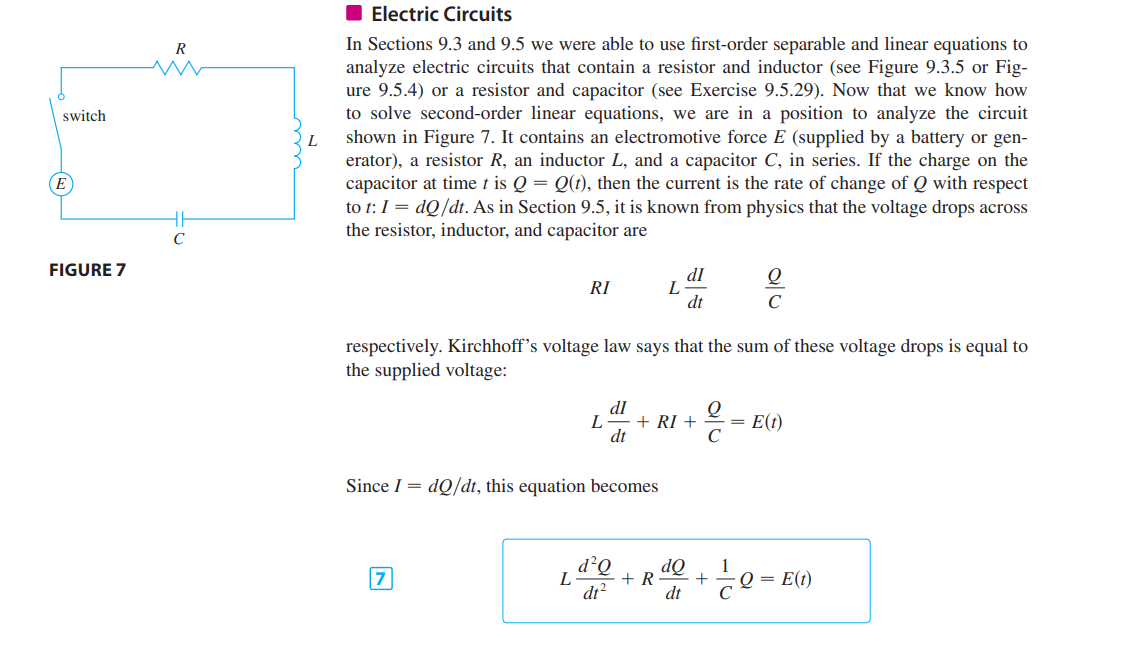
You can use the so-called KVL (the Kirchhoff Voltage Law) in AC circuits.
KVL is always valid, for trivial reason: it works intentionally with electric potential. Potential is always well-defined based on the conservative part of total electric field, and sum of its drops in closed path is always zero, whatever the induced field is.
It is true that KVL is sometimes not directly applicable (while still being true); this happens when potential drops on various elements cannot be easily expressed, such as when there is external induced electric field (due to other bodies such as moving magnets or other circuits) acting on current in the circuit. But this is not the case here. But it would be the case in transformer, for example. Then, one must go to the original Kirchhoff's second circuital law (the original formulation of KVL), which does not use potential drops, but complete loop EMFs.
So back to your example, KVL says that sum of all potential drops in a closed path equals zero. Since there is no external source of EMF (such as moving magnets or other circuit) in your question, KVL is applicable. Drop of potential on perfect inductor is $LdI/dt$ (follows from the Faraday law), drop on resistor is $RI$ (follows from absence of non-conservative field at the resistor), drop on capacitor is $Q/C$ and drop on the power source is $-E$ (the minus is because under normal circumstances, potential drop on a battery acts against its EMF). All this leads to the correct equation
$$
L\frac{dI}{dt} + RI + \frac{Q}{C} - E = 0.
$$
which your textbook gives.
The same result can be obtained starting from the Faraday law and then using the generalized Ohm's law (which is just another way of stating the Kirchhoff's second circuital law) to find that integral of total electric field over the resistor is $RI$. This method is arguably easier to explain and justify.
The KVL method requires some explaining about when it is applicable (no external emfs acting on the circuit) and that it works with potential based on the conservative part of field only. But it is easier and more tidy to use this method in practice when writing down equations for complicated circuits.