How should be the charges get distributed in this question?

Question

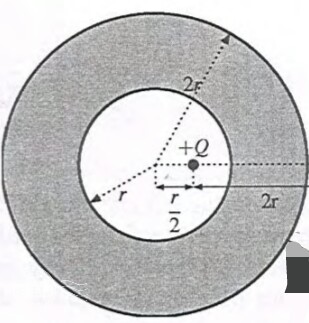
My teacher gave us a question today to just draw the charge distribution. The question goes like this

Suppose we have a neutral thick metallic shell of inner radius $r$ and outer radius $2r$. Now at distance of $\frac{r}{2}$ from its center , a positive charge of magnitude $Q$ is placed. How are charges induced (in terms of uniform and non - uniform distribution) on the inner and outer surface of the shell ?

He then explained that the charge on the inner surface of the shell is non uniform since the external charge is not at the center and I think it was quite intuitive but for the outer shell he suggested that the charge distribution is uniform but this time he didn't explain why he said this.

So please explain why the charge distribution should be uniform on the outer surface of the thick shell ?

Answer 1

So please explain why the charge distribution should be uniform on the outer surface of the thick shell ?

Because the sphere is a metal, the outer surface will be an equipotential surface. The electric potential is the same constant value over the entire outer surface (and at the inner surface and within the metal too).

There is no additional charge outside the outer surface of the sphere, therefore, in that region, the electric potential $\phi$ must satisfy the Laplace equation: $$ -\nabla^2 \phi = 0 \quad (\text{outside the sphere}) $$

Now we see that, outside the sphere, we have a spherically symmetric equation with spherically symmetric boundary conditions.

We already know one spherically symmetric solution to the Laplace equation: $$ \phi(r) \propto \frac{1}{r}\quad (\text{outside the sphere}) $$

This solution can be made to satisfy the boundary conditions by choosing $$ \phi(r) = \frac{Q}{r}\quad (\text{outside the sphere, gaussian units})\;, $$ or in SI units, $$ \phi(r) = \frac{Q}{4\pi \epsilon_0 r}\quad (\text{outside the sphere, SI units})\;. $$

Because the solution to the Laplace equation is unique, this must be the correct solution.

The associated electric field outside the sphere is $$ \vec E(r) = \hat r \frac{Q}{4\pi \epsilon_0 r^2}\quad (\text{outside the sphere, SI units})\;. $$

The charge distribution on the outer side of shell can be found by using the pillbox method. I'll leave that as an exercise, but it should be pretty clear that this charge distribution is also uniform on the outer surface since the field is spherically symmetric outside and zero inside.