What is the exchange particle in this Feynman diagram?

Question

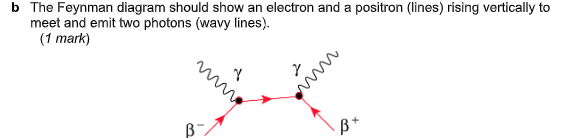
We just covered Feynman diagrams for the first time today, so please bear with me as a student who is not yet educated well in this subject. One question we did asked: "Draw the Feynman diagram for the interaction between an electron and a positron, producing two photons". The correct answer allegedly is this:

I understand that $\beta^-$, $\beta^+$ and $\gamma$ refer to the electron, positron and photons respectively, but we learnt Feynman diagrams as representative of boson interactions, or exchange particle interactions (is there a difference?), and in this diagram there is an unlabelled line running from the electron to the positron - all the diagrams we covered in class had labels with the relevant boson. I understand that this is the annihilation process of matter-antimatter, but what is the boson that triggers it? I've read around the subject (erratically and informally) and have heard of bosons for the EM force and strong/weak forces, but... an annihilation boson? I've no idea what this unlabelled line is supposed to represent, and I didn't have a chance to ask my teacher today.

If someone could please suggest what the unlabelled line is representing, and whether or not it even necessarily represents anything at all, I would be grateful. Also, are bosons synonymous with exchange particles in this context?

P.S. I am familiar with StackExchange guidelines (but never really use the physics site) and if this question doesn't make the cut as is stands I'll happily edit it.

Answer 1

There’s an error in your diagram which is abetting your confusion: the arrow on the positron line should point into the past, not into the future. This is a way of encoding that the electron and positron are, fundamentally, excitations of the same four-component quantum field. The mathematical transformation $\mathit{CP}$ which changes the particle into the antiparticle winds up being the same as the time-reversal transformation $T$.

Feynman and Wheeler were very excited by the idea that a positron is an electron moving backwards in time; Wheeler proposed, half-seriously, that perhaps there’s only one electron, zipping back and forth from the beginning of the universe to the end.

Every Feynman vertex must conserve electrical charge, lepton number, and various other quantum numbers that you’ll learn about later. So the line between your photon vertices must also be an excitation of the electron field. If it goes off into the future, it’s an electron; if it goes off into the past, it’s a positron; if it goes off in a spacelike direction, you’re in the middle of doing an integral.

“But then, why can’t an electron and a positron annihilate to make a single photon?” you ask. Because once you’re done, the initial state and the final state must also conserve energy and momentum; an electron-positron pair has a zero-momentum rest frame, but a photon doesn’t. Two is the minimum number of photons. Ortho-positronium, which has odd parity, decays to three photons.

Answer 2

I understand that 𝛽−, 𝛽+ and 𝛾 refer to the electron, positron and photons respectively,

Yes.

but we learnt Feynman diagrams as representative of boson interactions,

It's often said that bosons mediate interactions. In QED, the relevant boson is the photon; for now, to keep things simple, I recommend you not worry about others like the W and Z.

There's some truth to the statement that bosons mediate interactions (particularly if you look at what interaction terms are in the Lagrangian), but it can be misleading, because (as in your example) is not the case that only bosons are allowed to be internal lines in Feynman diagrams.

or exchange particle interactions (is there a difference?),

I think this kind of terminology is useful for describing things at a high level, but can be confusing in the kind of diagram you are considering. At least for me, I would describe the process where two electrons come in, exchange a photon on an internal line of the Feynman diagram, and two electrons go out, as an "exchange interaction mediated by a photon."

However, the electron-positron annihilation process isn't really like that, even though the Feynman diagram topology is similar. It's more like, an electron and positron meet and annhilate, and produce two photons.

The exchange process and the annihilation process are certainly related to each other, which is a deep insight of QED, but they are not the same physically or mathematically.

and in this diagram there is an unlabelled line running from the electron to the positron - all the diagrams we covered in class had labels with the relevant boson.

The internal line here is a fermion. I think you could call it either an electron going left to right or positron going right to left, either way you get the same result.

I understand that this is the annihilation process of matter-antimatter, but what is the boson that triggers it?

No boson triggers it. The annihilation process occurs because the electron and positron "collide."

Really, Feynman diagrams are just pictorial representations of a perturbation series. If you try to attach too much physical meaning to them, you can get confused. If you want to say in words what this Feynman diagram represents, you could say that an electron moves forward, emits a photon and changes direction, hits a positron, and the electron-positron annhilate and produce another photon.

But I think a more physical way to describe this process is that an electron and positron come in and annihilate, producing two photons. The intermediate line in the diagram is a fermion, because that's the only way to hook two QED vertices together and have an electron and positron as the initial state and two photons as the final state.