Best way to break a rope?

Question

What is a better way to break a rope, two kids pulling from opposite ends, like tug of war, OR , attaching one end of the rope to a wall and then the two kids pulling together.

I think the second one may be better, as in that case, if the kids apply $F_1$ and $F_2$ force, the tension turns out to be $F_1+F_2$ , but in the first case, it is $|F_1-F_2|$.

I am not sure if this correct, my explanation seems very "weird" to me, and as I am just starting physics (this was a problem in HRK), I can't yet judge my answers by myself, so I would really appreciate it is someone could please say if my explanation is correct, and if not, then what the correct explanation is.

Answer 1

Two kids pulling on the same end of a rope with forces $F_1$ and $F_2$ will generate a tension of $F_1+F_2$ in the rope.

Two kids pulling at opposite ends of a rope cannot pull with forces of $F_1$ and $F_2$ respectively, as the tension in the rope has to be constant along its length and at both ends. In practice, if the maximum forces the two kids can apply are $F_1$ and $F_2$ respectively, and $F_1<F_2$, then there are two possibilities. Either the rope will start to slip from kid_1's fingers as the force rises infinitesimally above $F_1$, so kid_2 can only apply $F_2$ - so tension in the rope is $F_1$. Or, if kid_1 doesn't let go and kid_2 applies $F_2$, then kid_1 will be dragged across the ground in accelerated motion (kid_2 will also have to accelerate backwards to maintain the force on the rope) and the tension will rise to $F_2$.

In practice, you don't even need kid_1 in the latter case. If the rope was tied to a feather at one end and kid_2 applies a constant force of $F_2$ on his/her end of the rope, then there will be a tension of $F_2$ in the rope (of cource, the resultant accelerations of feather and rope may be absurdly unlikely depending on the magnitude of $F_2$).

Answer 2

Two kids pulling on the same end will create more tension in the rope. Per equal but opposite action and reaction the wall will exert the same pull on the rope as the two kids exert whereas one kid at each end will exert one half the tension.

Answer 3

What is a better way to break a rope, two kids pulling from opposite ends, like tug of war, OR , attaching one end of the rope to a wall and then the two kids pulling together.

It's highly unlikely the kids could break a rope, but it would be more likely to occur if the two kids pulled together. But not due to your calculations.

For the first case, the tension in the rope would not equal $|F_{1}-F_{2}|$. If it did equal the tension, then if each kid pulled with the same magnitude of force $F$, then the tension would be zero, when in fact the tension would be $F$ due to Newton's 3rd law.

The tension will equal the greater force of the two, say $F_1$, and the kid pulling with the lower force, say $F_2$ will experience an acceleration toward Kid 1 of

$$a_{2}=\frac{|F_{1}-F_{2}|}{m_2}$$

Now let one of the kids tie his/her end of the rope to the surface of a building and join the other kid so that each kid pulls on the rope for a total force of $|F_{1}+F_{2}|$. Assuming the building does not move, the building will exert an equal and opposite force of $|F_{1}+F_{2}|$. This again assumes that that the maximum total pulling force by the kids is not limited to something less due to the maximum possible static friction force between the kids feet and the ground being exceeded.

UPDATE:

Thank you so much for your answer, could you please explain in the first case, why tension will be $F_1$ and not $F_2$?

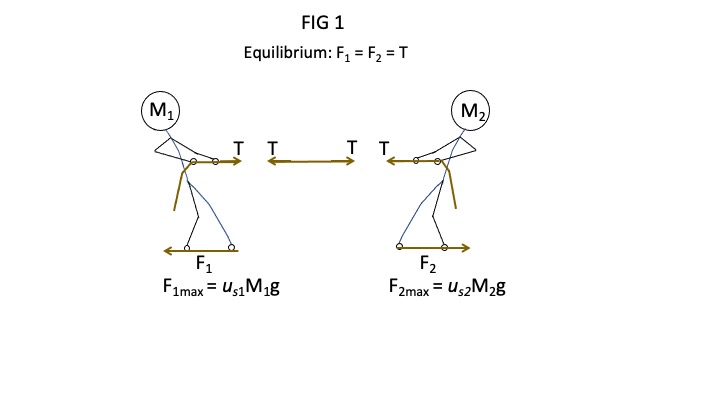
To understand why you need to realize that the forces $F_1$ and $F_2$ are not necessarily the forces on the kids arms. The forces on the kids arms will always be equal to each other and equal to the tension in the rope (assuming an ideal rope), per Newton's 3rd law. See FIG 1.

Forces $F_1$ and $F_2$ are the friction forces between ground and the feet of kid 1 and kid 2 respectively. The friction force resists the tension in the rope. As long as these friction forces are static friction, they will always match the tension in the rope making the net force on each kid zero as shown in FIG 1.

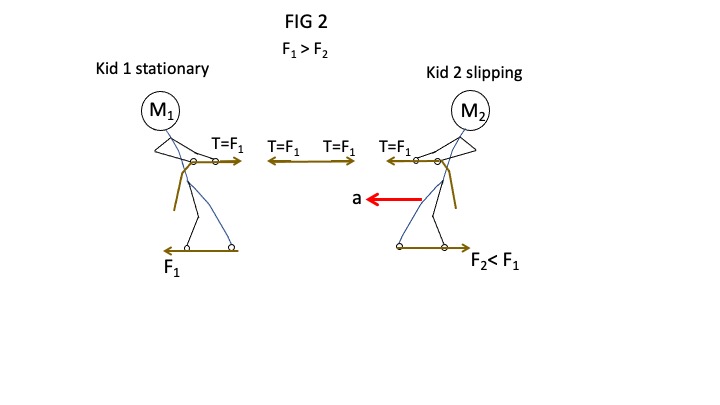
On the other hand, if the maximum possible static friction force is exceed for either kid, that friction on that kid becomes a kinetic friction force, which is generally less than the max static friction force. This is shown in FIG 2 for the case where kid 2 slides because the kinetic friction force $F_2$ is less than the static friction force $F_1$ on kid 1, for a net force on kid 2 of $F_{1}-F_2$, and an acceleration of kid 2 per Newton's 2nd law.

Hope this helps.