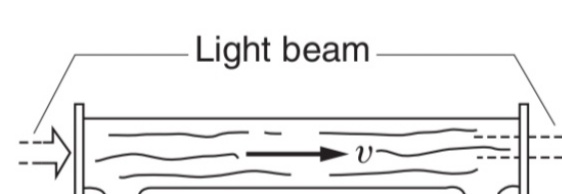
I'm in a frame in which a medium is at rest, and I observe light move at some speed.
Another person observers this medium move at some constant speed, in this case he'll observe a different speed for light.
But the principle of relativity states that the laws of physics have the same form in all inertial systems therefore Maxwells equations should have the same form in both the two frames, which implies that the speed of light should be the same in both frames.
Can anyone please point out my error. Thank you.
