Period of a pendulum in free fall

Question

Let's say I have a pendulum hanging from a bar that's fixed to the wall of an elevator. Assume that there's no air or anything inside the elevator, that the string of the pendulum is very light and that the bob of the pendulum is more or less a heavy point mass. After setting the pendulum in motion, the elevator starts going down, increasing the period of the pendulum, until the cable holding the elevator runs out and brings the whole contraption into a free fall situation.

The formula for the period of a pendulum with length $L_0$ where the bob experiences a gravitational acceleration of $a_0$ is: $T = 2 \pi \sqrt{\frac{L_0}{a_0}}$. In free fall, $a_0 = 0$ so the pendulum wouldn't swing at all.

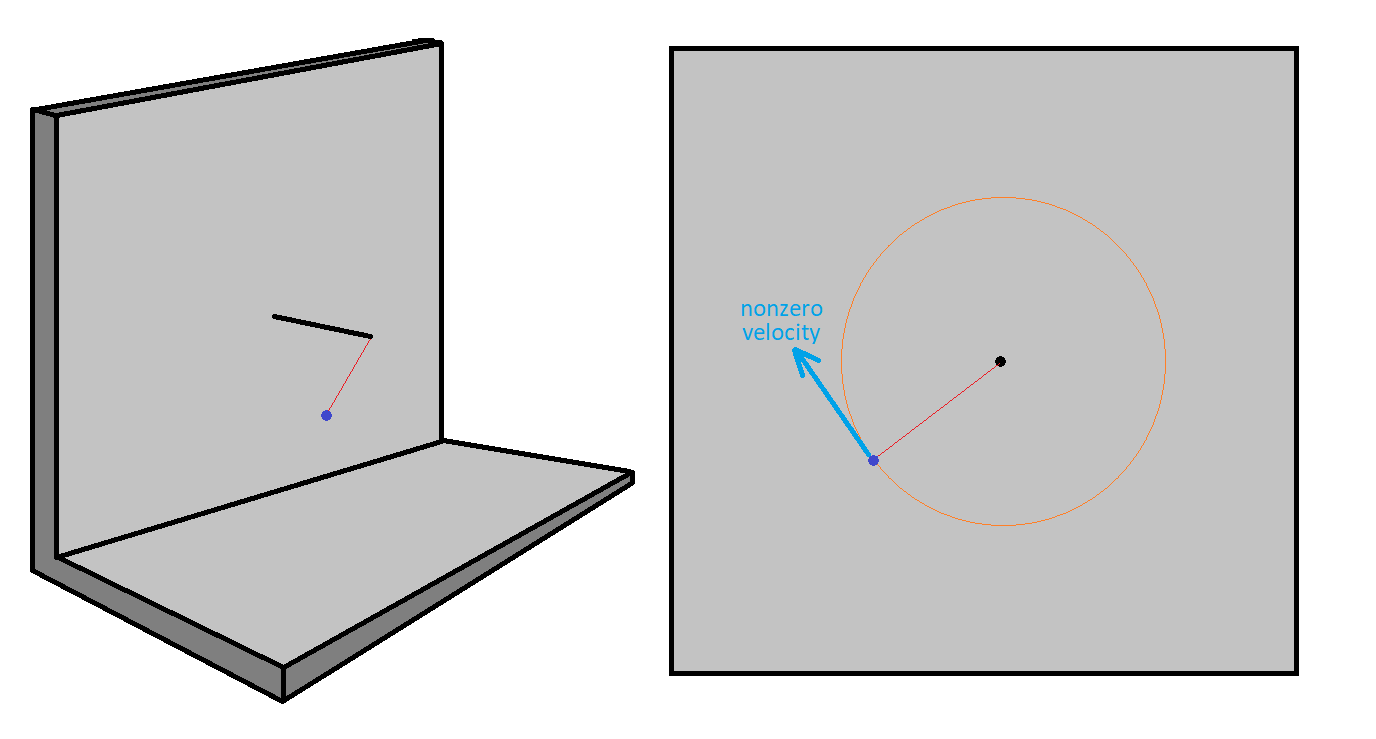
However, in my hypothetical situation, bob of the pendulum could've had a velocity right before going into free fall, so wouldn't the pendulum transition into a uniform circular motion which gives rise to a new period?

If so, shouldn't there be a better formula to describe the period of a pendulum that also correctly predicts the period depending on how the acceleration on the bob changes with respect to time?

Answer 1

As long as there is a net velocity on the pendulum bob at the moment the elevator goes into free fall, the pendulum will go into uniform circular motion.

The formula you have stated for the time period is only valid for a pendulum. Once the bob goes into circular motion, it is no longer a pendulum as there is no restoring force acting on the bob. The formula still makes logical sense as the bob will never reverse its direction and will hence take infinite time to come back to its starting path.

Answer 2

$T=2\pi\sqrt{L_0/a_0}$ is the period of a simple pendulum of length $L_0$ with small-angle oscillations. The parameter $a_0$, sometimes also denoted as $g$, is usually the acceleration due to gravity, but I suppose it is technically the acceleration due to some constant force that is proportional to the mass of the pendulum bob. So the equation of motion obtained from Newton's second law is

$$\frac{\text d\theta^2}{\text dt^2}=-\frac {a_0}{L_0}\cdot\sin\theta\approx \frac {a_0}{L_0}\cdot\theta$$

However, in free fall the equation of motion becomes $$\frac{\text d\theta^2}{\text dt^2}=0$$

And here is the issue. This second equation does not give you a unique period! You can have any period you want with $\ddot\theta=0$ depending on the initial conditions.

Linking this back to your period equation, note that when $a_0=0$ we get an undefined value, which is what we just determined above. So technically, $T=2\pi\sqrt{L_0/a_0}$ is still a valid equation for your free-fall scenario: it tells us the period is not defined by this equation, which makes sense. The period is instead defined by the angular velocity $\omega_0$ when free fall began: $$T=\frac{2\pi}{\omega_0}$$

Answer 3

Look at the forces acting on bob, when elevator's acceleration is $g$, in the axis which is perpendicular to velocity of pendulum. Let angle between rope and $y$ axis be $\theta$. So: $$\frac{mv^2}{l}=T+ma\cos(\theta)-mg\cos(\theta)\mathrel{\stackrel{{\mbox{ a=g}}}{=}}T$$ So: $\frac{mv^2}{l}=T$. And there's no force in direction of velocity there's only perpendicular to it velocity doesn't change. So our equation is just for circular motion of bob. If you want to find period of this motion you'll need velocity at time that $a(t_1)=g$. Our period will be $$\tau=\frac{2\pi l}{v}$$And for this you'll need to find $\theta(t)$. And you can find it by the equation $$\ddot{\theta}=-\frac{g-a(t)}{l}\theta$$ I think it's impossible to find this without knowing $a(t)$. And velocity at time $t_1$ will be $l\dot{\theta}(t_1)$. And coming to better formula for period, if you look at equation for motion at time $t_1$ you'll see $$\ddot{\theta}=0$$ And solution for this is $\theta=c_2t+c_1$ from this equation you see that there's no sign of harmonic motion and there's no period of harmonic motion which is valid for our formula $T=2\pi\sqrt{\frac{l}{a_0}}$

I hope I have answered to your question