-1
$\begingroup$

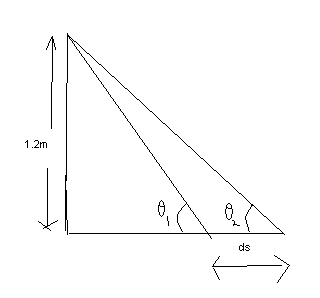
An object is placed on a frictionless table with its one end attached to a cord which is connected to a pulley and the tension is maintained constant at 25 N. what is the change in kinetic energy of the object from its position 3m away to 1m away from the raised platform?

poorly drawn, so not at all to scale...just shows the setup.

I think that we should integrate work with respect to theta here, over the interval $\tan^{-1}(1.2/3)$ to $\tan^{-1}(1.2/1)$. But for that, we need to differentiate work with respect to $\theta$, right? So doesn't it end up the same if I differentiate it and then integrate it? Or do I integrate the expression $F s \cos(\theta)$ with $\mathrm{d}\theta$ without differentiating it first?

Improve this question
$\endgroup$
10
  • $\begingroup$ Are u trying for JEE? :) $\endgroup$
    – Sreekanth Karumanaghat
    Commented Apr 7, 2013 at 11:43
  • $\begingroup$ Yes...although my classes haven't started yet. But tell me, is it one of the tough questions in that category, or easier? Btw, I found this in Halliday walker book $\endgroup$
    – Saurabh Raje
    Commented Apr 7, 2013 at 14:20
  • $\begingroup$ there are small problems in the question.. the string has to be attached to the centre of the block and the distance "1.2m" should be distance between centre of block and the top of the platform... $\endgroup$
    – Sreekanth Karumanaghat
    Commented Apr 10, 2013 at 7:22
  • 1
    $\begingroup$ this isnt one of the toughest problems as far as JEE is concerned I would rate this as medium complexity. The tough questions will be a combination of mechanics,electro magnetism and themodynamics.. :) JEE is the toughest engineering entrance in the whole world.. even MIT entrance test is easier, all the best keep a back up plan...ie there is no guarantee that u will succeed even if u study 10 hrs a day for 3 years.. dont expect too much... make ur fundas strong.. $\endgroup$
    – Sreekanth Karumanaghat
    Commented Apr 10, 2013 at 12:27
  • 1
    $\begingroup$ I havent heard about vidyamandir... yeah HC Verma is physics.. sorry I forgot chemistry books names. $\endgroup$
    – Sreekanth Karumanaghat
    Commented Apr 11, 2013 at 17:23

3 Answers 3

Reset to default
0
$\begingroup$

As the work $W=\int\vec F. {d\vec s}$ The work will be only dependent on the component of force along the displacement , or otherwise the length of rope moved along the force.

I hope we are done!.

Now you can try it.

Improve this answer
$\endgroup$
2
0
$\begingroup$

First of all, you pretty much never need to integrate work. There is no formula I can think of which involves the integral of work.

Secondly, just because you are going to be integrating something over $\theta$ doesn't mean you have to differentiate something with respect to $\theta$. That is one way to do the problem, but it may not be the most straightforward way. I would suggest going back to the formula $W = \int \vec{F}\cdot\mathrm{d}\vec{s}$ and trying to express it in terms of $\theta$. Drawing a diagram and doing some geometry should help.

Improve this answer
$\endgroup$
10
  • $\begingroup$ Won't just calculating the length of rope pulled out be enough to get work done? $\endgroup$
    – ABC
    Commented Apr 6, 2013 at 4:51
  • $\begingroup$ Well that was integral of dW...sorry about the typo, $\endgroup$
    – Saurabh Raje
    Commented Apr 6, 2013 at 5:21
  • $\begingroup$ How would I get f in terms of dS? Isn't fcos(theta) along displacement? So fcos(theta) is changing with a change in theta...so shouldn't I integrate it with theta? $\endgroup$
    – Saurabh Raje
    Commented Apr 6, 2013 at 5:28
  • 2
    $\begingroup$ @exploringnet sure, but then you bypass all the practice you would get doing integrals ;-) $\endgroup$
    – David Z
    Commented Apr 6, 2013 at 6:32
  • 1
    $\begingroup$ @SaurabhRaje You will need to choose one variable to parametrize the motion. It could be $\theta$ or $s$ for example; any variable that takes a different value at every point along the path. Then you need to express everything else in terms of that variable. $\endgroup$
    – David Z
    Commented Apr 6, 2013 at 6:33
0
$\begingroup$

We should note that theta varies here... As far as the kinetic energy change is concerned.. Kinetic energy change is nothing but work done by a varying force... At any point work done is W = F s $\cos(\theta)$ here it has been mentioned that F is maintained a constant we are only concerned about Fcos theta wer theta varies from $\tan^{-1}(1.2/3)$ to $\tan^{-1}(1.2)$

so the ultimate formula is W = $\int \vec{F}\cdot\mathrm{d}\vec{s}$

We have to find a relation between ds and d$\theta$ by some geometric method and approximation finding the relation between a small change in linear and angular displacements in the triangle

d$\theta$ is $\theta$2 - $\theta$1 and ds is S2- S1 we need to establish a relation between ds and d$\theta$ in the process we can use approximation that d$\theta$ is very small and ds too is very small.. I have not been able to do that yet.. if any one is able to do that lemme know.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.