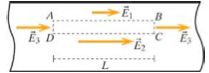 

$\Delta V_{ABCDA} = - \int_A^A \vec{E} \dot{}d\vec{l}$
The requirement that the round-trip potential difference be zero means that $E_1$ and $E_2$ have to be equal. Therefore the electric field must be uniform both along the length of the wire and also across the cross-sectional area of the wire. Since the drift speed is proportional to $E$, we find that the current is indeed uniformly distributed across the cross section. This result is true only for uniform cross section and uniform material, in the steady state. The current is not uniformly distributed across the cross section in the case of high-frequency (non-steady-state) alternating currents, because time-varying currents can create non-Coulomb forces, as we will see in a later chapter on Faraday's law.
I understand whySay the electric field could be non-uniform across a cross section ofis non-uniform materialbut completely longitudinal. But exactly what part ofThat would result in the integral above argument (the voltage across a circular path mustbeing non-zero, which is impossible, so the field always has to be zero) failsuniform if its longitudinal.
If the cross sectionwire is made of different types of material, the different parts would act as dielectrics, which would cause the material to polarize and dilute the electric field at different points, would cause the electric field to be non-uniform materialbut still longitudinal, which contradicts the point made in the paragraph above.
How can I resolve this apparent contradiction?
