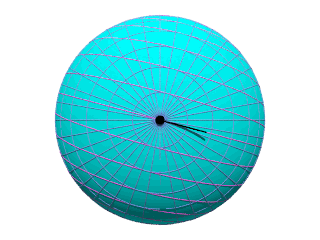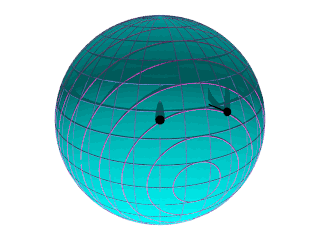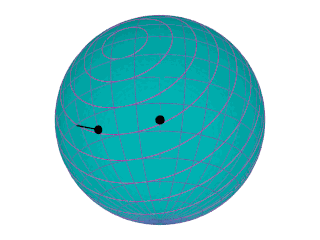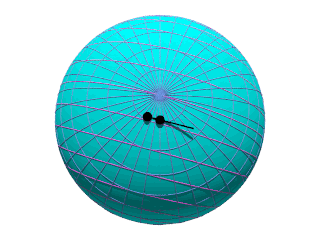
Angular velocities add like vectors. The relative angular velocity of the two particles is zero. You have to think of it as being in the rotating frame of one particle and looking at the other. So, if you are satsitting on the disc next to one particle, going around with it, what is the apparent motion of the other particle in this rotating frame?
If the angular velocities are not identical, they still add like vectors, but the result is rather unintuitive.
Consider two aircraft flying due north at the same speed, crossing the equator at the same time, but 30 degrees$30^\circ$ apart in longitude. What is their relative velocity?
Intuition suggests their relative velocity should be zero, but we are talking about motion on a spherical planet, so in fact, they are both rotating about axes at right angles to the plane of the great circle they follow. The difference between these (the relative angular velocity) is a much shorter angular velocity vector pointing towards the point on the equator half wayhalfway between their paths. The relative angular velocity has each spinning about this point relative to the other. This is hard to see, so we need an animation.
In the figure below, first, watch the blue lines of latitude and longitude. You can see that the two aircraft start at one pole, move along their own meridian to the equator, separatingseparate, and then converge on the other pole. The relative motion of one aircraft, as seen in the frame of the other, forms a figure-8 shape. Note that when crossing the equator, the relative velocity is non-zero.
If we now watch the red lines, we see that at every point on its trajectory, the relative velocity is pointing parallel to the red circles. It'sIts relative velocity is spinning around the mid-point, but the midpoint is itself moving in this rotating reference frame.
Relative angular velocity is perfectly meaningful, and follows the usual vector addition rule, even when the rotation axes are not parallel. But the result is unintuitive.