How exactly prove (11.50) and (11.52) from Lorentz transform ?

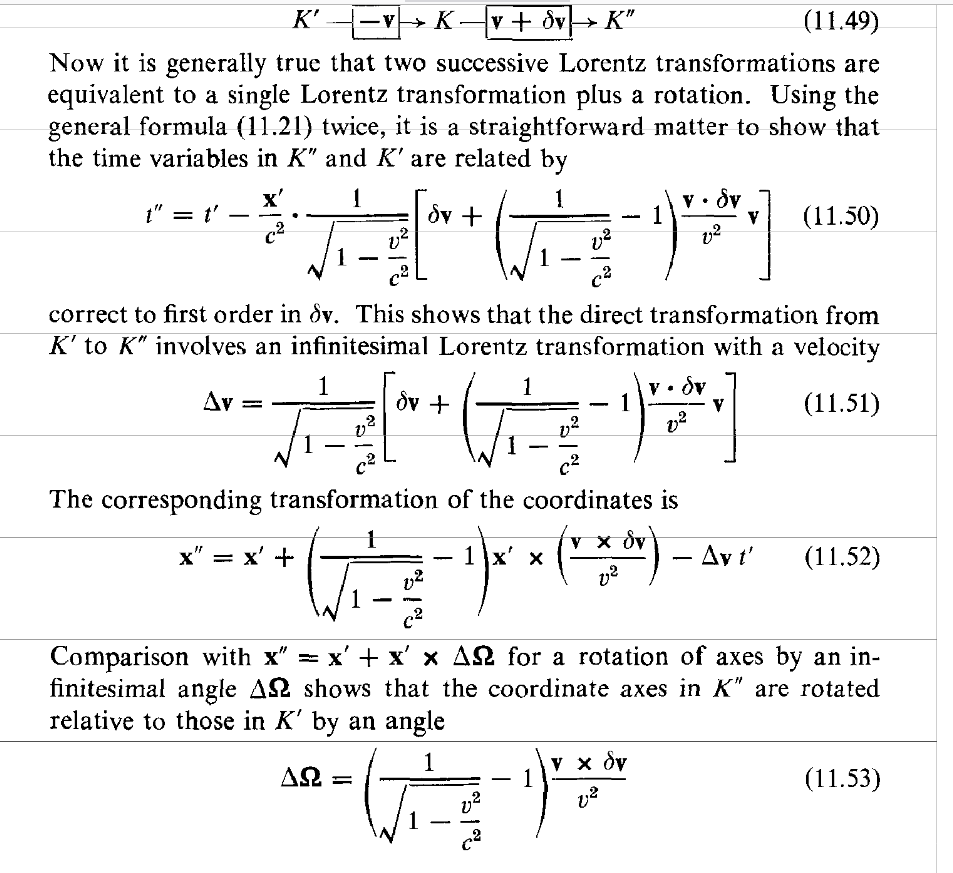
How exactly prove (11.50) and (11.52) from Lorentz transform ?

$ t'' = t' - \frac{\mathbf{x'}}{c^2} \gamma (\delta \mathbf{v} + (\gamma - 1) \frac{\mathbf{v}\mathbf{\delta v}}{v^2}\mathbf{v})$

$ \mathbf{x''} = \mathbf{x'} + (\gamma - 1)(\mathbf{x'} \times (\frac{\mathbf{v} \times \mathbf{\delta v}}{v^2})) - \Delta \mathbf{v} t'$