I am a beginner in QFT,so let me introduce my question by the problem in MIT8.323 (2023 spring,by Hong Liu) pset 1
.
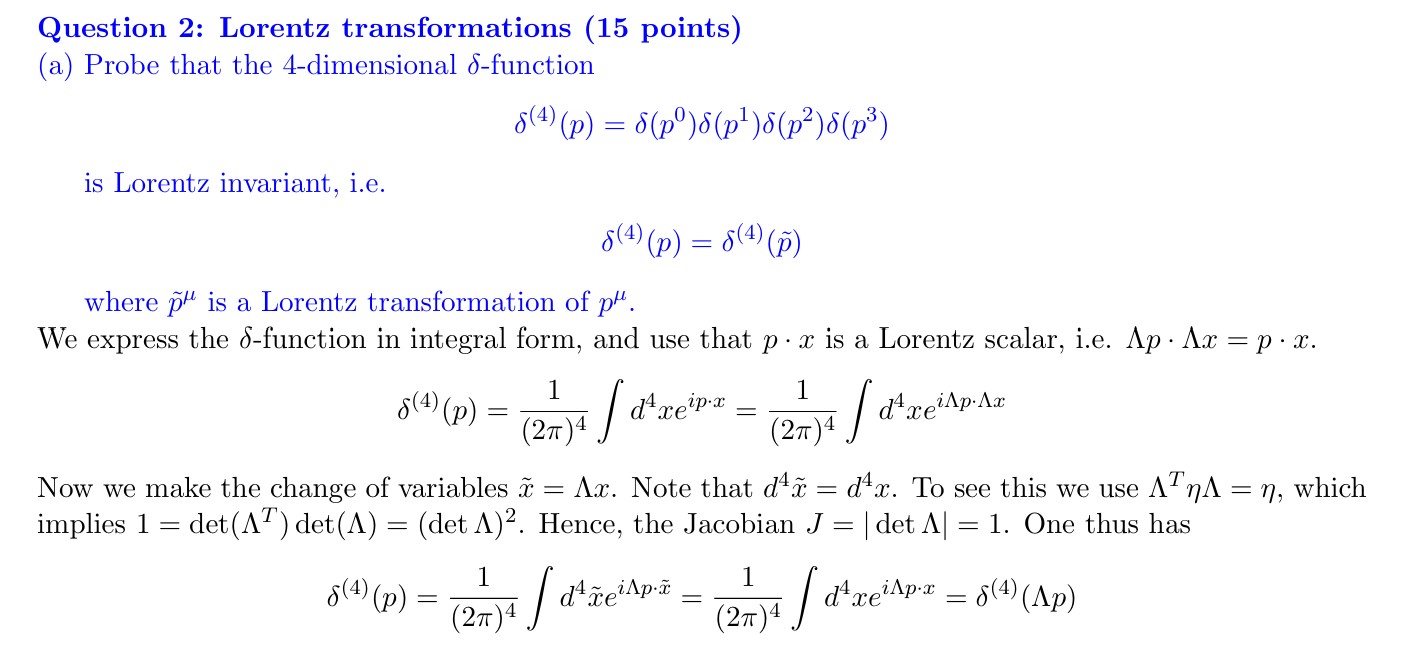
All steps seem clear, the measure is invariant because the Jacobian is 1 and the contraction $p^\mu x_\mu$ is invariant.
But now let's consider another point of view. Now we treat the $\delta$ function as the integral on $(R^4,\eta)$,and the Lorentz transformation is a coordinate transformation on $R^4$,so this integral is invariant.
But there is a subtlety,because when we prove a integral on a manifold is independent to the choice of coordinate chart $\phi$,we need to introduce the pullback of the form $\omega$,i.e. we have $\int_{\phi(U)} (\phi^{-1})^*(\omega)$ is invariant. but in this problem we don't use the pullback of a Lorentz transformation,how this happens?Are they the same thing?
EDIT:to make my question more clear.Let's consider a form $\omega =f(x^1,x^2)dx^1 \wedge dx^2$.Now we do a coordiante transformation $x$ to $y(x)$,then is it correct that the form become $$f(x^1(y^i),x^2(y^j)) \det {M }\space dy^1 \wedge dy^2=g(y^1,y^2) \det {M}\space dy^1 \wedge dy^2$$
And then the change of integral domain denotes a $\det M^{-1}$ so the integral remains the same.
In this question,why $p$ also changes into $\Lambda p$?Physically its obivous,but how this holds mathematically?
