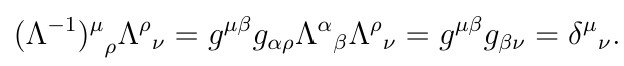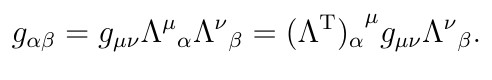We define Lorentz transformation as $\Lambda$ satisfying:$$\eta_{\alpha\beta}=\eta_{\mu\nu}\Lambda^{\mu}_{\space\space\alpha}\Lambda^{\nu}_{\space\space\beta}$$
And its inverse$$(\Lambda^{-1})^{\mu}_{\space\space\nu}:=\eta^{\mu\beta}\eta_{\nu\alpha}\Lambda^{\alpha}_{\space\space\beta}$$$$(\Lambda^{-1})^{\mu}_{\space\space\nu}:=\eta^{\mu\beta}\eta_{\nu\alpha}\Lambda^{\alpha}_{\space\space\beta}.$$
Because we have
But there exists a subtlety, if we use the two $\eta$ in the definition of $\Lambda^{-1}$ rising index $\beta$ up and lowing index $\alpha$ down, we have $$(\Lambda^{-1})^{\mu}_{\space\space\nu}=\Lambda_{\nu}^{\space\space\mu},$$
where the RHS is usually defined as the transpose of the matrix form of $\Lambda$, but we know that $\Lambda^{-1}=\Lambda^T$ is not right in general. How does this happen?
Even more confusingly, we have
This implies the matrix equation $\Lambda^T \eta \Lambda=\eta$, which is indeed how we define the Lorentz group mathematically, as the elements in $\mathbf{O}(1,3)$ preserving the inner product with respect to the metric $\eta$.