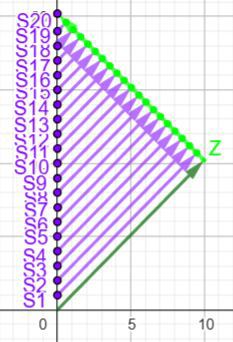
Normally we would expect the supernova events to be randomly distributed in the galaxy, but that makes the analysis complicated, because what john actually sees depends on whether he is travelling towards or away from the events. Initially, let's just suppose the supernovae occur at the same location at the edge of the Galaxy at a frequency of once every 10,000 years in the earth reference frame. John is sent to investigate this strange region. The spacetime diagram for his journey is shown below::
The horizontal axis id distance and the vertical axis is time and both axes should be be multiplied by 10,000. From the diagram it can be seen that John sees 20 supernova events (the purple events) on his outward leg (the green worldline with a velocity close to c). Whenever he sees a supernova, he reports this back to Earth (the orange signal vectors). On arrival at Z, 500 years has passed according to John's clock and he sees the supernovae occurring with a frequency of once every 25 years. Earth calculates that John should arrive at Z after about 100,000 years earth time and in that 100,000 years Earth has only observed 10 supernovae. John has seen twice as many, essentially due to the Doppler effect due to moving towards where the signals are coming from. On arrival at Z, John sends a signal back to Earth stating he has seen 20 supernovae (the red signal vector), but Earth does not receive this information until 200,000 years have passed, by which time Earth will also have seen the same 20 supernovae events. If John turns around immediately at event z and returns to Earth at near the speed of light, he will not see any more supernovae from region Z, because he is outrunning the light from them and on his return he will have seen just as many supernova events as Earth did.
Now lets analyse what John sees if all the supernova events happened close to Earth (ignoring the damage to Earth).
In this new spacetime diagram John does no see any Supernova events on his outward journey (except $S0$ which occurred exactly as he was about to leave), because he is outrunning the light from those events. On arrival at Z he only seen one event and Earth has seen 10 supernova events at the time Earth calculates John has arrived at Z. After John turns around he sees all 20 events on his return trip and now he is seeing the supernova events occurring with a frequency of once every 12.5 years by his clock. (this is counterintuitive, if you expect John to see the evolution of the Galaxy as time dilated). John arrives back at earth shortly after the signal he sent from Z informing earth that he had not seen any events on his outward journey.
In both scenarios, John sees the same number of supernovae as Earth sees after completing a round trip and returning to Earth. It can reasonably be concluded that John will see exactly the same amount of supernova events as Earth sees, no matter where the supernova events are located in the galaxy.