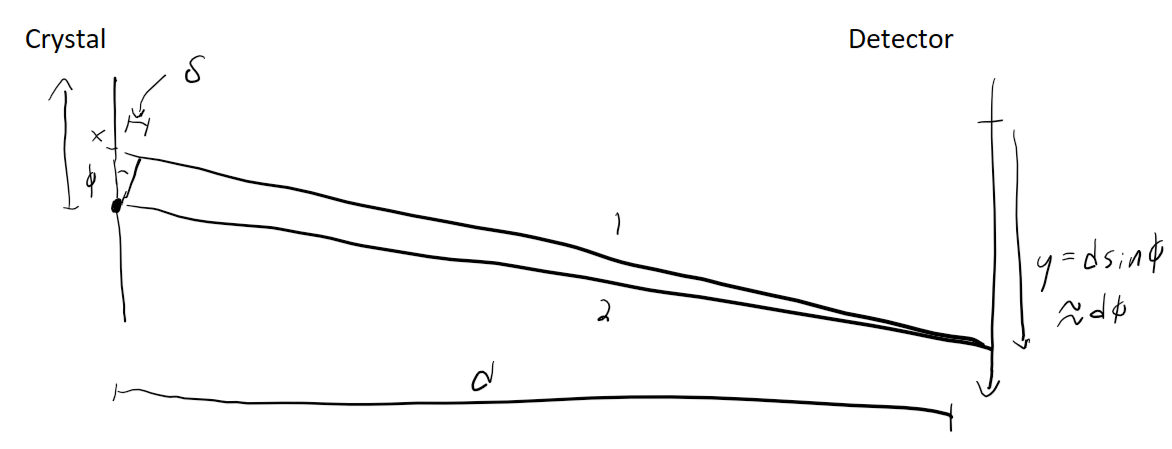
The relationship between lattices and reciprocal lattices can be derived in the Fraunhofer approximation, which says that the deflection of the light rays leaving the solid and hitting the detector is small. Your question can be answered in 1D, so let's work through that case for simplicity. In the picture below, this means that $d \gg x, y$. It also implies that the two rays labeled 1 and 2 are of approximately equal length, but differ by some small amount $\delta \approx \phi x \approx x y / d$. The amplitude of a light ray emitted from a point $x$ in the object and propagating along one of these rays to the detector is then
$$e^{2\pi i (d+\delta) / \lambda} \propto e^{2\pi i x y / \lambda d}$$
Adding up the fields from every point in the object gives the field at the detector
$$\int_{-\infty}^\infty f(x) e^{2\pi i x y / \lambda d} dx = \tilde{f}(y / \lambda d)$$
where $f(x)$ is the scattering amplitude of the object at position $x$ and $\tilde{f}(k)$ is the Fourier transform of $f(x)$. This derives the connection between diffraction patterns and reciprocal lattices.
The probability to detect a photon at position $y$ is given by the magnitude squared of the above. As you can see, the diffraction pattern gets scaled by $\lambda d$, so it does indeed depend on the wavelength, as you guessed.
Finally, what about tilting the object by an angle $\theta$? This has two effects. One is that it changes the relative distance $\delta$ from the object to the screen by a distance $x \sin(\theta)$. However it also changes how far the incident light must travel before hitting the object, so the net effect on the phase of the light hitting the detector is unaffected by this tilt.
The second effect is, as you mention, that the projection of the object along the propagation direction of the light. This effectively contracts the size of the object according to the transformation $x \rightarrow x \cos \theta$$x \rightarrow 1 / \cos \theta$. Plugging this into the first equation, you can see that this has the effect of expanding the diffraction pattern by a factor $1/\cos\theta$.