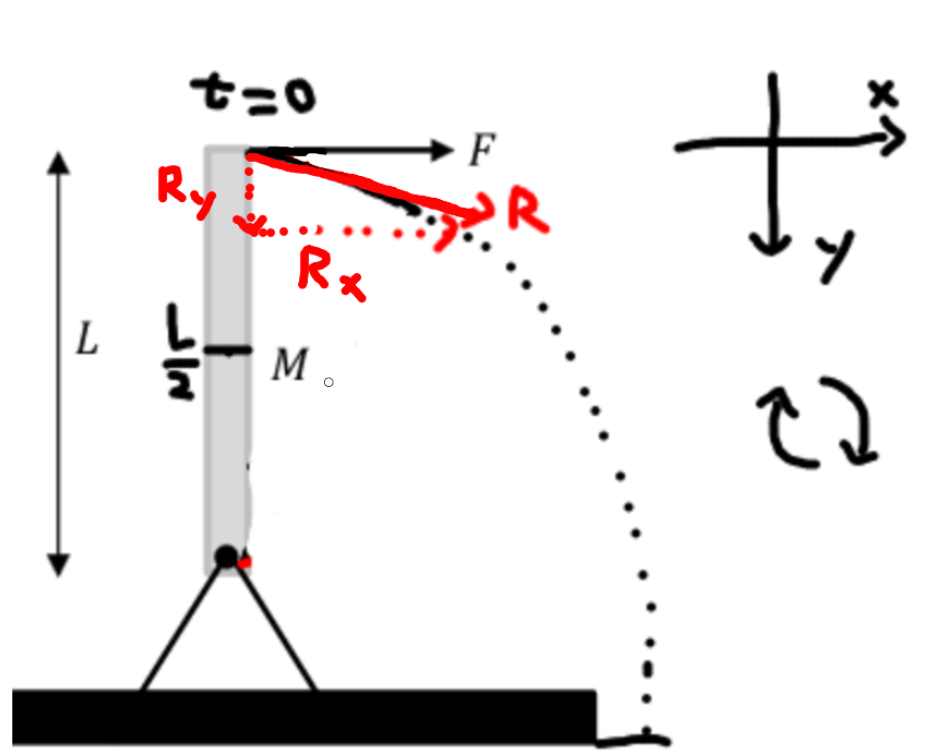
the question is regarding a problem in Rotational Motion for rigid bodies in AP Physics 1. I have tried to translate it as best as I could from Swedish. The problem is as follows: " A homogeneous rod with length L and mass M is fixed to the horizontal axis. The rod can freely move around its axis. Initially, the rod is standing vertically. At t=0, a force F is applied at the end of the Rod (see image)
What is the magnitude of the Reaction Force, R, that the axis of rotation applies to the rod at t=0? "
"A homogeneous rod with length $L$ and mass $M$ is fixed to the horizontal axis. The rod can freely move around its axis. Initially, the rod is standing vertically. At t=0, a force F is applied at the end of the Rod (see image)
What is the magnitude of the Reaction Force, R, that the axis of rotation applies to the rod at t=0?"
I have tried to draw a Free-Body Diagram with the "Reaction Force" split into its x- and y-components. My teacher has published the solution, which is $R=\sqrt{(( M\cdot g)^2+( \frac{1}{2} \cdot F)^2)}$ with no explanation... I understand that we are finding the hypothenuse R via Pythagoras but it is especially the fact that the solution implies that $Rx = \frac{1}{2} \cdot F$. I cant seem to wrap my head around as to why though? And how do we know so?