You are too optimistic about the possibilities. With Babinet's principe you can only replace a mask by its inverse. So where it is transparent it becomes blocking and vice versa.
If you have a mask which represents a big screen with many small holes, then Babinet's principle converts it to the case where you only have the many separate small fill patterns where the holes previously were. But that doesn't mean you can separately solve the light distribution, or EM wave patterns, for all those little pieces and then add the results. They might still influence each other electromagnetically, so you are only allowed to solve the problem of the little pieces combined. And then (following the Babinet procedure) use that result to get back the result for the original screen.
Of course if the small pieces are far from each other then you can treat them separately, but then of course you could also have started by treating the holes of the original problem separately. The most useful part of Babinet's principle is that if you have an infinite (or very large) screen to begin with, you transform it to a much smaller size problem.
Now in the picture you show, it is not exactly clear what is the case:
- Your screen might be very large (i.e. sufficiently big to be treated as infinite) and the picture just does not show the full size. In that case Babinet's principle would help, for instance, if you want to use it as input into an EM simulator. With Babinet you have as the only object in the input the cross, not the large screen.
- Your structure may be exactly as shown. Then Babinet makes it probably worse, you will end up with an infinite screen containing a square hole in which a cross sized floating piece is present. Not likely to help.
In no case can you (based on Babinet's principle) cut the cross in, say, 5 squares and then only compute the case of one square, later combining 5 copies of the result.
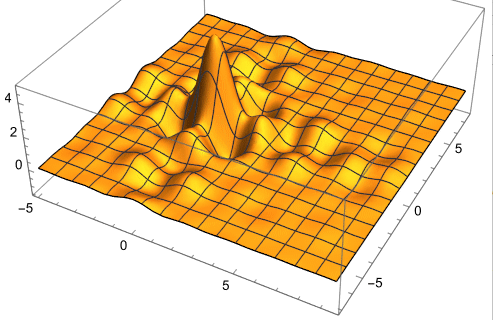
EDIT(EDIT) PS: With other methods, like Fraunhofer diffraction , you actually can get away with splitting objects into pieces (if you combine them later in the right way). So let's also not be too pessimistic, the procedure you propose might work after all. For instance with the equation after the sentence: "Explicitly, the Fraunhofer diffraction equation is" in [ref 3]. You could just assume $A(x,y)=1$ if you illuminate with a plain wave. And treating the cross as two crossed $1\times 3$ rectangles minus a $1\times 1$ square to undo the double counting, leads immediately to the diffraction pattern in terms of $\text{sinc}$ functions: $$ \frac{\sin(3x)}{x}\cdot \frac{\sin y}{y} + \frac{\sin x}{x}\cdot \frac{\sin(3y)}{y} - \frac{\sin x}{x}\cdot\frac{\sin y}{y} $$ where scaling to the right size is left as an exercise for the reader. Here's a quick plot: