In Newtonian physics there is just a single "escape speed" for a body with some mass and gravitational field. If you launch an object at greater than this speed and outward fro the surface at any angle, then that object has a total energy (kinetic, potential and rest-mass) $>mc^2$ and will escape to infinity.
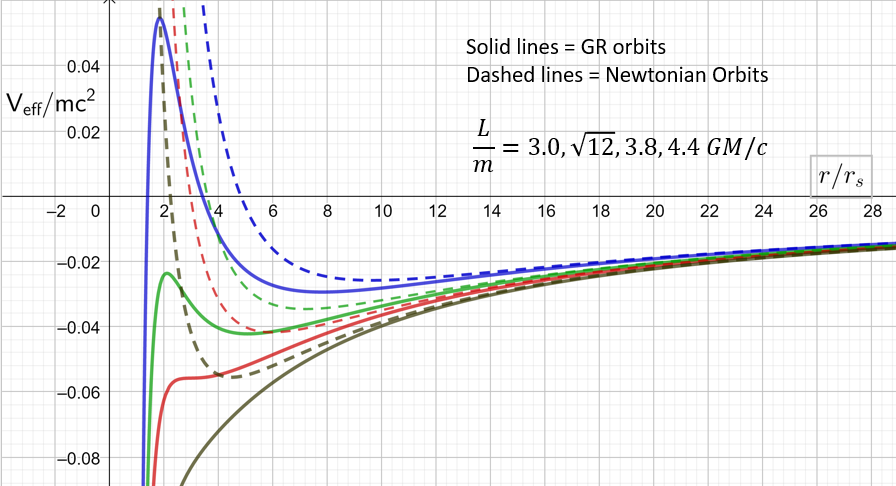
This is not necessarily the case in General Relativity. If we consider the Schwarzschild metric, then the effective potential that a particle moves in can have a local minimum (like in Newtonian physics, which defines the limit of a stable bound orbit) but also, if the specific angular momentum is $>\sqrt{3}r_sc$, a local maximum, which is quite unlike the Newtonian case (see below).
Effective potential in the Newtonian and Schwarzschild (GR) cases as a function of the specific angular momentum ($L/m$). Solid lines show the GR case, dashed lines are the equivalent in Newtonian physics. The solid blue line shows an example where an object launched from a neutron star surface with that value of $L/m$ (i.e. at an angle to radially outward) would need a total energy greater than some threshold corresponding to the local maximum in the effective potential in order to escape to infinity.
The local maximum is at a position $3r_s/2 < r <3r_s$, where $r_s$ is the Schwarzschild radius and if the specific angular momentum of any inertial (ballistic) body is $>2r_s c$ then the local maximum can be such that a body travelling outwards from a radial coordinate less than the local maximum and with total energy of $mc^2$ will encounter a potential barrier and fall back inwards -a so-called trapped orbit.
What this means is that a body launched directly outwards (with no angular momentum) from a neutron star will behave like in Newtonian physics - if its total energy is $>mc^2$ it will escape. However, if the body is launched with the same total energy but at an angle so that it has a specific angular momentum $>2r_s c$ then it is possible for that object to fall back towards the neutron star surface after attaining a maximum radial coordinate somewhere between the neutron star surface and the position of the local maximum in the effective potential. i.e. The escape speed becomes dependent on the launch angle.
The same is true ofSomething similar happens for light. If a neutron star has a radius of $<1.76 r_s$, then although radially directed light will always escape, there is a threshold angle to the normal to the surface beyond which an emitted light beam will fall back and hit the neutron star surface. For a neutron star of larger radius, light will always escape in whichever (outward) direction is is launched - see How small would a neutron star be to see the entirety of it? . Also see Falling angle of a photon near the event horizon .