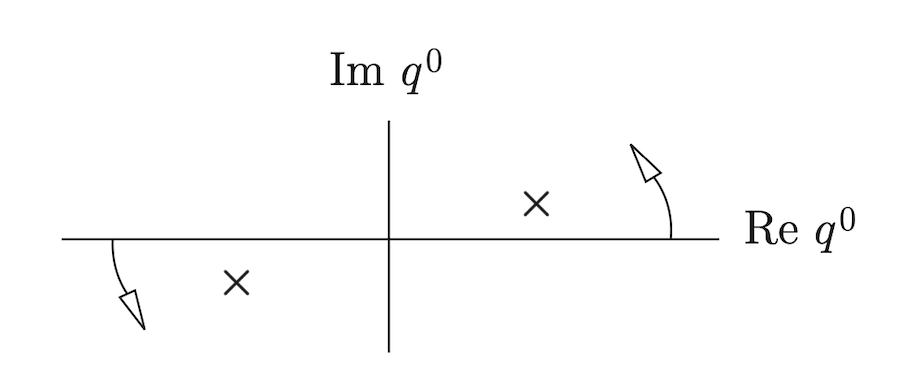Peskin:
by taking $T$ to $\infty$ in a slightly imaginary direction: $T → \infty(1 - i\epsilon)$
where $\epsilon >0$.
We can take $T → \infty(1 + i\epsilon)$, $\epsilon >0$ and this is still a slightly imaginary direction. And thus you can continue down the desired path. The pre-factor in front of all the $E_n >0$ on the dual space summation is $$e^{iE_nT(1+i\epsilon)} = e^{-E_n\epsilon T}e^{iE_nT}$$ which has the desired effect of cancelling the higher order terms as $T\rightarrow\infty$ faster than the $E_0$ term.
Further explanation:
When the Epsilon trick is introduced in Srednicki (Ch 6), he concludes
What all this means is that if we use $(1−i\epsilon)H$ instead of $H$, we can be cavalier about the boundary conditions on the endpoints of the path. Any reasonable boundary conditions will result in the ground state as both the initial and final state. Thus we have
$$\langle 0|0\rangle_{f,h} = \int \mathscr{D}p\mathscr{D}q\exp\Big[i\int_\infty^\infty dt\big(p\dot{q}-(1-i\epsilon)H+fq+hp\big)\Big]$$
Srednicki does this to $H$ rather that to $T$ but its equivalent, and for the same reason (i.e. getting rid of the additional states).
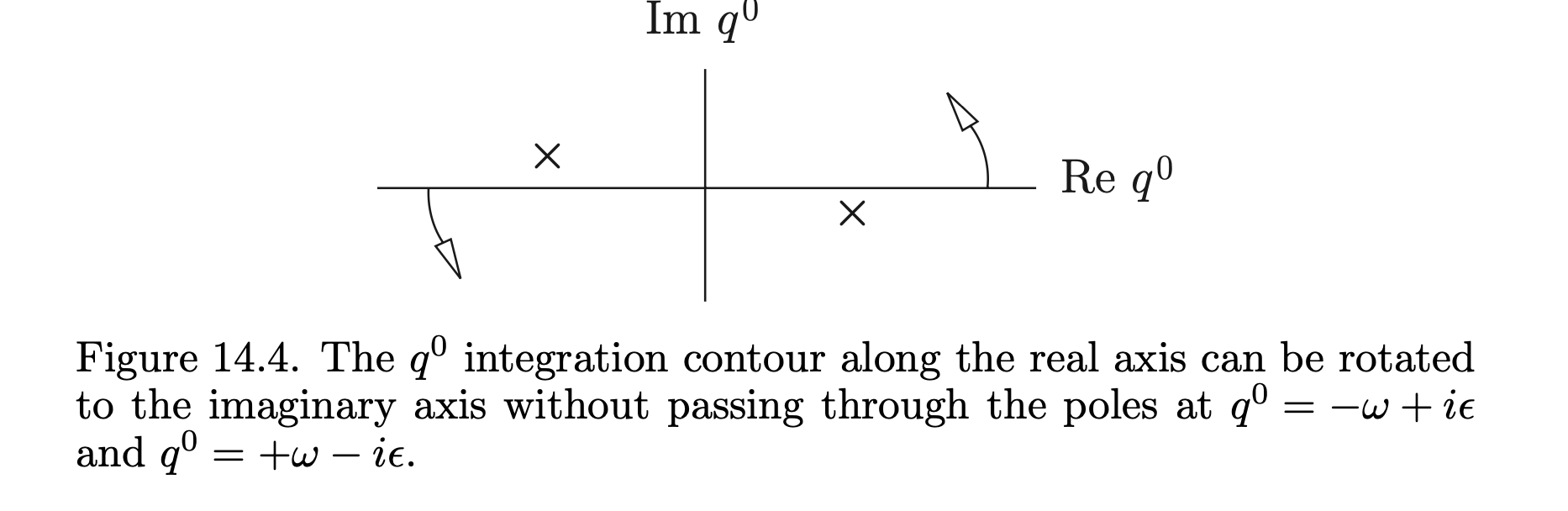
Down the line, we learn that taking $ H \rightarrow H(1-i\epsilon)$ is the same as taking $ m^2 \rightarrow m^2-i\epsilon$ which results in this Feynman propagator $$\Delta (x-x') = \int \frac{d^4k}{(2\pi)^4}\frac{e^{ik(x-x')}}{k^2+m^2-i\epsilon}$$ and so the loop correction integral in Chapter 14 looks like $$\int \frac{d^d q}{(2\pi)}\int_0^1 dx \big[q^2 +D-i\epsilon\big]^{-2}$$
and so the poles look like this:
However, if we take $ H \rightarrow H(1+i\epsilon)$ and so $ m^2 \rightarrow m^2+i\epsilon$ the poles will shift to
but we have to do the Wick rotation the opposite of shown in the picture (i.e clockwise). Related and Related.