The acceleration is constant since the system demonstrates a single
body behavior.
Being connected by an inextensible, massless string insures both masses move as one thus having the same acceleration. Whether or not that acceleration is constant depends on whether or not the external force pulling on them is constant.
Then if m2 is lesser than m1 it means that the force transmitted to m2
has been reduced because if it was the same then it would produce a
greater acceleration and vice versa. What causes this change in force?
WhyWhy isn't the original force transmitted to m2
TheIn order for the two masses to accelerate together due to the pulling force $F$ on $m_1$, the string exertspulling force on $m_2$ will alwaysmust be less than the external force $F$ applied to $m_1$, regardless of the magnitudemagnitudes of the two masses, for the following reasons:.
The force that the connecting string exerts on $m_1$ must be less than the applied force $F$ on $m_1$ in order for $m_1$ to accelerate per Newton's 2nd law.
Then since the force the connecting string applies to $m_2$ has to be equal and opposite to the force the connecting string exerts on $m_1$ per Newton's 3rd law, the force exerted by the connecting string to $m_2$ must also be less than $F$.
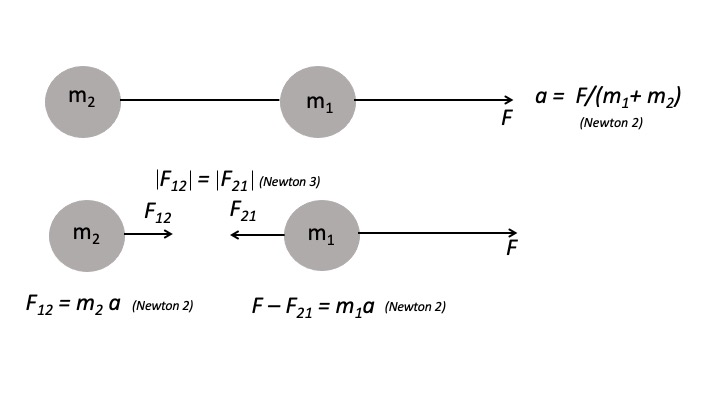
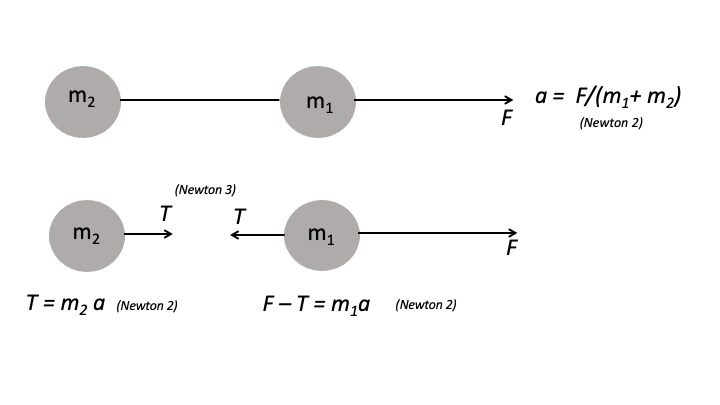
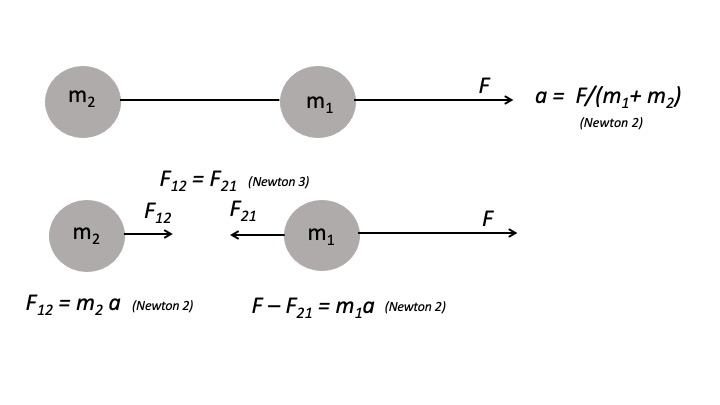
If you feel allTo see this may be hard to follow you are right. They say a picture is worth a thousand words, and in this case that picture is arefer to the free body diagramdiagrams (FBD) below, which assumes the only net external force on the twosystem of masses is $F$, as shown belowand that the strings are massless and inextensible. It assumes
From the FBD on $m_1$, for $m_1$ to accelerate to the right the tension force $T$ force in the string connecting the two masses must be less than $F$. From the FBD on $m_2$, the only net external force acting on the two body system$m_2$ is the tension force $T$. Therefore the force acting on $m_2$ must be less than $F$.
Hope this helps.