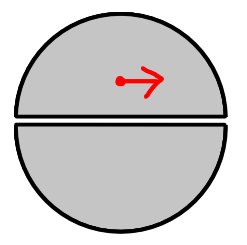
The velocities will be the same. Inspired by @NulliusinVerba's dumbell example, I came up with an intuitive proof:
Let's say we split the ball in two, and momentarily apply the same force to the center of mass of one of the halves:
The upper half gets twice the velocity (compared to the first ball from the question, because it has half its mass), and no angular velocity.
Then we immediately attach the two halves together.
The velocity of the resulting body is the same as that of the first ball, because of the coservationconservation of momentum.
And the angular velocity of the resulting body is obviously non-zero (which can be thought of in terms of conservation of angular momentum relative to the center of the ball).