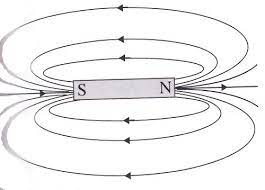
If a cylindrical magnet has a uniform magnetization $\vec{M}$ along its axis its magnetic field $\vec{B}$ looks approximately like this:
There seems to be a contradiction to me. Since there are no free currents anywhere, $\nabla \times H = \vec{0}$$\nabla \times \vec H = \vec{0}$ everywhere. But also, $\vec{B} = \mu_0(\vec{H} + \vec{M})$ everywhere. Then, because $\vec{M} = \vec{0}$ outside of the magnet, $\vec{H}$ has to be nonzero outside of the magnet, because clearly $\vec{B}$ is not zero there. But according to the Griffith's textbook, $\vec{H}$ is the magnetic field only due to free currents, or alternatively, the field that remains if one were to remove all magnetization. So if $\vec{H}$ is nonzero outside of the magnet, and it is not caused by free currents (because there are none), and it also is not caused by the magnetization, then what is H$\vec H$ and why does it exist in this case?