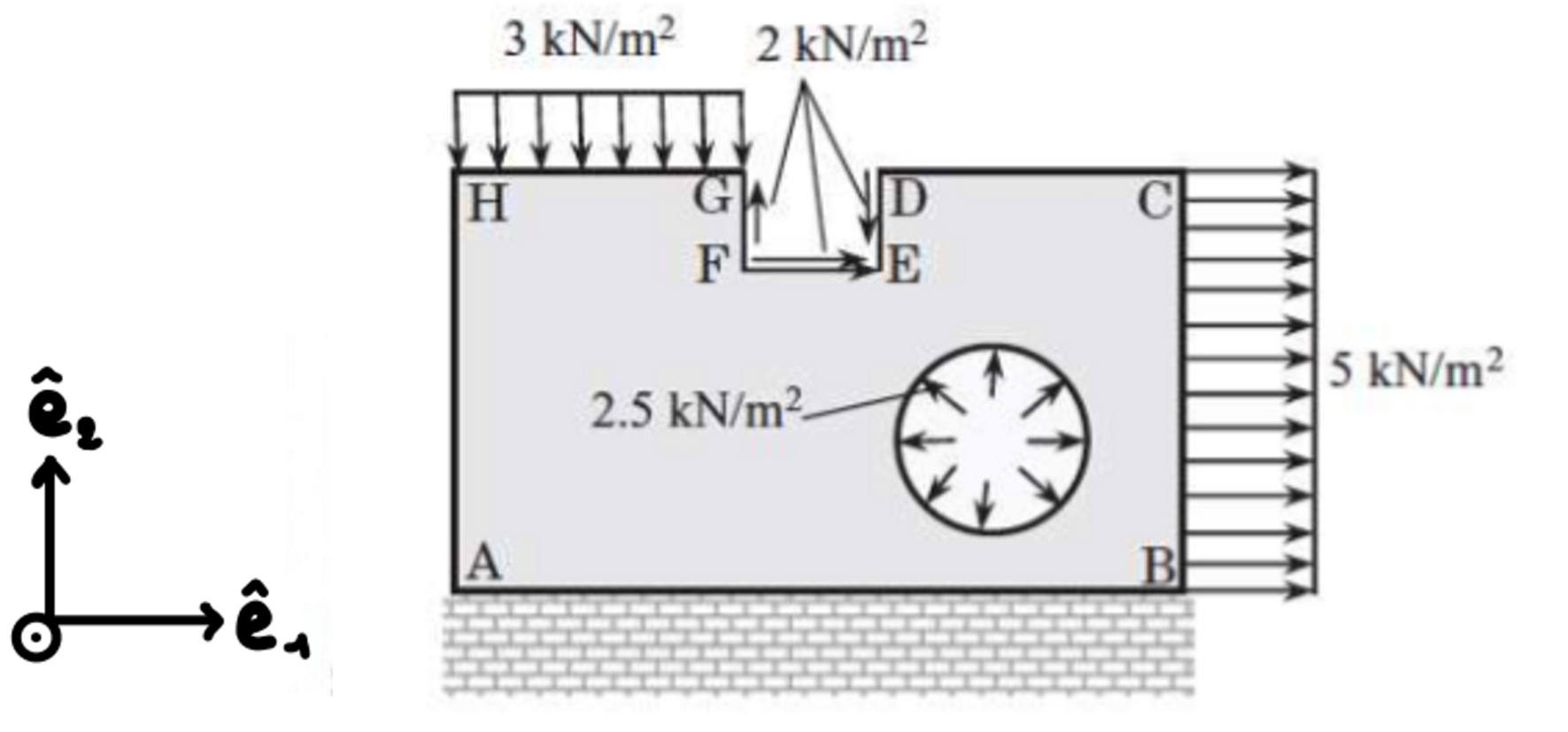
In the following problem, concerning the stress vectors applied on the surface of the circle, why is there a minus sign in $t(- ê_r) = t(− cos θ ê_1 − sin θ ê_2)$ $t(- ê_r) = t(− \cos θ\ ê_1 − \sin θ\ ê_2)$?
In this problem, forcesloads are applied as can be seen:

The solution from my course indicates the following answer:
Stress vector on the boundary of the circular cavity:
$t(- ê_r) = t(−cos θ ê_1 − sin θ ê_2) = 2.5(cos θ ê_1 + sin θ ê_2)$$t(- ê_r) = t(−\cos θ\ ê_1 − \sin θ\ ê_2) = 2.5(\cos θ\ ê_1 + \sin θ\ ê_2)$.
I just don't understand why there's a minus sign in the first two terms.