There are no negative probabilities.
There exists in the phase space formulation of QM “quasi-probability” distributions $W(x,p)$ that are somewhere negative, but the probabilities that occur as a result of integrating such distributions are always non-negative.
To be explicit, $$ \vert\psi(x)\vert^2=\int dp\, W(x,p) $$ (for instance) is obtained by integrating all momenta of the joint quasi-distribution $W(x,p)$, $\vert \psi(x)\vert^2$ agrees with the probability density computed from the Born rule, and is everywhere non-negative so that the probability of finding the system in some space interval is always non-negative.
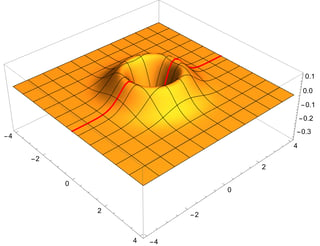
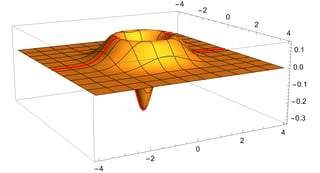
To illustrate this, look at the following two figures:
They show the same quasi-distribution function, which contains regions of negativity near the center, as illustrated in the right figure: the near the central "anti"-peak the quasidistribution is clearly negative. However, integrating this quasi distribution along the line $p$ at $x=-2$ (shown on the left figure by the red line) yields
$$ \int dp W(x=-2,p)=\vert\psi(x=-2)\vert^2 > 0 $$ so even if there are regions of negativity the probability of finding the system near $x=-2$, which is $\vert\psi(x=-2)\vert^2\,dx$, is of course positive. For completeness here $\psi(x)$ is in fact $\psi_1(x)$, the wavefunction of the $n=1$ harmonic oscillator state.
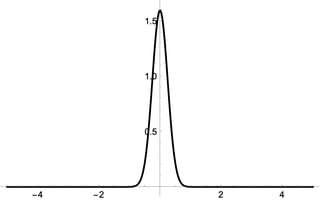
To make absolutely explicit the difference between a probability density and a probability, consider the following normalized Gaussian wavefunction:
$$ \psi(\sigma)=\frac{e^{-x^2/(2\sigma)}}{(\pi \sigma)^{1/4}} \tag{1} $$ for $\sigma=1/8$. $\psi(1/8)$ is a perfectly legitimate wavefunction for a particle in a harmonic oscillator. The resulting probability density $\vert \psi(1/8)\vert^2$ is $>1$ near the origin:
but of course this is not a problem. Since $\int_{-\infty}^{\infty} dx \vert \psi(1/8)\vert^2=1$ and $\vert \psi(1/8)\vert^2 \ge 0$ everywhere, it follows that, for any subinterval $[a,b]$: $$ \int_a^b dx \vert \psi(1/8)\vert^2 \le 1 $$ even if $\vert \psi(1/8)\vert^2$ can be greater than one somewhere. Nobody in their right mind would suggest that, because the probability density $\vert \psi(1/8)\vert^2$ is greater than one1 somewhere, probabilities greater than one1 are possible possible.
It's the same argument for negative regions of Wigner functions, which are (quasi)probability densities, with the distinction that these (quasi)probability densities can be negative.