This is no rocket science...
You see a frictionless railroad wagon passing by at speed $V$. A passenger on the wagon throws a ball at speed $u$ (relative to the train) in the opposite direction of travel. Will the speed of the wagon increase when $u \le V$?
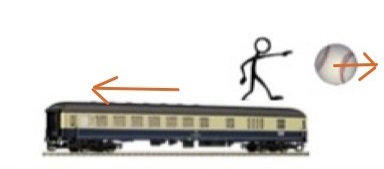
To arrive at an answer, all we need is momentum conservation. Let's call the mass of the train plus passenger $M$, and the mass of the ball $m$. Before throwing the ball, total momentum is $(M +m)\ V$. After throwing the ball, the total momentum equals the momentum $m(V-u)$ of the ball, plus the momentum $M(V+v)$ of the wagon with passenger (here, $v$ donates the unknown speed increase). Momentum conservation leads to the condition
$$M(V+v) \ + \ m(V-u) \ = \ (M + m)\ V$$
This can be re-arranged into
$$M\ v \ = \ m \ u$$
The speed $V$ has dropped out of this equation, and we have arrived at the momentum conservation condition as observed from the reference frame moving with the initial velocity of the train.
So the speed $V$ is irrelevant, and the value of ratio $u/V$ doesn't matter: as long as $u$ is nonzero, the wagon will increase its speed.
Change $M\ v \ = \ m \ u$$\ M\ v \ = \ m \ u\ $ into $M\ dv \ = \ -dM \ u$$\ M\ dv \ = \ -dM \ u\ $ and we refer to it as rocket science.. Yet, it's nothing more than Newton's law of momentum conservation.
