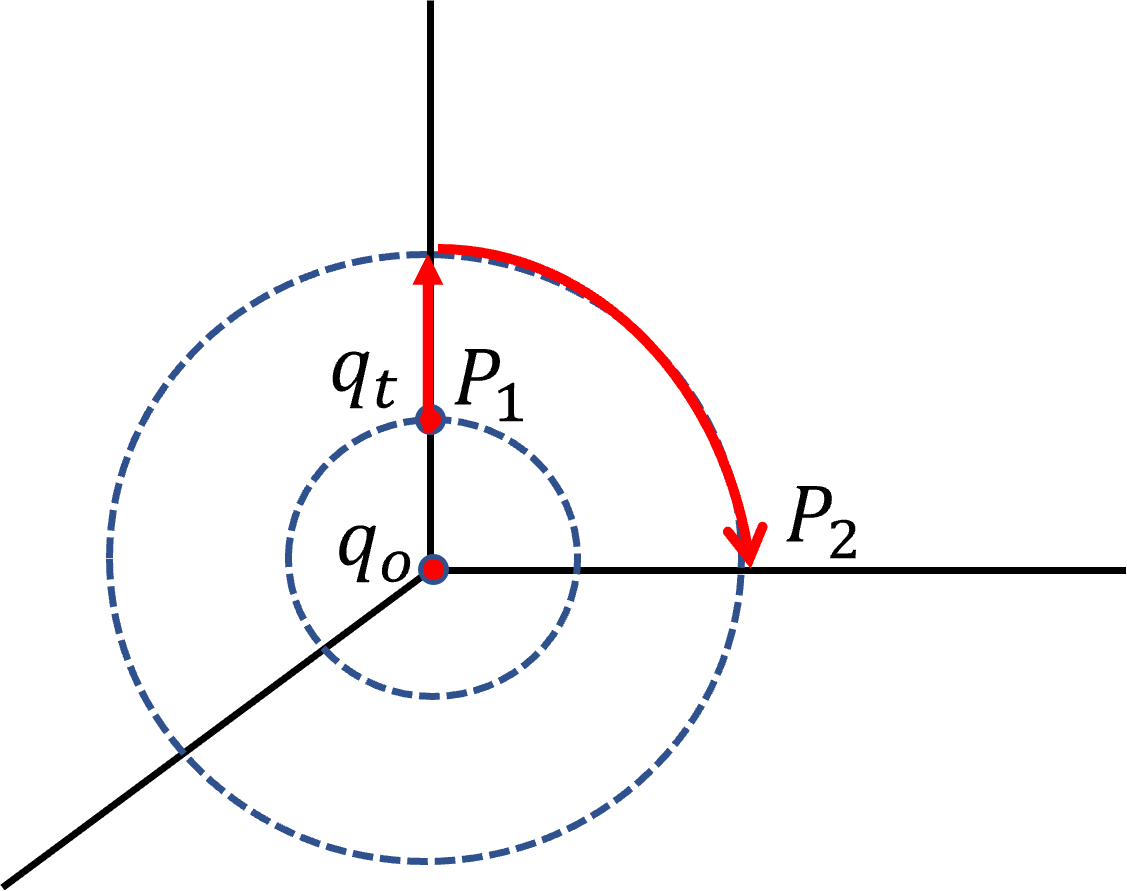
1. Calculate the work that charge $q_O$ do to $q_t$ when $q_t$ moves from $P_1$ to $P_2$ in the first picture, and here is its formula
$W=\int^{P_1}_{P_2}\vec u_R \frac{q_oq_t}{4\pi\epsilon_{0}R^2}\cdot[\vec u_RdR+\vec u_\theta Rd\theta]$$$W=\int^{P_1}_{P_2}\vec u_R \frac{q_oq_t}{4\pi\epsilon_{0}R^2}\cdot[\vec u_RdR+\vec u_\theta Rd\theta]$$
I want to ask why can we just write $\cdot [\vec u_RdR+\vec u_\theta Rd\theta] $ to describe this irregular path ?

In the second picture,we have to calculate the work that charge $q_O$ do to $q_t$ when $q_t$ moves from $P_1$ to $P_2$ along the red line path, the radius of inner circle and outer circle is $r_1$ and $r_2$ and here is its formula
$W=W_1+W_2$$$W=W_1+W_2$$
$W_1=\int^{r_2}_{r_1}\vec u_R \frac{q_oq_t}{4\pi\epsilon_{0}R^2} \cdot \vec u_R dR$$$W_1=\int^{r_2}_{r_1}\vec u_R \frac{q_oq_t}{4\pi\epsilon_{0}R^2} \cdot \vec u_R dR$$
$W_2=\int^{\frac{\theta}{2}}_{\theta=0}\vec u_R \frac{q_oq_t}{4\pi\epsilon_{0}R^2} \cdot \vec u_\theta Rd\theta$$$W_2=\int^{\frac{\theta}{2}}_{\theta=0}\vec u_R \frac{q_oq_t}{4\pi\epsilon_{0}R^2} \cdot \vec u_\theta Rd\theta$$
in the second picture,i want ask why should write
1.$\vec u_R \frac{q_oq_t}{4\pi\epsilon_{0}R^2}$, not $\vec u_\theta \frac{q_oq_t}{4\pi\epsilon_{0}R^2}$,why must the direction be R,not $\theta$ ?
2.why should we $\cdot \vec u_\theta Rd\theta$ in the $W_2$, instead of $\cdot \vec u_R Rd\theta$