Return to Answer

Here $V_0$ is the potential to which the conducting hemisphere has been charged, and $a$ is the hemisphere's radius. The angle $\theta$ is the usual polar angle from the $z$-axis in spherical polar coordinates, when the hemisphere is the $z\ge 0$ half of the sphere; in other words, $0\le\theta\le\pi/2$ where crown of the bowl is at $\theta = 0$ and the rim at $\theta = \pi/2$.

The fact that $\sigma_\text{out}-\sigma_{in}$ is a constant is interesting, and I don't know of a physical explanation for it. That constant, $V_0/4\pi a$, is the same difference as for a spherical shell.

A check on my calculation is that the capacitance I found agrees with equation (8.17) in Ref. 1. Ref. 2 mentions the constant difference in the inside and outside charge densities. So I'm confident that my equations are correct.

Here $V_0$ is the potential to which the conducting hemisphere has been charged, and $a$ is the hemisphere's radius. The angle $\theta$ is the usual polar angle from the $z$-axis in spherical polar coordinates, when the hemisphere is the $z\ge 0$ half of the sphere; in other words, $0\le\theta\le\pi/2$ where the crown of the bowl is at $\theta = 0$ and the rim at $\theta = \pi/2$.

The fact that $\sigma_\text{out}-\sigma_\text{in}$ is a constant is interesting, and I don't know of a physical explanation for it. That constant, $V_0/4\pi a$, is the same difference as for a spherical shell.

A check on my calculation is that the capacitance I found agrees with equation (8.17) in Ref. 1. Ref. 2 mentions the constant difference in the inside and outside charge densities. So I'm confident that my equations are correct.

If you want to calculate the charge density from the potential yourself, a computer algebra system will come in handy.

The infinite charge density at the rim is due to the assumption that the bowl is infinitely thin.

The infinite charge density at the rim is due to the assumption that the bowl is infinitely thin and thus has an infinitely sharp edge.

The surface charge density will not be uniform.

The problem of the electrostatics of a conducting hemispherical shell is a special case of a more general problem that appears in the 1949 paper "Potential problems and Capacitance for a Conductor Bounded by Two Intersecting Spheres" by Chester Snow, published in the Journal of Research of the National Bureau of Standards, Volume 43.

See Figure 1(f) for how the general problem reduces to a hemispherical shell when $\omega = 2\pi$ and $\theta = \pi/2$.

Snow obtains an exact expression for the potential in toroidal coordinates, from which one could calculate the field and the surface charge density.

The potential of the hemisphere is complicated, but its capacitance turns out to have a particularly simple form,

$$C = a\left(\frac12+\frac1\pi\right),$$

where $a$ is the radius of the hemisphere. (Note: This formula is in Gaussian units where capacitance has the dimensions of length, emphasizing its essential geometric nature.)

Snow's paper is a lengthy tour de force of applied mathematics and is not easy reading.

The surface charge density of a charged conducting hemispherical bowl is not uniform. In fact, it is highly non-uniform and looks like this:

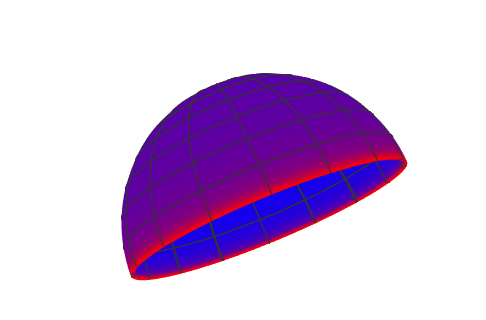
Red coloring means high charge density and blue coloring means low.

More than 10% of the charge is within 1 degree of the rim of the conducting hemispherical bowl, more than 34% within 10 degrees, and more than 50% within 20 degrees.

There is a surface charge density $\sigma_\text{out}$ on the outside, and a different (and lower) charge density $\sigma_\text{in}$ on the inside; the total charge density $\sigma$ is the sum of these. These three charge densities are given by reasonably simple expressions involving only elementary functions:

$$\begin{align} \sigma_\text{out} &= \frac{1}{4\pi^2}\left(\pi + \sqrt{\sec\theta} - \arctan{\sqrt{\sec\theta}}\right)\frac{V_0}{a}; \\ \sigma_\text{in} &= \frac{1}{4\pi^2}\left(\sqrt{\sec\theta} - \arctan{\sqrt{\sec\theta}}\right)\frac{V_0}{a}; \\ \sigma &= \frac{1}{4\pi^2}\left(\pi + 2\sqrt{\sec\theta} - 2\arctan{\sqrt{\sec\theta}}\right)\frac{V_0}{a}. \\ \end{align}$$

Here $V_0$ is the potential to which the conducting hemisphere has been charged, and $a$ is the hemisphere's radius. The angle $\theta$ is the usual polar angle from the $z$-axis in spherical polar coordinates, when the hemisphere is the $z\ge 0$ half of the sphere; in other words, $0\le\theta\le\pi/2$ where crown of the bowl is at $\theta = 0$ and the rim at $\theta = \pi/2$.

The fact that $\sigma_\text{out}-\sigma_{in}$ is a constant is interesting, and I don't know of a physical explanation for it. That constant, $V_0/4\pi a$, is the same difference as for a spherical shell.

Each of these charge densities increases monotonically from a non-zero constant value at the crown to infinity at the rim, as shown by the following graph:

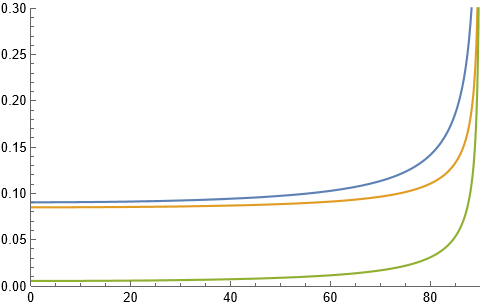
Here the yellow line is the outside charge density, the green line is the inside density, and the blue line is the total density. The horizontal axis is in degrees, and the vertical axis is the surface charge density in units of $V_0/a$.

The infinite charge density at the rim is due to the assumption that the bowl is infinitely thin.

Despite the fact that the charge densities become infinite at the rim, the integrated total charge turns out to be finite, as one would expect:

$$\begin{align} \\ Q_\text{out} &= \left(\frac{1}{2}+\frac{1}{2\pi}\right)V_0a; \\ Q_\text{in} &= \left(\frac{1}{2\pi}\right)V_0a; \\ Q &= \left(\frac{1}{2}+\frac{1}{\pi}\right)V_0a. \\ \end{align}$$

The capacitance of a conducting hemisphere is thus

$$C = \frac{Q}{V_0} = \left(\frac{1}{2}+\frac{1}{\pi}\right)a.$$

A full sphere has capacitance $a$, so the capacitance of a hemisphere is about 64% greater than half the capacitance of a full sphere.

I calculated the above formulas for charge density and total charge myself, starting with the exact electrostatic potential of the hemisphere, which is derived in Ref. 1. The gradient of the potential gives the field, and the surface charge density is proportional to the (normal) field at the surface:

$$\sigma = \frac{1}{4\pi}E = -\hat n \cdot \vec\nabla \varphi$$

You didn't ask about the potential, so I won't state the formula for it. It's much more instructive to look at a plot of it, so that you can understand why the charge density is non-uniform and why it is less on the inside.

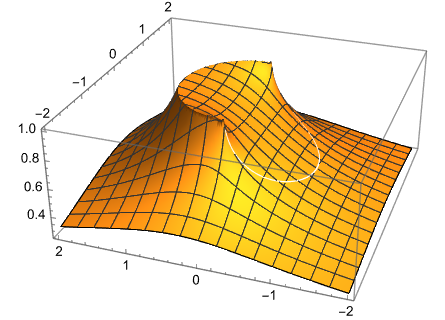
This plot show the potential on a plane containing the axis of the hemisphere. Due to the cylindrical symmetry of the problem, it's the same on any such plane. The vertical axis is the value of the potential in units of $V_0$. The horizontal axes are, say, $x$ and $z$, and are in units of $a$.

If you are interested in knowing the formula for the potential, it is given by equations (6.10) and (9.6) in Ref. 1. Like the charge density, it does not involve infinite series, integrals, or special functions, but only elementary functions.

A complication is that it is expressed in toroidal coordinates $\alpha$ and $\beta$, whose relationship to cylindrical coordinates is given by equations (1.5) and (1.6). The reason is that the potential of the hemisphere is derived in Ref. 1 by solving Laplace's equation in toroidal coordinates.

An additional complication of Ref. 1 is that it actually considers a much more general case involving conductors that consist of two intersecting spherical surfaces. Bowls are the case where the parameter $\omega$ is equal to $2\pi$, and the hemispherical bowl is the case where the parameter $\theta$ (which in Ref. 1 is not the polar angle but the opening angle of the bowl) is $\pi/2$.

Ref. 1 is from 1949, but, according to Ref. 2, Lord Kelvin found the charge density of a conducting spherical bowl in 1869. His approach started with a charged disk and used a technique called inversion.

A check on my calculation is that the capacitance I found agrees with equation (8.17) in Ref. 1. Ref. 2 mentions the constant difference in the inside and outside charge densities. So I'm confident that my equations are correct.

NOTE: All formulas in this answer in Gaussian units where the Coulomb constant has the dimensionless value $1$ rather than being equal to $1/4\pi\epsilon_0$.

References:

Ref. 1: Chester Snow, "Potential problems and Capacitance for a Conductor Bounded by Two Intersecting Spheres", Journal of Research of the National Bureau of Standards, Volume 43, 1949.

Ref. 2: Phil Lucht, "The Charged Bowl in Toroidal Coordinates", ResearchGate.net, 2016.

Ref. 3: S. Loh, "Calculation of the electric field and the capacitance of a charged spherical bowl by means of toroidal co-ordinates", Proceedings of the Institution of Electrical Engineers, Vol. 17, Issue 3, p. 641, 1970.