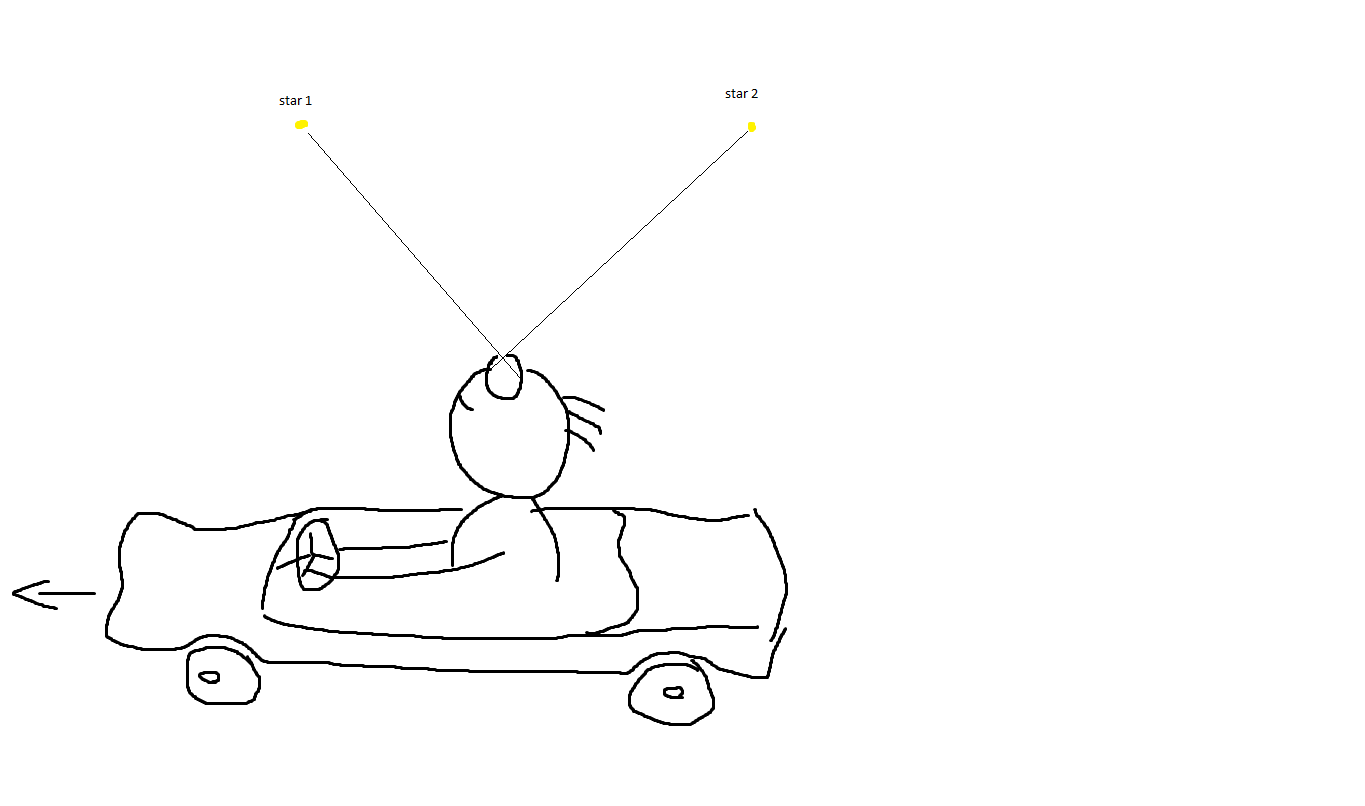
Consider the following situation:
You are moving with high speed on a road on earth and are looking at the night sky, in particular at two stars. From an outside perspective (at
In the siderest frame of the road)earth, you and your eye are therefore length contracted.
For simplicity, let's say the outside perspective can look in your eye and see the two dots on your retina that correspond to those two stars. This This implies that, from the outside perspective, theproper distance between those twothe images of the stars observed by youon your retina is greater than the distance observed frombetween the outside perspectiveimages on the retina of an observer at rest w.r.t. the earth (in my calculations the angles also change, but this does not influence the distance, because both angles change).
But because the position of the dots on your retina are independent of the frame of reference (or more specifically, the cells that are excited by starlight) are independent of the frame of reference, you also observe a distance between the two stars that is greater than from the outside perspectiveother observer. Now that is a problem, because special relativity says you should observe a smaller distance (the sky is length contracted).
So the question is did I make a mistake? Is this actually what happens?