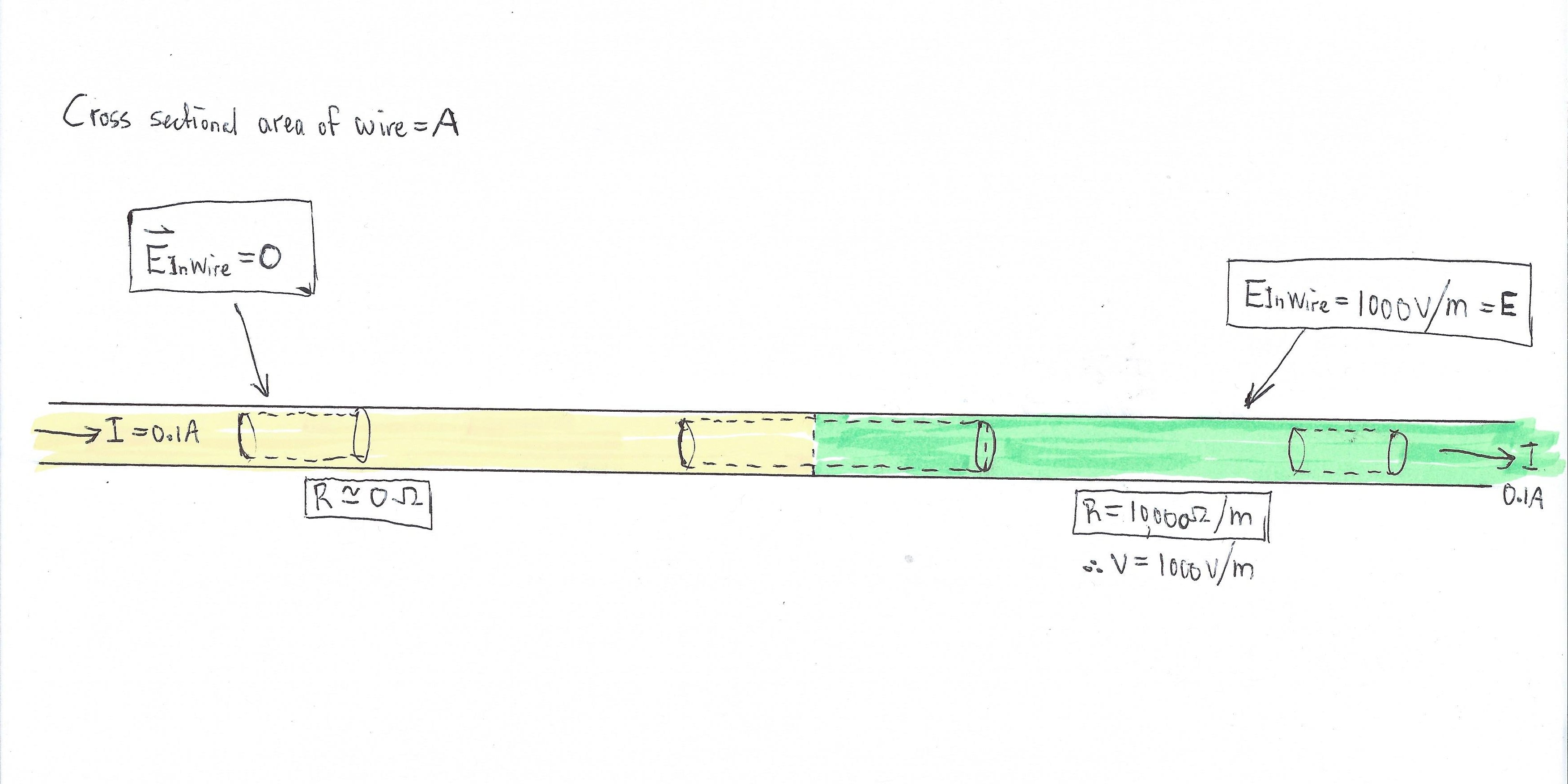
Consider the very long, current-carrying wire in the picture below. On the left-hand side, in yellow, the wire has a very low resistance, that we will consider to be zero. But on the right hand-hand side in green, the resistance is much higher, for example 10,000 ohms per meter. There is a current of 0.1 A, so in this example the voltage across the resistive part of the wire is 1000 V per meter of wire. The $E$-field inside the resistive part of the wire is therefore 1000 V/m.
No let'sLet's do some textbook application of Gauss Law, which states that the surface integral of the electric field, over any closed surface, is equal to the enclosed charge divided by the permittivity constant. On the left, inside the zero-resistance wire, the electric field in the wire is of course zero, because the resistance of the wire is zero, so there is no voltage drop along the wire. As sketched, we take a cylindrical Gaussian surface, just inside the surface of the wire. The electric field is zero everywhere on the Gaussian surface, so the surface integral is zero, and thus there is no enclosed charge. Yes. Everything ship-shape here, noting the well-known fact that there is zero net charge inside of a conductor, and that any excess charge resides on the surface, just outside of our Gaussian surface.
Now consider a similar cylindrical Gaussian surface on the right, inside the green resistive wire. The electric field inside the wire here has the value $E$, in this case 1000 V/m. So the product of the electric field and the area is (AE) on each end of the cylinder. However, the electric flux is entering on one end, and leaving at the other end, so the sum of the flux is still zero, telling us there is zero enclosed charge. Gauss wins again.
Now consider the Gaussian cylindrical surface in the center. On the LH end of the cylinder, the flux is zero, because the electric field here is zero. But on the RH end the flux is (EA), the non-zero electric field strength of 1000 V/m, multiplied by the cross sectional-sectional area of the wire. Hmmm. This tells us that there must be charge enclosed inside the wire, inside of the cylindrical Gaussian surface. But it is well knowknown and accepted that there is no charge inside of a conductor, and so we have an apparent paradox, a violation of the Gauss Law.
I'm pretty sure that I know what is happening, but it is such a cute problem that I would not wish to deprive others the opportunity to think about it.