At this point in Leonard Susskind's eighth lecture on general relativity, he begins a discussion about finding the metric of a black hole formed by an incoming, spherically symmetric shell of light. At this point, after explaining that flat spacetime is correct for the interior of the shell, while the Schwarzschild spacetime is correct for the exterior, he states that splicing the two correct regions of the diagrams together yields the answer.
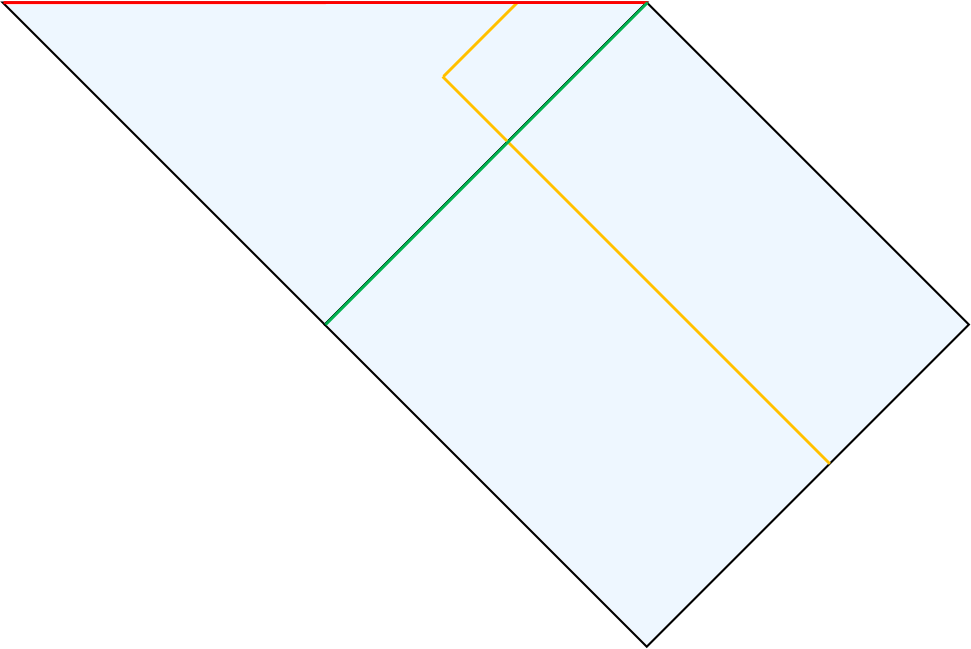
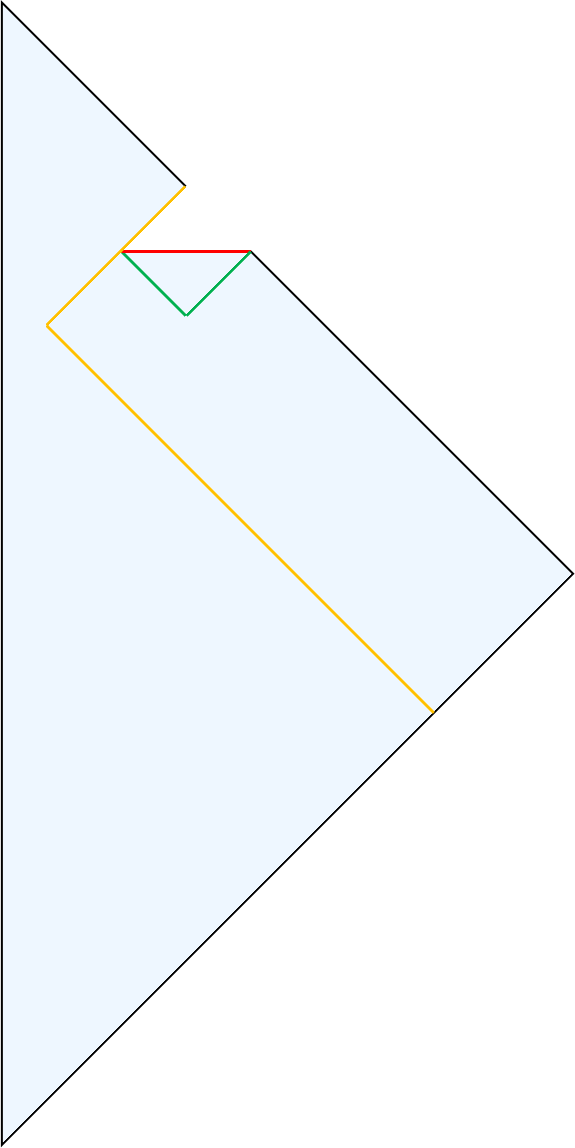
Inspired by the discussion that follows, consider building a spherical mirror which can reflect the light back, with a radius smaller than the Schwarzschild radius. The two diagrams for the flat and Schwarzschild spacetimes would look like this
and this
respectively (the yellow lines are the paths of the light, and the green line is the event horizon).
Following the same principle of splicing diagrams, is it legitimate to conclude that this is the correct overall spacetime?